filmov
tv
Solving f(x-f(y))=1-x-y

Показать описание
If you need to post a picture of your solution or idea:
#ChallengingMathProblems #FunctionalEquations #Functions
via @YouTube @Apple @Desmos @NotabilityApp
@googledocs @canva
PLAYLISTS 🎵 :
#ChallengingMathProblems #FunctionalEquations #Functions
via @YouTube @Apple @Desmos @NotabilityApp
@googledocs @canva
PLAYLISTS 🎵 :
Solving f(x-f(y))=1-x-y
Solving the Functional Equation f(f(x)+y)=x/(1+xy)
Solving f(x)+f(y)=f[(x+y)/(1-xy)]
Solving the Functional Equation f(x+y)=f(x)f(y)
Solving the Functional Equation f(x-y)=f(x)f(y)
Solving the Functional Equation f(xy+1)=xf(y)-f(x)+6
if f(x)=log(1+x/1-x) then show that f(x)+f(y)=f(x + y/1+xy)
Solving the Functional Equation f(x - y) = f(x) - f(y)
Methods of Solving Differential Equation | Homogeneous Method | Math | JEE 2025 Preparation
Solving f(xy)=f(x)-f(1/y)
A Functional Equation f(x+y)=f(x)+f(y)-xy
Solving f(xy)=xf(y) in Two Ways
Solving an equation for y and x
Solving the functional equation f[x+f(y)]=x+y+1
Learn how to evaluate for a function
Solving f(x/y)=f(x)/f(y), A Nice Functional Equation
Solving the Functional Equation f(x+y)=xf(y)
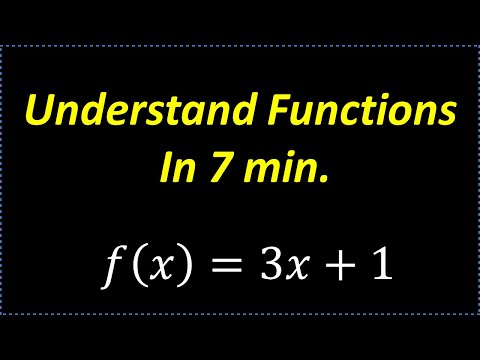
Learn Functions – Understand In 7 Minutes
Solving f(x^y)=yf(x) | A Functional Equation
Graph transformations y=1/f(x)
f(x+y) = f(x)f(y)
Evaluating f(xy)=f(x)+f(y)-1
5 simple unsolvable equations
How REAL Men Integrate Functions
Комментарии
 0:06:56
0:06:56
 0:05:51
0:05:51
![Solving f(x)+f(y)=f[(x+y)/(1-xy)]](https://i.ytimg.com/vi/Xyl1M40zmbs/hqdefault.jpg) 0:14:09
0:14:09
 0:10:18
0:10:18
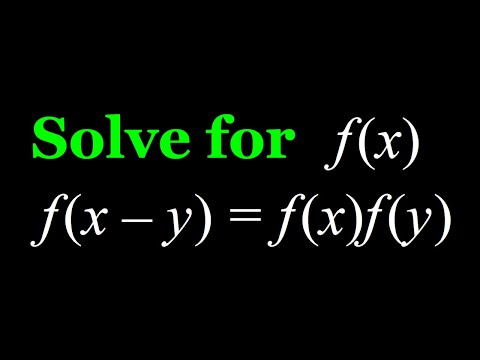 0:05:41
0:05:41
 0:05:51
0:05:51
 0:07:48
0:07:48
 0:06:21
0:06:21
 0:45:31
0:45:31
 0:06:00
0:06:00
 0:07:54
0:07:54
 0:03:27
0:03:27
 0:03:47
0:03:47
 0:06:01
0:06:01
 0:02:15
0:02:15
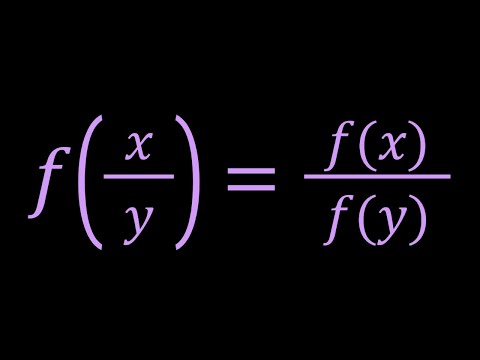 0:07:10
0:07:10
 0:06:11
0:06:11
 0:09:43
0:09:43
 0:06:10
0:06:10
 0:07:55
0:07:55
 0:07:14
0:07:14
 0:04:41
0:04:41
 0:00:50
0:00:50
 0:00:35
0:00:35