filmov
tv
Solving a Suggested Functional Equation
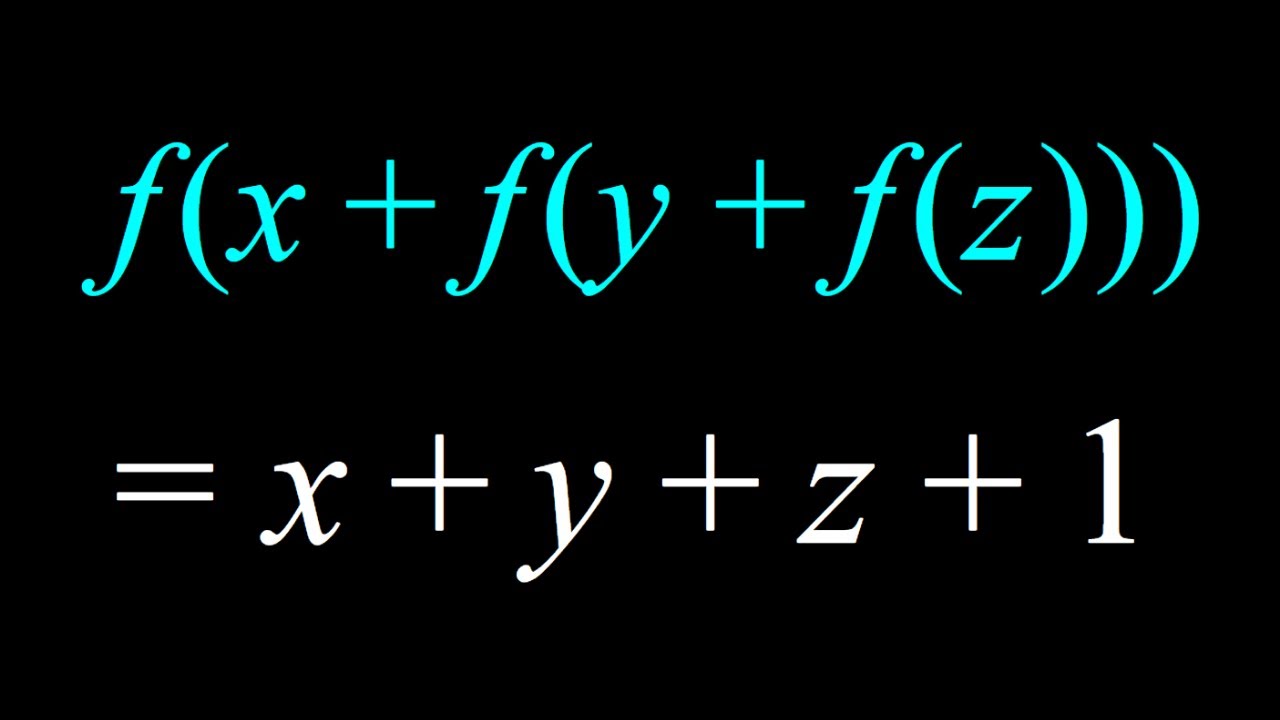
Показать описание
If you need to post a picture of your solution or idea:
#ChallengingMathProblems #FunctionalEquations
EXPLORE 😎:
PLAYLISTS 🎵 :
#ChallengingMathProblems #FunctionalEquations
EXPLORE 😎:
PLAYLISTS 🎵 :
Solving a Suggested Functional Equation
A Quick and Easy Functional Equation
Solving a Quick and Easy Functional Equation
A Nice and Easy Functional Equation
Methods of Functional Equations
Solving a Functional Equation
Solving a Homemade Functional Equation in Two Ways
A Functional Equation from Samara Math Olympiads
Solving a Quick and Easy Functional Equation
the greatest functional equation of all time.
A Functional Equation with Golden Flavor
A Quick and Easy Functional Equation from Slovenia
A Cool Functional Equation
Solving A Nice Functional Equation
Solving a Quick and Easy Functional Equation
Solving A Fun Functional Equation
Functional Equation
Solving a Functional Equation | f(x)+f(x-1)=x^2
Solving A Functional Equation
a functional equation /functional equation
a notorious functional equation.
A Functional Equation from 2019 IMO
A Functional Equation from British Math Olympiads 2009
Let's Solve A Functional Equation | 2nd Method?
Комментарии
 0:09:00
0:09:00
 0:06:49
0:06:49
 0:05:47
0:05:47
 0:05:07
0:05:07
 0:07:40
0:07:40
 0:10:36
0:10:36
 0:10:26
0:10:26
 0:08:47
0:08:47
 0:08:18
0:08:18
 0:07:48
0:07:48
 0:07:21
0:07:21
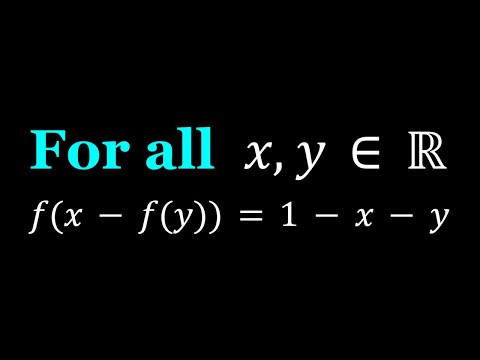 0:06:25
0:06:25
 0:12:43
0:12:43
 0:09:50
0:09:50
 0:06:19
0:06:19
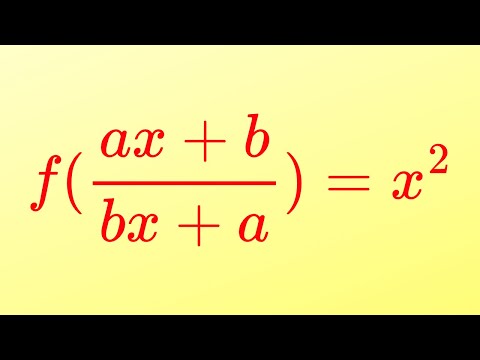 0:09:14
0:09:14
 0:14:15
0:14:15
 0:08:59
0:08:59
 0:00:29
0:00:29
 0:04:42
0:04:42
 0:19:30
0:19:30
 0:09:44
0:09:44
 0:08:33
0:08:33
 0:08:53
0:08:53