filmov
tv
A Quick and Easy Functional Equation

Показать описание
If you need to post a picture of your solution or idea:
#ChallengingMathProblems #FunctionalEquations
EXPLORE 😎:
PLAYLISTS 🎵 :
#ChallengingMathProblems #FunctionalEquations
EXPLORE 😎:
PLAYLISTS 🎵 :
A Quick and Easy Functional Equation
Solving a Quick and Easy Functional Equation
Solving a Quick and Easy Functional Equation
Solving a Quick and Easy Functional Equation
A Quick and Easy Functional Equation from Slovenia
Solving a Quick and Easy Homemade Functional Equation
A Nice and Easy Functional Equation
Learn Functional Groups FAST (Organic Chemistry)
Dual Function Vacuum #PowerfulSuction #VacuumBlower #CarVacuum #PortableVacuum #short #fy #fyp
Functional Resume Defined I Indeed Career Quick Tips
Creating A Functional And Perfect Bedroom In A Two-Square-Meter Space #shorts
#maths | A Quick And Easy Functional Equation | #function #youtubevideos
VEVOR Multi-Functional Food Processor - Vegetable Cutter Commercial
The Most Functional Exercise You Can Do
Super functional strength exercise! Helps you to become stronger, healthier and overall fit! ❤️🔥...
Functional programming - A general introduction
Making a Fully Functional Jet Engine using Soda can | diy Jet Engine
Functional Requirements and Specifications: A Quick Tutorial
Comfortable, functional, simple – The Rolex Glidelock
Solving A Quick Functional Equation in Two Ways
What is functional programming | Easy way
how to make simple and functional tools #weldingtipsandtricks #tools #welding #weldingtools #diy
Pollos En Peligro || Chicken in Danger || Galline in pericolo #CeroMiedos #ZeroFears
An Easy Home Functional Training Workout for Beginners (Can't Do a Push up? No Problem!)
Комментарии
 0:06:49
0:06:49
 0:08:18
0:08:18
 0:05:47
0:05:47
 0:06:19
0:06:19
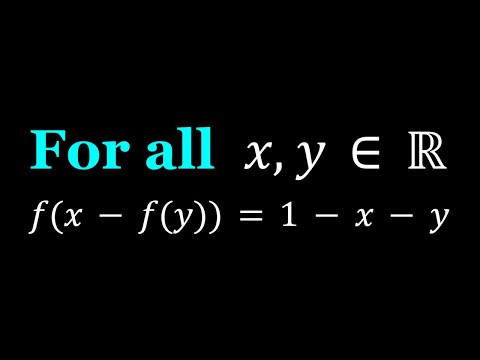 0:06:25
0:06:25
 0:05:21
0:05:21
 0:05:07
0:05:07
 0:03:51
0:03:51
 0:00:21
0:00:21
 0:00:18
0:00:18
 0:00:54
0:00:54
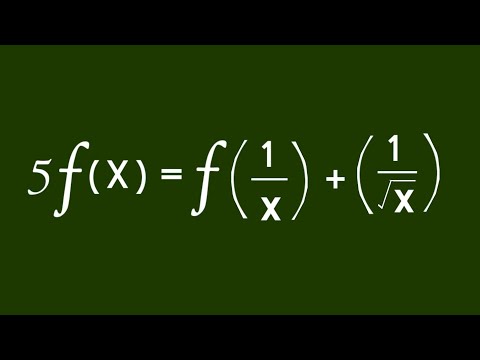 0:08:09
0:08:09
 0:01:08
0:01:08
 0:01:24
0:01:24
 0:00:26
0:00:26
 0:11:47
0:11:47
 0:16:27
0:16:27
 0:15:03
0:15:03
 0:00:11
0:00:11
 0:05:43
0:05:43
 0:09:18
0:09:18
 0:01:00
0:01:00
 0:00:09
0:00:09
 0:17:35
0:17:35