filmov
tv
Methods of Functional Equations

Показать описание
In this video, I showed how to solve functional equations using both substitution and form manipulation
Methods of Functional Equations
A Tale of Two Methods: Solving Functional Equations Like a Pro !
Slick Solutions to Functional Equations
Gaining Insights: Solving a Functional Equation
A Quick and Easy Functional Equation
Let's Solve A Functional Equation | 2nd Method?
Functional Equations #4 - The Substitution Strategy (Circle method)
Methods of Functional Equations || Math Question
Can you solve this Exponential equations?math Olympiad questions
Solving a Radical Functional Equation in Two Ways
Solving A Functional Equation | Substitution
Linear vs nonlinear function #equation #graph #table #slope
Functional Equation from JEE: Proven Methods for Finding x
Functional Equations (9-2) - Fixed point technique
A Functional Equation | f(f(x-3)+x)=x^2
Have You Solved a Functional Equation Before?
A Fun Functional Equation
A Nice Algebra Challenge | Functional Equation | Olympiad Prep!
Ultimate Functional Equation: Solving for f(x)
a functional equation/ an exciting functional equation
A Nice Algebra Challenge from Functional Equations | Olympiads
A Rational Functional Equation Solved in Two Ways
Solving a Quick and Easy Functional Equation
Solving a Homemade Functional Equation 1
Комментарии
 0:07:40
0:07:40
 0:10:57
0:10:57
 0:08:22
0:08:22
 0:10:22
0:10:22
 0:00:45
0:00:45
 0:08:53
0:08:53
 0:09:20
0:09:20
 0:08:10
0:08:10
 0:07:02
0:07:02
 0:09:16
0:09:16
 0:08:28
0:08:28
 0:00:05
0:00:05
 0:09:05
0:09:05
 0:10:05
0:10:05
 0:00:38
0:00:38
 0:00:40
0:00:40
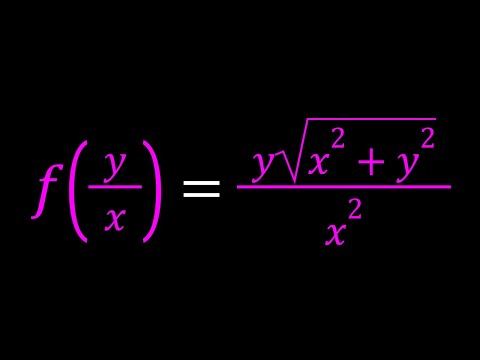 0:10:19
0:10:19
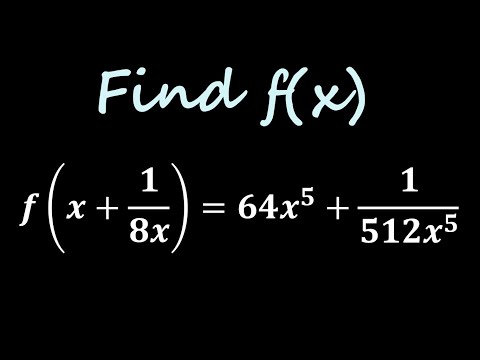 0:10:50
0:10:50
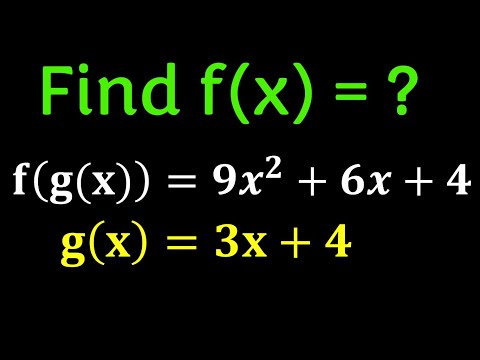 0:08:48
0:08:48
 0:05:30
0:05:30
 0:11:58
0:11:58
 0:08:51
0:08:51
 0:06:19
0:06:19
 0:08:55
0:08:55