filmov
tv
Let's Solve A Functional Equation | 2nd Method?
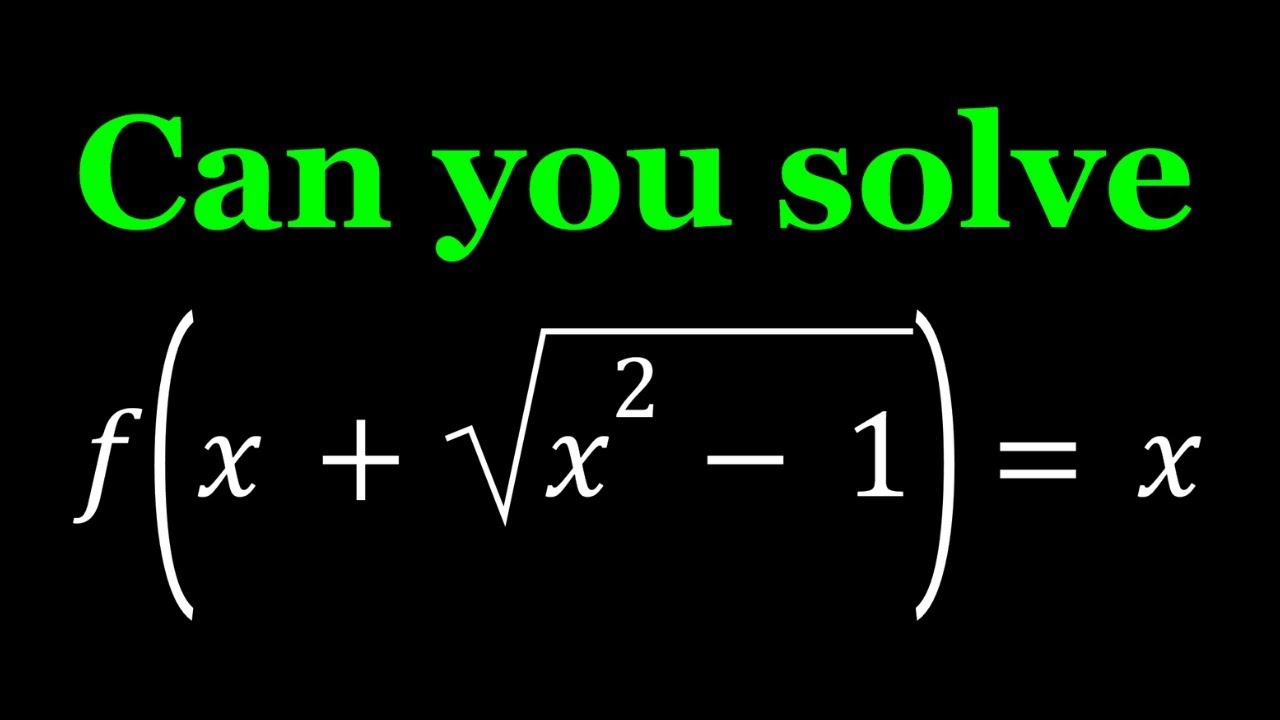
Показать описание
🤩 Hello everyone, I'm very excited to bring you a new channel (aplusbi)
Enjoy...and thank you for your support!!! 🧡🥰🎉🥳🧡
If you need to post a picture of your solution or idea:
#algebra #functionalequations #functions
via @YouTube @Apple @Desmos @NotabilityApp @googledocs @canva
PLAYLISTS 🎵 :
Enjoy...and thank you for your support!!! 🧡🥰🎉🥳🧡
If you need to post a picture of your solution or idea:
#algebra #functionalequations #functions
via @YouTube @Apple @Desmos @NotabilityApp @googledocs @canva
PLAYLISTS 🎵 :
A Super Quick and Easy Functional Equation
Solution 67: Substitution and Symmetry vs. a Functional Equation
Solving a Quick and Easy Functional Equation
Solving a Rational Functional Equation
Solving a Homemade Functional Equation 1
Solving a Quick and Easy Functional Equation
Solving A Nice Functional Equation
a functional equation
the greatest functional equation of all time.
A Curious Functional Equation | Math Olympiads
A Cool Functional Equation
Solving a Quick and Easy Functional Equation
A Functional Equation from Putnam and Beyond
Solving a Functional Equation
Solving a Radical Functional Equation in Two Ways
Solving a Functional Equation by Elimination
A Homemade Functional Equation
a FUNctional equation...
Functional Equation: How to solve? #math #maths
A Cool Functional Equation
A Quick Functional Equation | India National Mathematical Olympiad 2005 Problem 6
A fun functional equation!!
Solving an Interesting Functional Equation
a fun functional equation
Комментарии
 0:06:49
0:06:49
 0:12:16
0:12:16
 0:08:18
0:08:18
 0:06:53
0:06:53
 0:08:55
0:08:55
 0:06:19
0:06:19
 0:09:50
0:09:50
 0:16:24
0:16:24
 0:07:48
0:07:48
 0:08:29
0:08:29
 0:05:38
0:05:38
 0:05:47
0:05:47
 0:12:07
0:12:07
 0:10:36
0:10:36
 0:09:16
0:09:16
 0:09:08
0:09:08
 0:09:36
0:09:36
 0:16:40
0:16:40
 0:07:50
0:07:50
 0:12:43
0:12:43
 0:06:09
0:06:09
 0:11:13
0:11:13
 0:09:49
0:09:49
 0:09:20
0:09:20