Visual Group Theory, Lecture 2.2: Dihedral groups

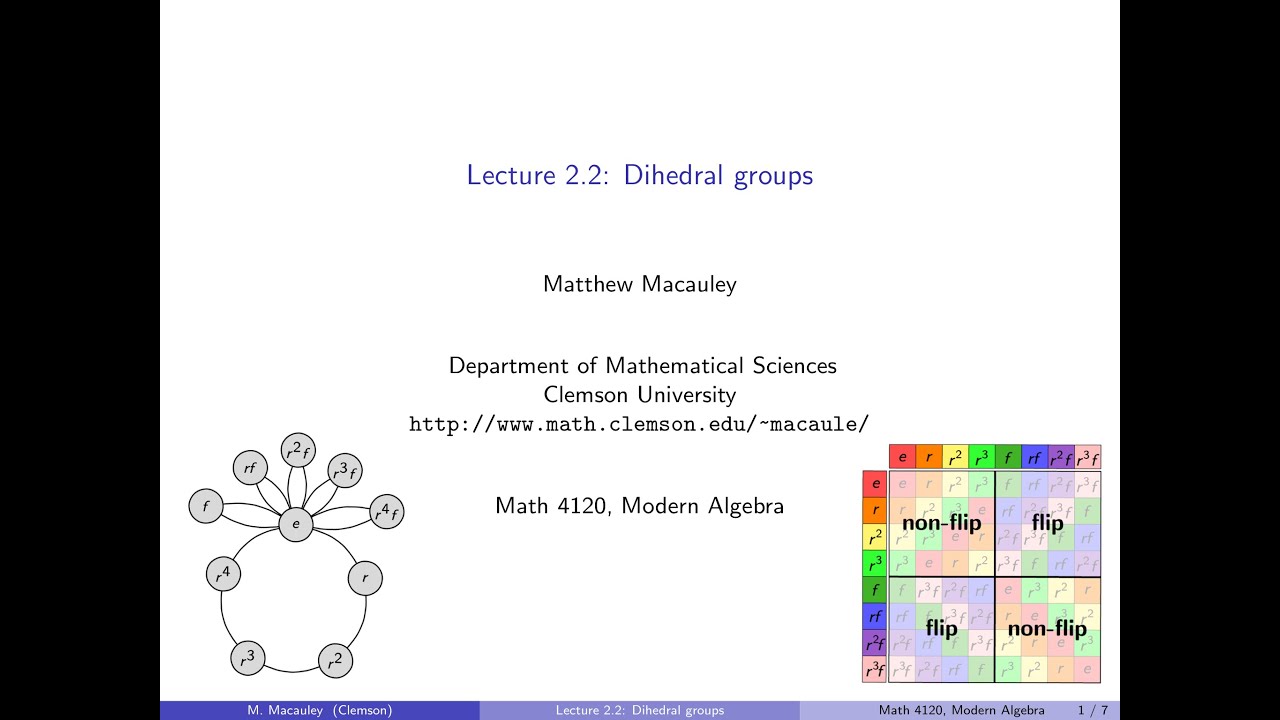
Cyclic groups describe the symmetry of objects that exhibit only rotational symmetry, like a pinwheel. Dihedral groups describe the symmetry of objects that exhibit rotational and reflective symmetry, like a regular n-gon. The corresponding dihedral group D_n has 2n elements: half are rotations and half are reflections. In this lecture, we introduce these groups and then study their properties using tools such as Cayley diagrams, group presentations, cycle graphs, and multiplication tables. We also get glimpse into two more advanced topics which we will study later: subgroups, and quotient groups.

[Jessica-nkwo]

I'm a visual learner and currently taking abstract algebra. I've really struggled with understand how to visualize groups so this series has been so good for me. Thank you!

[cristianchavez]

I can't recommend enough these lectures

[guiwenluo]

Elegant lectures regarding galois theory.

[xintongbian]

Finally it clicked on me what a quotient group is... btw bravo for this series, way better than socratica's clickbaits

[hanyanglee]

2:40 when you say Dn is more natural, your video signal doesn't agree.
3:45 when you say you don't think we have seen, your video signal doesn't agree again.

[joshmendez]

Anyone know where I would find solutions to the HW or perhaps other problems with solutions? I was thinking of looking at the textbook but wasn't sure as I'm quite new to higher maths

[SHASHAQUADADNIESHA]

Professor is there somewhere I can find you doing the solutions to the homework problems?

[sahhaf]

@9:35 the set derived seems not to be correct.. İf your rotations are st, ts, (st)^2, (ts)^2... up to n-1 th power you have 2n-1 rotations, but you should have n. Same with reflections. Hence, fot rotations you should only have (st)^k and similarly for reflections.

[wayneqwele]

Does anyone could recommend any problem sets for this video series?

[Agus-ofrh]

Guys, do you remember in which video he shows that the dihedral group has loops in opposite directions? (I mean, the inner loop in counter-clockwise and thou outer in clockwise)