Visual Group Theory, Lecture 6.5: Galois group actions and normal field extensions

Visual Group Theory, Lecture 6.5: Galois group actions and normal field extensions

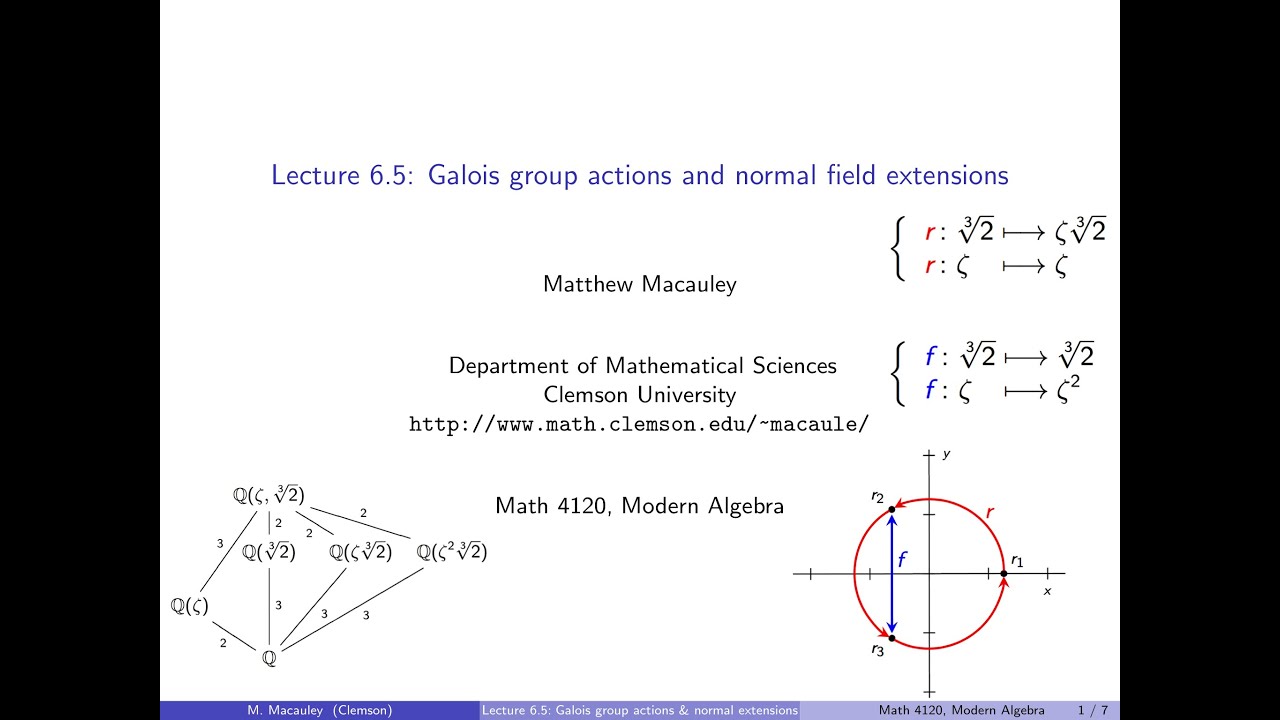
If f(x) has a root in an extension field F of Q, then any automorphism of F permutes the roots of f(x). This means that there is a group action of Gal(f(x)) on the roots of f(x), and this action has only one orbit iff f(x) is irreducible. An extension of Q is said to be "normal" if it is the splitting field of some polynomial, and the degree of a normal extension of the order of its Galois group. We ilustrate these concept with several examples: the reducible polynomial x^4-5x^2+6, and the irreducible polynomial x^3-2.

[witness]

20:50 There is a mistake at the last line, |Gal(Q(cubic-root 2))|=2. There are 2 automorphisms to fix Q(cubic-root 2), which are:

#1, the identical mapping: id(cubic-root 2)=cubic-root 2, id(zeta)=zeta
#2, sigma(cubic-root 2)=cubic-root 2, sigma(zeta)=zeta^2

Another way to show it: let K=Q(cubic-root 2, zeta), E=Q(cubic-root 2), apparently, K is a Galois extension of E, hence

[ericway]

The formal definition of a normal extension is "an algebraic field extension L/K for which every irreducible polynomial over K which has a root in L, splits into linear factors in L." But there's also a theorem: "A field extension L/K is normal and finite if and only if L is a splitting field for some polynomial over K." Note that the extension needs to be finite in the theorem. So the definition in slide 5 should be

"A *finite* extension field E of F is normal if E is the splitting field for some polynomial f over F".

Consider the field of all algebraic numbers as an infinite algebraic extension of Q. Then this field extension is normal, but it is not a splitting field for some polynomial over Q. But since we're mainly concerned with finite field extensions in the lectures, this isn't a major problem. (Again, thank you so much for all the inspiring lectures!)

[rasraster]

My apologies for the criticism, but it seems to me that your formal definition of a normal extension is not well stated. I had to look up the definition in order to get a confirmation that it means the "all or none" interpretation you followed up with in the video. In any case, thanks for all these great lessons. They're very helpful to me.

[fsaldan]

In Wikipedia, under Normal Extension, one can see that a normal extension is the splitting field of an *irreducible* polynomial.

[ChuanChihChou]

8:05 Hmm, it's not obvious to me that the corollary follows the "One orbit theorem"?
The "One orbit theorem" only guarantees that there is an isomorphism ϕ from Q(r_1) -> Q(r_2) s.t. ϕ(r_1) = r_2
There is no (obvious) guarantee that such isomorphism can be extended into an automorphism of the splitting field, say Q(r_1, r_2)...

[ajabila]

In slide 5, not sure if I'm wrong but is it "If E is a normal extension.... that has a root in E splits over E" and not F? Just making sure I understand the idea. Thanks! :)

[hyperduality]

Automorphism = Duality -- conjugate root theorem.
"Sith lords come in pairs (duals)" -- Obi Wan Kenobi.
Injective is dual to surjective synthesizes bijective or isomorphism.
Subfields are dual to subgroups -- the Galois correspondence.
"Always two there are" -- Yoda.
Brahman (thesis, the creator God) is dual to Shiva (anti-thesis, the destroyer God) synthesizes Vishnu (the preserver God) -- the time independent Hegelian dialectic.
Hinduism is consistent with the Hegelian dialectic!
Duality (thesis, anti-thesis) creates reality (non duality).