filmov
tv
Visual Group Theory, Lecture 1.2: Cayley graphs
Показать описание
Visual Group Theory, Lecture 1.2: Cayley graphs
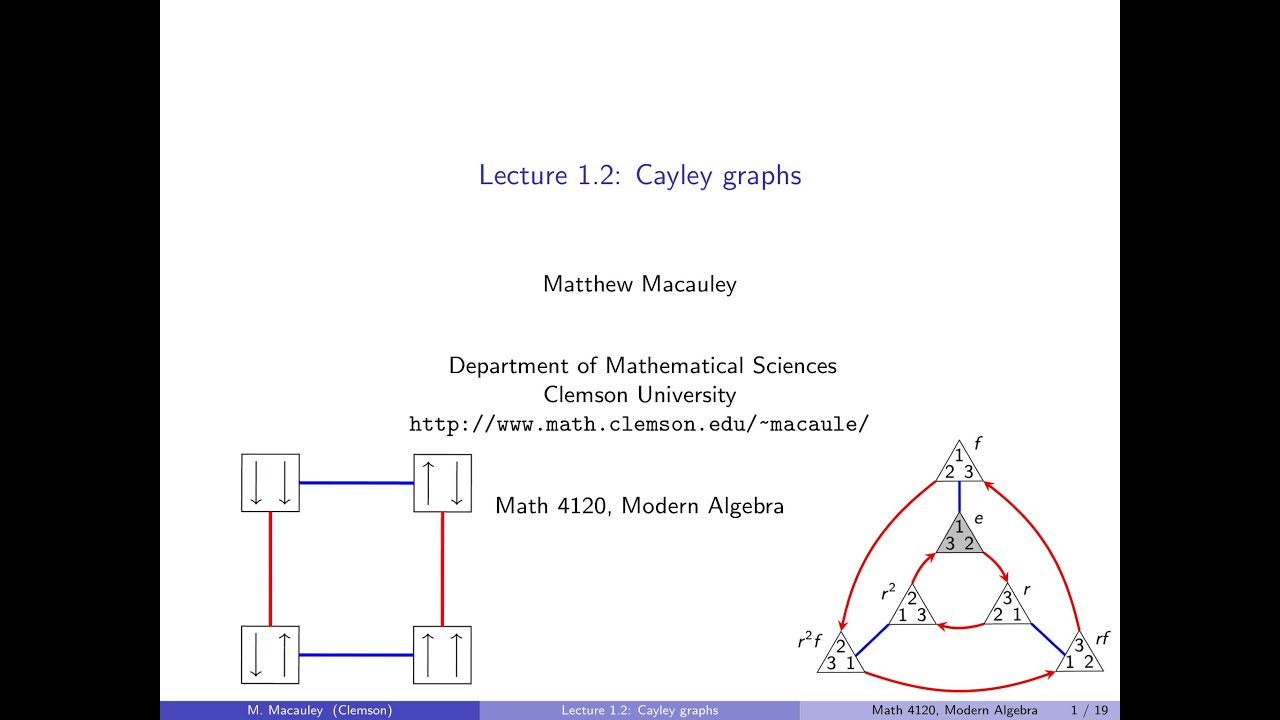
In this lecture, we introduce a visual way to "map out" a group using an object called a Cayley graph. This concept is a useful visualization tool, but it is often omitted entirely from traditional Abstract Algebra classes.
In this lecture, we introduce a visual way to "map out" a group using an object called a Cayley graph. This concept is a useful visualization tool, but it is often omitted entirely from traditional Abstract Algebra classes.
Visual Group Theory, Lecture 1.2: Cayley graphs
Visual Group Theory, Lecture 2.1: Cyclic and abelian groups
Visual Group Theory, Lecture 1.1: What is a group?
Visual Group Theory, Lecture 1.6: The formal definition of a group
Visual Group Theory, Lecture 5.1: Groups acting on sets
Visual Group Theory, Lecture 3.1: Subgroups
Grant Sanderson (3Blue1Brown): Best Way to Learn Math | AI Podcast Clips
Visual Group Theory, Lecture 6.1: Fields and their extensions
Visual Group Theory, Lecture 1.3: Groups in science, art, and mathematics
Group theory, abstraction, and the 196,883-dimensional monster
Visual Group Theory, Lecture 1.5: Multiplication tables
Visual Group Theory, Lecture 4.1: Homomorphisms and isomorphisms
Visual Group Theory, Lecture 4.6: Automorphisms
Visual Group Theory, Lecture 5.6: The Sylow theorems
Visual Group Theory, Lecture 2.3: Symmetric and alternating groups
Visual Group Theory, Lecture 1.4: Group presentations
Visual Group Theory, Lecture 5.2: The orbit-stabilizer theorem
Visual Group Theory, Lecture 4.2: Kernels
Lecture 1
Visual Group Theory, Lecture 5.7: Finite simple groups
Visual Group Theory, Lecture 3.7: Conjugacy classes
Visual Group Theory, Lecture 4.3: The fundamental homomorphism theorem
Chapter 1: Symmetries, Groups and Actions | Essence of Group Theory
Visual Group Theory, Lecture 2.4: Cayley's theorem
Комментарии
 0:33:27
0:33:27
 0:30:45
0:30:45
 0:16:15
0:16:15
 0:12:48
0:12:48
 0:32:35
0:32:35
 0:13:43
0:13:43
 0:03:22
0:03:22
 0:26:34
0:26:34
 0:30:36
0:30:36
 0:21:58
0:21:58
 0:19:24
0:19:24
 0:47:18
0:47:18
 0:24:34
0:24:34
 0:48:37
0:48:37
 0:29:50
0:29:50
 0:18:12
0:18:12
 0:27:41
0:27:41
 0:31:32
0:31:32
 0:34:05
0:34:05
 0:36:34
0:36:34
 0:39:19
0:39:19
 0:32:52
0:32:52
 0:06:07
0:06:07
 0:11:00
0:11:00