filmov
tv
Visual Group Theory, Lecture 2.3: Symmetric and alternating groups
Показать описание
Visual Group Theory, Lecture 2.3: Symmetric and alternating groups
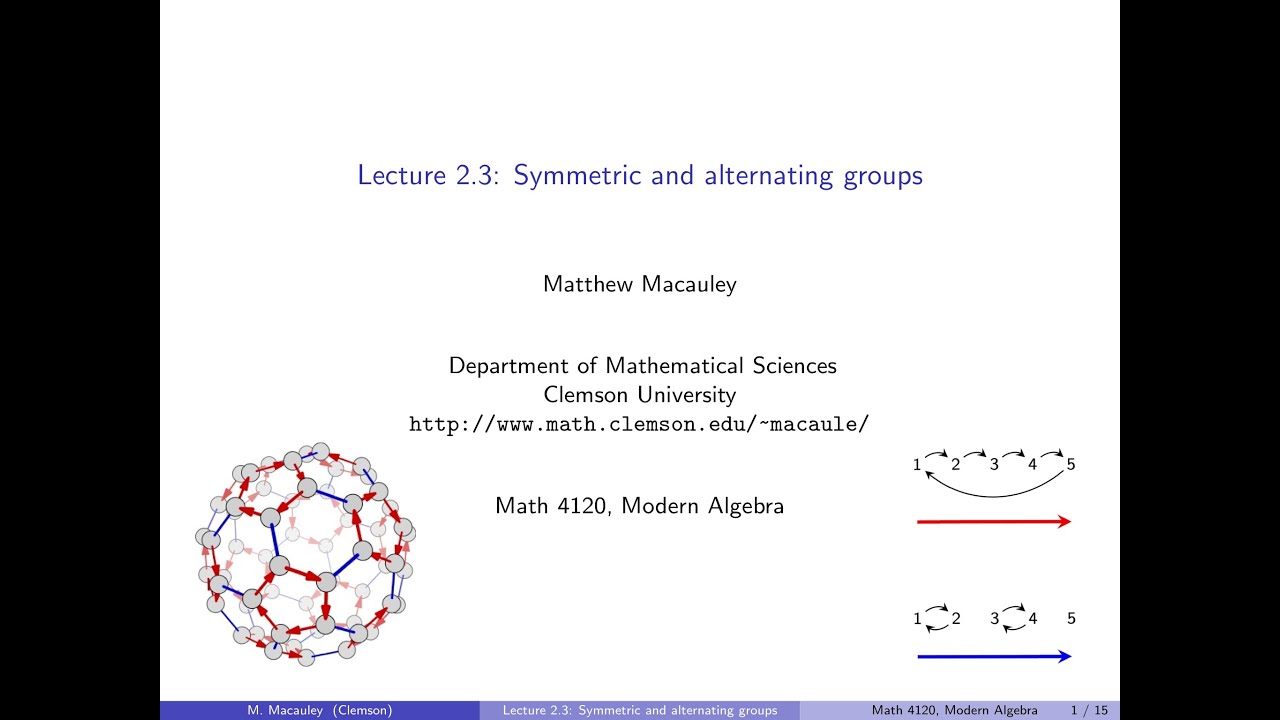
In this lecture, we introduce the last two of our "5 families" of groups: (4) symmetric groups and (5) alternating groups. The symmetric group S_n is the group of all n! permutations of {1,...,n}. We see several different ways to describe permutations, though we primarily stick with the "cycle notation". The alternating group A_n is a subgroup of S_n, and it consists of the permutations that can be written as a product of an even number of transpositions. There are exactly five regular three-dimensional convex polyhedra (tetrahedron, cube, octahedron, dodecahedron, icosahedron), and the symmetry groups of each of these are symmetric or alternating groups.
In this lecture, we introduce the last two of our "5 families" of groups: (4) symmetric groups and (5) alternating groups. The symmetric group S_n is the group of all n! permutations of {1,...,n}. We see several different ways to describe permutations, though we primarily stick with the "cycle notation". The alternating group A_n is a subgroup of S_n, and it consists of the permutations that can be written as a product of an even number of transpositions. There are exactly five regular three-dimensional convex polyhedra (tetrahedron, cube, octahedron, dodecahedron, icosahedron), and the symmetry groups of each of these are symmetric or alternating groups.
Visual Group Theory, Lecture 2.3: Symmetric and alternating groups
Visual Group Theory, Lecture 1.2: Cayley graphs
Visual Group Theory, Lecture 2.1: Cyclic and abelian groups
Visual Group Theory, Lecture 3.2: Cosets
Visual Group Theory, Lecture 1.3: Groups in science, art, and mathematics
Grant Sanderson (3Blue1Brown): Best Way to Learn Math | AI Podcast Clips
Visual Group Theory, Lecture 3.6: Normalizers
Group theory, abstraction, and the 196,883-dimensional monster
Visual Group Theory, Lecture 3.7: Conjugacy classes
Visual Group Theory, Lecture 4.6: Automorphisms
Visual Group Theory, Lecture 2.2: Dihedral groups
Visual Group Theory, Lecture 5.2: The orbit-stabilizer theorem
Visual Group Theory, Lecture 1.1: What is a group?
Visual Group Theory, Lecture 6.5: Galois group actions and normal field extensions
Lecture 3 || Composition Table, Addition Modulo, Multiplication Modulo || B.A. / B.Sc. Mathematics
Visual Group Theory, Lecture 5.1: Groups acting on sets
Visual Group Theory, Lecture 3.5: Quotient groups
Visual Group Theory, Lecture 6.1: Fields and their extensions
Visual Group Theory, Lecture 5.7: Finite simple groups
Visual Group Theory, Lecture 2.4: Cayley's theorem
Group Theory, lecture 1.4: Examples
Group Theory, lecture 3.1: Cosets
Visual Group Theory, Lecture 1.4: Group presentations
Visual Group Theory, Lecture 3.4: Direct products
Комментарии
 0:29:50
0:29:50
 0:33:27
0:33:27
 0:30:45
0:30:45
 0:19:11
0:19:11
 0:30:36
0:30:36
 0:03:22
0:03:22
 0:22:46
0:22:46
 0:21:58
0:21:58
 0:39:19
0:39:19
 0:24:34
0:24:34
 0:16:14
0:16:14
 0:27:41
0:27:41
 0:16:15
0:16:15
 0:26:28
0:26:28
 0:44:44
0:44:44
 0:32:35
0:32:35
 0:40:38
0:40:38
 0:26:34
0:26:34
 0:36:34
0:36:34
 0:11:00
0:11:00
 0:23:36
0:23:36
 0:20:29
0:20:29
 0:18:12
0:18:12
 0:23:16
0:23:16