Visual Group Theory, Lecture 4.2: Kernels

preview_player
Показать описание
Visual Group Theory, Lecture 4.2: Kernels

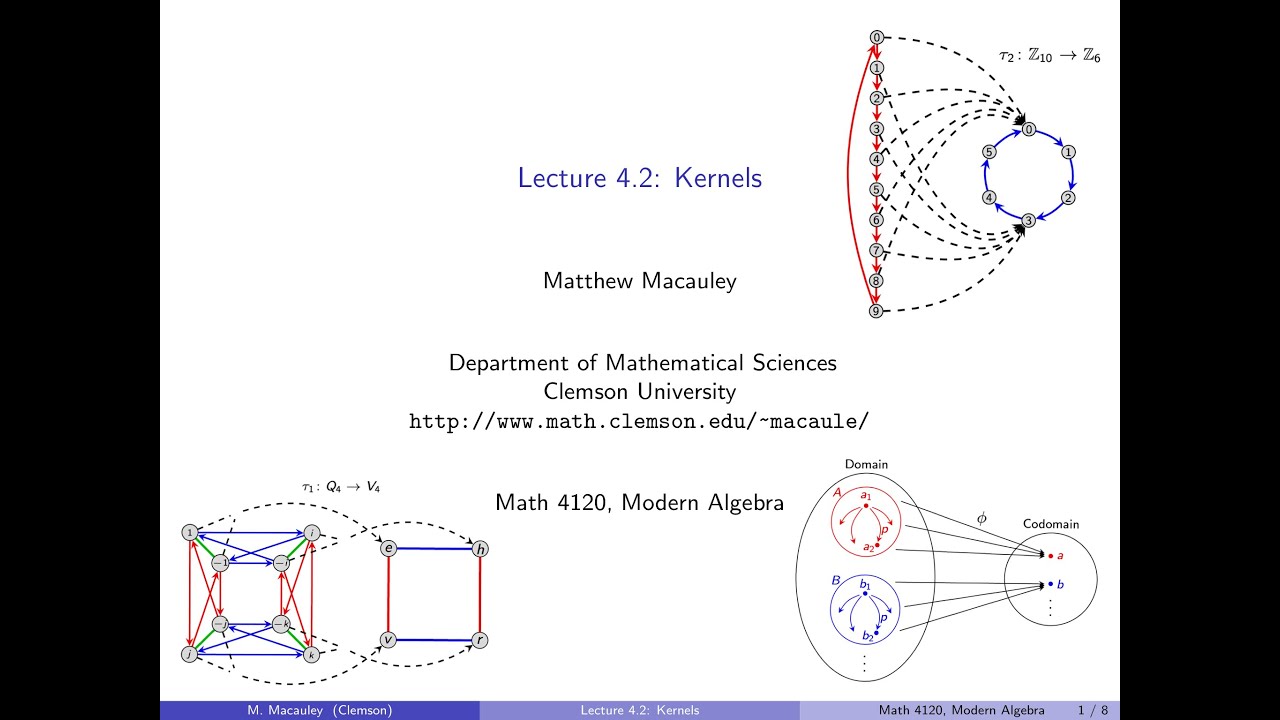
The kernel of a homomorphism is the set of elements that get mapped to the identity. We show that it is always a normal subgroup of the domain, and that the preimages of the other elements are its cosets. This means that we can always quotient out by the kernel, and this key observation leads us to the fundamental homomorphism theorem. We concluding with two visual examples: one using multiplication tables, and the other using Cayley diagrams.

  1. Professor Macauley
  2. Mathematics
  3. Group theory
  4. Visual group theory
  5. Homomorphism
  6. Kernel
Рекомендации по теме
Visual Group Theory, 0:11:00

Visual Group Theory, Lecture 2.4: Cayley's theorem

Visual Group Theory, 0:27:41

Visual Group Theory, Lecture 5.2: The orbit-stabilizer theorem

Visual Group Theory, 0:18:12

Visual Group Theory, Lecture 1.4: Group presentations

Visual Group Theory, 0:29:50

Visual Group Theory, Lecture 2.3: Symmetric and alternating groups

Visual Group Theory, 0:33:27

Visual Group Theory, Lecture 1.2: Cayley graphs

Visual Group Theory, 0:30:45

Visual Group Theory, Lecture 2.1: Cyclic and abelian groups

Visual Group Theory, 0:23:16

Visual Group Theory, Lecture 3.4: Direct products

Visual Group Theory, 0:24:34

Visual Group Theory, Lecture 4.6: Automorphisms

Visual Group Theory, 0:47:18

Visual Group Theory, Lecture 4.1: Homomorphisms and isomorphisms

Visual Group Theory, 0:46:19

Visual Group Theory, Lecture 4.5: The isomorphism theorems

Visual Group Theory, 0:19:11

Visual Group Theory, Lecture 3.2: Cosets

Visual Group Theory, 0:12:48

Visual Group Theory, Lecture 1.6: The formal definition of a group

Visual Group Theory, 0:34:13

Visual Group Theory, Lecture 6.4: Galois groups

Visual Group Theory, 0:36:34

Visual Group Theory, Lecture 5.7: Finite simple groups

Visual Group Theory, 0:26:28

Visual Group Theory, Lecture 6.5: Galois group actions and normal field extensions

Visual Group Theory, 0:24:47

Visual Group Theory, Lecture 4.4: Finitely generated abelian groups

Visual Group Theory, 0:32:52

Visual Group Theory, Lecture 4.3: The fundamental homomorphism theorem

Visual Group Theory, 0:14:00

Visual Group Theory, Lecture 3.3: Normal subgroups

Visual Group Theory, 0:16:14

Visual Group Theory, Lecture 2.2: Dihedral groups

Visual Group Theory, 0:26:34

Visual Group Theory, Lecture 6.1: Fields and their extensions

Visual Group Theory, 0:35:41

Visual Group Theory, Lecture 6.2: Field automorphisms

Visual Group Theory, 0:32:35

Visual Group Theory, Lecture 5.1: Groups acting on sets

Visual Group Theory, 0:13:43

Visual Group Theory, Lecture 3.1: Subgroups

Visual Group Theory, 0:39:19

Visual Group Theory, Lecture 3.7: Conjugacy classes

Комментарии
Автор

truly fantastic, thank you for uploading

zpie
Автор

Thanks for preparing and posting these videos. I've been studying group theory for years; nevertheless, I'd not seen a presentation of group theory in terms of Cayley diagrams, so while watching your videos, I'm still learning something new. It's wonderful to have these new perspectives / "languages" with which to study group theory.

kevinbyrne
Автор

Nobody:
Millennials in Textmessages: 30:33

em-ildq
Автор

May I point out two small issues with your sketch of proving that the preimage of h is a subset of gK? (1) you never posit that phi(g) = h, so there is not enough info to prove g' and g are in the same coset, and (2) at the end, g'=gk should be phrased as g' = gx and the reader should determine that x is in K.

rasraster
Автор

In the proof, weren't you also required to show that if phi(a) <> phi(b) then a <> b?

rasraster