filmov
tv
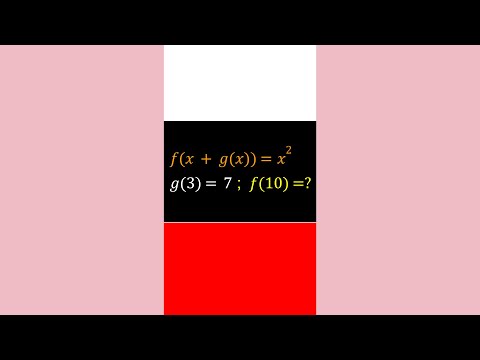
Solving Two Functional Equations

Показать описание
If you need to post a picture of your solution or idea:
#ChallengingMathProblems #FunctionalEquations
PLAYLISTS 🎵 :
#ChallengingMathProblems #FunctionalEquations
PLAYLISTS 🎵 :
Solving Two Functional Equations
5 simple unsolvable equations
Methods of Functional Equations
Slick Solutions to Functional Equations
A Functional Equation with Two Functions
A Nice and Easy Functional Equation
Solving a Functional Equation
CMU Putnam Seminar 10/2 Functional Equations
Gradient of a straight line. #math #mathforfun #mathteacher #mathstudent #mathematics #maths
Solving a Quick and Easy Functional Equation
Solving a Functional Equation | f(x)+f(x-1)=x^2
A Quick and Easy Functional Equation
Weak Spots of Functional Equations | International Mathematical Olympiad 2011 Shortlist A3
Have You Solved a Functional Equation Before?
A Functional Equation from Samara Math Olympiads
Functional Equation
Solving a Homemade Functional Equation in Two Ways
Solving A Functional Equation
A Functional Equation from Putnam and Beyond
Solving a Radical Functional Equation in Two Ways
A Quick and Easy Functional Equation
Solving a Rational Functional Equation
A Rational Functional Equation Solved in Two Ways
A Functional Equation with Two Functions
Комментарии
 0:08:50
0:08:50
 0:00:50
0:00:50
 0:07:40
0:07:40
 0:08:22
0:08:22
 0:06:56
0:06:56
 0:05:07
0:05:07
 0:10:36
0:10:36
 0:58:00
0:58:00
 0:01:01
0:01:01
 0:05:47
0:05:47
 0:08:59
0:08:59
 0:06:49
0:06:49
 0:12:27
0:12:27
 0:00:40
0:00:40
 0:08:47
0:08:47
 0:14:15
0:14:15
 0:10:26
0:10:26
 0:00:29
0:00:29
 0:12:07
0:12:07
 0:09:16
0:09:16
 0:00:45
0:00:45
 0:06:53
0:06:53
 0:08:51
0:08:51
 0:00:39
0:00:39