filmov
tv
Visual Group Theory: Lecture 7.4: Divisibility and factorization
Показать описание
Visual Group Theory: Lecture 7.4: Divisibility and factorization
The ring of integers have a number of properties that we take for granted: every number can be factored uniquely into primes, and all pairs of numbers have a unique gcd and lcm. In this lecture, we investigate when this happens in other integral domains. We define what it means for elements to be prime and to be irreducible. Prime implies irreducible, except the converse fails unless we are in a special ring called a "principal ideal domain" (PID). There are rings for which every ideal is generated by a single element, and they have other nice properties. For example, every pair of elements in a PID has a unique gcd and lcm. A larger class of rings, for which some of these properties still old are the unique factorization domains (UFD). We conclude with a summary of the types of rings we've seen so far: fields, PIDs, UFD, integral domains, and commutative rings, and how they fit together.
The ring of integers have a number of properties that we take for granted: every number can be factored uniquely into primes, and all pairs of numbers have a unique gcd and lcm. In this lecture, we investigate when this happens in other integral domains. We define what it means for elements to be prime and to be irreducible. Prime implies irreducible, except the converse fails unless we are in a special ring called a "principal ideal domain" (PID). There are rings for which every ideal is generated by a single element, and they have other nice properties. For example, every pair of elements in a PID has a unique gcd and lcm. A larger class of rings, for which some of these properties still old are the unique factorization domains (UFD). We conclude with a summary of the types of rings we've seen so far: fields, PIDs, UFD, integral domains, and commutative rings, and how they fit together.
0:30:09
Visual Group Theory: Lecture 7.5: Euclidean domains and algebraic integers
0:32:36
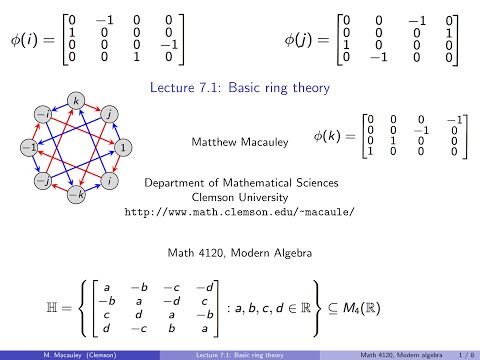
Visual Group Theory, Lecture 7.1: Basic ring theory
0:34:20
Visual Group Theory, Lecture 7.2: Ideals, quotient rings, and finite fields
0:36:34
Visual Group Theory, Lecture 5.7: Finite simple groups
0:39:19
Visual Group Theory, Lecture 3.7: Conjugacy classes
0:48:37
Visual Group Theory, Lecture 5.6: The Sylow theorems
0:26:28
Visual Group Theory, Lecture 6.5: Galois group actions and normal field extensions
0:26:34
Visual Group Theory, Lecture 6.1: Fields and their extensions
0:24:34
Visual Group Theory, Lecture 4.6: Automorphisms
0:13:22
Group Theory Lecture 7.4 Coset Action
0:32:35
Visual Group Theory, Lecture 5.1: Groups acting on sets
0:30:36
Visual Group Theory, Lecture 1.3: Groups in science, art, and mathematics
0:30:45
Visual Group Theory, Lecture 2.1: Cyclic and abelian groups
0:34:13
Visual Group Theory, Lecture 6.4: Galois groups
0:29:50
Visual Group Theory, Lecture 2.3: Symmetric and alternating groups
0:10:18
Abstract Algebra | The Alternating Group
0:53:13
Group Theory: Lecture 7/30 - Disjoint Cycles
0:32:52
Visual Group Theory, Lecture 4.3: The fundamental homomorphism theorem
0:33:27
Visual Group Theory, Lecture 1.2: Cayley graphs
0:22:46
Visual Group Theory, Lecture 3.6: Normalizers
0:46:19
Visual Group Theory, Lecture 4.5: The isomorphism theorems
0:10:51
Chapter 7: Group actions, symmetric group and Cayley’s theorem | Essence of Group Theory
0:23:01
Galois Theory Lecture 7
0:12:03
Chapter 4: Conjugation, normal subgroups and simple groups | Essence of Group Theory
Комментарии