QIP2021 | Tsirelson's problem and MIP*=RE (Thomas Vidick)

Authors: Zhengfeng Ji, Anand Natarajan, Thomas Vidick, John Wright, Henry Yuen

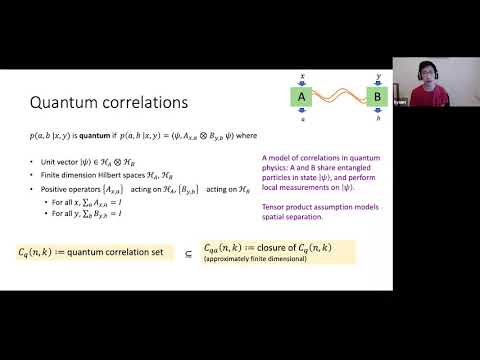
Boris Tsirelson in 1993 implicitly posed "Tsirelson's Problem", a question about the possible equivalence between two different ways of modeling locality, and hence entanglement, in quantum mechanics. Tsirelson's Problem gained prominence through work of Fritz, Navascues et al., and Ozawa a decade ago that establishes its equivalence to the famous "Connes' Embedding Problem" in the theory of von Neumann algebras.

Recently we gave a negative answer to Tsirelson's Problem and Connes' Embedding Problem by proving a seemingly stronger result in quantum complexity theory. This result is summarized in the equation MIP* = RE between two complexity classes.

In the talk I will present and motivate Tsirelson's problem, and outline its connection to Connes' Embedding Problem. I will then explain the connection to quantum complexity theory and show how ideas developed in the past two decades in the study of classical and quantum interactive proof systems led to the characterization (which I will explain) MIP* = RE and the negative resolution of Tsirelson's Problem.

Based on joint work with Ji, Natarajan, Wright and Yuen available at arXiv:2001.04383.

Get entangled with us!