filmov
tv
ICPCU by Henry Yuen: A Tale of Turing Machines, Quantum-Entangled Particles, and Operator Algebras

Показать описание
In a recent result known as "MIP* = RE," ideas from three disparate fields of study — computational complexity theory, quantum information, and operator algebras — have come together to simultaneously resolve long-standing open problems in each field, including a 44-year old mystery in mathematics known as Connes’ Embedding Problem. In this talk, I will describe the evolution and convergence of ideas behind MIP* = RE: it starts with three landmark discoveries from the 1930s (Turing’s notion of a universal computing machine, the phenomenon of quantum entanglement, and von Neumann’s theory of operators), and ends with some of the most cutting-edge developments from theoretical computer science and quantum computing.
ICPCU by Henry Yuen: A Tale of Turing Machines, Quantum-Entangled Particles, and Operator Algebras
Henry Yuen: An overview of MIP* = RE and some of its ingredients
insideQuantum S2E8: Quantum Complexity with Dr Henry Yuen
Henry Yuen - Crash Course (Part 1B)
MIP* = RE - Henry Yuen
Henry Yuen - Crash Course (Part 3)
Henry Yuen: Testing low-degree polynomials in the noncommutative setting
Henry Yuen (Columbia University)
Spooky Action Explained 👻 By A Columbia Quantum Expert
Multiprover Protocols I
Summer Cluster in Quantum Computation | Polylogues
Multiprover Protocols II
Interactive Proofs for Synthesizing Quantum States and Unitaries
MIP* = RE
Parallel Repetition for Entangled Games via Fast Quantum Search
Perfect zero knowledge for quantum multiprover interactive proofs
Quantum certified deletion with Anne Broadbent | Episode 3
Interactive proofs for synthesizing quantum states and unitaries - Gregory Rosenthal
QIP2021 | Tsirelson's problem and MIP*=RE (Thomas Vidick)
TQC 2020: Day 2
Panel Discussion on The Role of Proofs in MIP* = RE | Quantum Colloquium
MIP* = RE Part 1: The Quantum Low-Degree Test
Jakub Tarnawski: Approximation Algorithms for the (Asymmetric) Traveling Salesman Problem
Dr Dries Sels, Flatiron Institute/NYU
Комментарии
 0:45:06
0:45:06
 1:29:01
1:29:01
 0:47:20
0:47:20
 0:21:48
0:21:48
 0:58:54
0:58:54
 0:59:09
0:59:09
 1:09:24
1:09:24
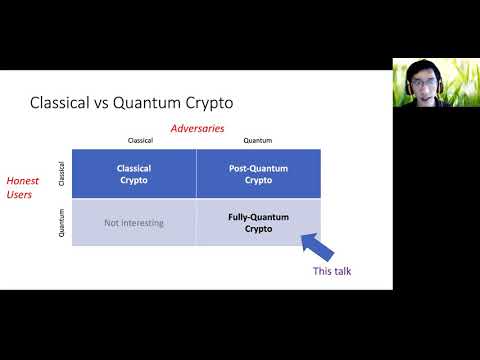 0:55:54
0:55:54
 0:02:04
0:02:04
 0:53:51
0:53:51
 0:31:33
0:31:33
 0:51:14
0:51:14
 0:45:16
0:45:16
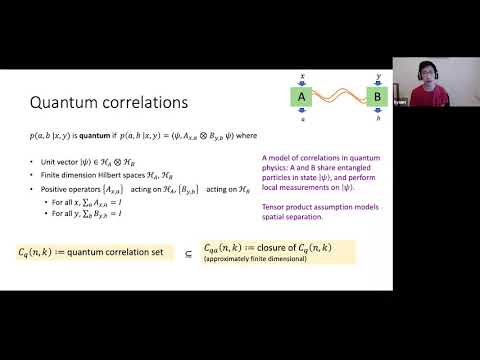 0:59:49
0:59:49
 0:28:59
0:28:59
 0:22:08
0:22:08
 0:35:21
0:35:21
 0:41:02
0:41:02
 0:54:18
0:54:18
 6:38:59
6:38:59
 0:44:53
0:44:53
 1:10:10
1:10:10
 0:54:08
0:54:08
 1:02:33
1:02:33