filmov
tv
Tsirelson's space

Показать описание
In 1974, Tsirelson constructed a Banach space that contains no subspace isomorphic to c_0 or l_p. In this video we establish all the preliminary notions required to define Tsirelson's space, describe its construction, and then prove that no subspace is isomorphic to c_0 or l_p.
The notes described in the video can be found at this link:
I hoped the video would be under an hour, but I guess I failed. It would have been even longer if I included all of the proofs, so sadly I had to omit most of them, but they are in the notes above for your careful scrutiny.
0:00 Preamble and basic definitions
11:15 Schauder bases
28:25 Definition of Tsirelson's space and lemmas
45:46 Proof that l_1 does not embed into T
1:05:52 Proof that T does not contain c_0 or l_p
(This video was created for the class MATH 567 Introduction to Functional Analysis for the Fall 2021 semester at McGill University.)
The notes described in the video can be found at this link:
I hoped the video would be under an hour, but I guess I failed. It would have been even longer if I included all of the proofs, so sadly I had to omit most of them, but they are in the notes above for your careful scrutiny.
0:00 Preamble and basic definitions
11:15 Schauder bases
28:25 Definition of Tsirelson's space and lemmas
45:46 Proof that l_1 does not embed into T
1:05:52 Proof that T does not contain c_0 or l_p
(This video was created for the class MATH 567 Introduction to Functional Analysis for the Fall 2021 semester at McGill University.)
Tsirelson's space
#21: Pete Casazza - On undefinability of Tsirelson's space
Tsirelson space | Wikipedia audio article
William Slofstra:Tsirelson’s problem & an embedding theorem for groups arising from non-local ga...
Lecture Notes in Mathematics Tsirelson's Space Peter G Casazza, T J Shura,R Aron
QIP2021 | Tsirelson's problem and MIP*=RE (Thomas Vidick)
#3: Kevin Beanland- Closed ideals of operators on Tsirelson space
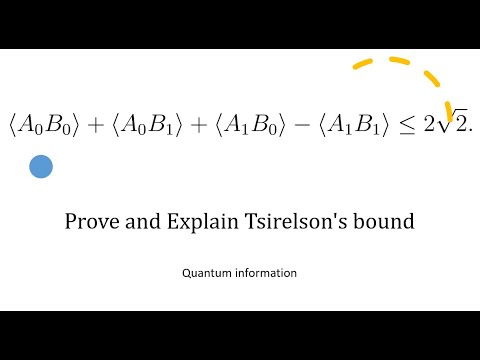
Prove and Explain Tsirelson's bound
Thomas Vidick: Connes embedding problem, Tsirelson’s problem, and MIP^{∗} = RE
ZAW LIN HTOO: Detecting quantumness and entanglement with uniform precessions
An Underlying Coherence Between Special Relativity and Quantum Mechanics
Advanced Topics in Quantum Information Theory: Lecture 6
Juan León - Remote states, Reeh-Schlieder theorem...
#17: Niels Laustsen - A C(K)-space with few operators and few decompositions
Prof. Vern Paulsen | Embezzlement of entanglement
C*-algebras and Synchronous Games
Travis Russell, Quantum correlations via Operator Systems, 2022.09.20
The Role of Proofs in MIP* = RE | Quantum Colloquium
Retrocausality as an Axiom | Daniel Rohrlich
Why was Connes' embedding conjecture refuted and there are still no known... -Michael Chapman
Quantum correlations, tensor norms, and factorizable quantum channels
Schlumprecht, Zsák, Freeman: Banach spaces for which the space of operators has 2^𝔠 closed ideals...
Ph CS 219A Lecture 7A Bell Polytope
#38 Thomas Schlumprecht- Banach spaces with lots of closed operator ideals
Комментарии
 1:20:15
1:20:15
 0:52:14
0:52:14
 0:07:13
0:07:13
 0:51:05
0:51:05
 0:18:20
0:18:20
 0:54:18
0:54:18
 1:03:32
1:03:32
 0:12:38
0:12:38
 0:45:26
0:45:26
 0:53:54
0:53:54
 0:20:46
0:20:46
 0:39:34
0:39:34
 0:35:37
0:35:37
 0:56:36
0:56:36
 1:37:54
1:37:54
 0:46:08
0:46:08
 0:52:54
0:52:54
 1:02:30
1:02:30
 0:54:24
0:54:24
 1:51:50
1:51:50
 0:51:49
0:51:49
 2:02:45
2:02:45
 1:17:49
1:17:49
 1:10:18
1:10:18