Visual Group Theory, Lecture 3.1: Subgroups

preview_player
Показать описание
Visual Group Theory, Lecture 3.1: Subgroups

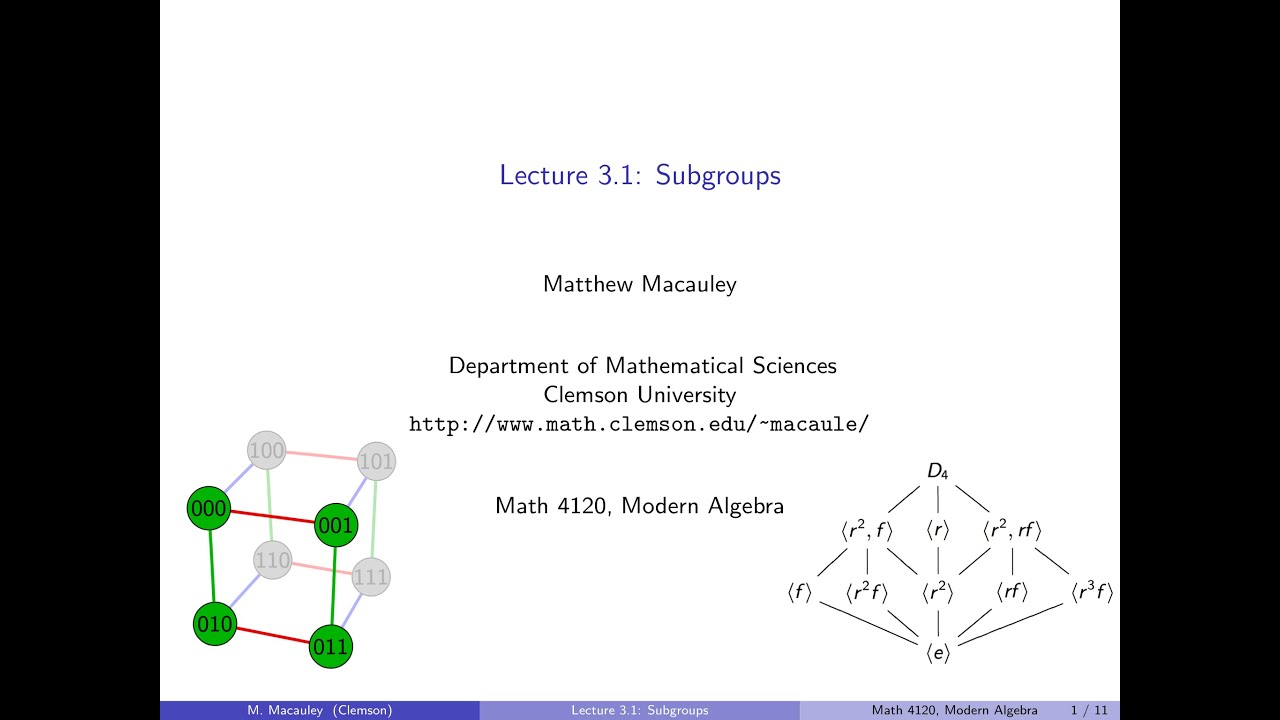
In this lecture, we begin by examining a property about Cayley graphs called "regularity" that we've hinted at but not yet spelled out explicitly. Next, we introduce the concept of a subgroup, provide some examples, and show how the subgroups of any group can be arranged in a lattice.

  1. Professor Macauley
  2. Mathematics
  3. Visual group theory
  4. Group theory
  5. Subgroups
  6. Orbits
Рекомендации по теме
Visual Group Theory, 0:30:36

Visual Group Theory, Lecture 1.3: Groups in science, art, and mathematics

Visual Group Theory, 0:22:46

Visual Group Theory, Lecture 3.6: Normalizers

Visual Group Theory, 0:16:15

Visual Group Theory, Lecture 1.1: What is a group?

Visual Group Theory, 0:30:45

Visual Group Theory, Lecture 2.1: Cyclic and abelian groups

Visual Group Theory, 0:39:19

Visual Group Theory, Lecture 3.7: Conjugacy classes

Visual Group Theory, 0:40:38

Visual Group Theory, Lecture 3.5: Quotient groups

Group theory, abstraction, 0:21:58

Group theory, abstraction, and the 196,883-dimensional monster

Visual Group Theory, 0:18:12

Visual Group Theory, Lecture 1.4: Group presentations

Visual Group Theory, 0:33:27

Visual Group Theory, Lecture 1.2: Cayley graphs

Visual Group Theory, 0:29:50

Visual Group Theory, Lecture 2.3: Symmetric and alternating groups

Visual Group Theory, 0:32:35

Visual Group Theory, Lecture 5.1: Groups acting on sets

Visual Group Theory, 0:23:16

Visual Group Theory, Lecture 3.4: Direct products

Visual Group Theory, 0:14:00

Visual Group Theory, Lecture 3.3: Normal subgroups

Visual Group Theory, 0:19:11

Visual Group Theory, Lecture 3.2: Cosets

Visual Group Theory, 0:24:34

Visual Group Theory, Lecture 4.6: Automorphisms

Lecture 3 || 0:44:44

Lecture 3 || Composition Table, Addition Modulo, Multiplication Modulo || B.A. / B.Sc. Mathematics

Visual Group Theory, 0:26:34

Visual Group Theory, Lecture 6.1: Fields and their extensions

Group Theory, lecture 0:23:36

Group Theory, lecture 1.4: Examples

Group Theory, lecture 0:20:29

Group Theory, lecture 3.1: Cosets

Visual Group Theory, 0:47:18

Visual Group Theory, Lecture 4.1: Homomorphisms and isomorphisms

The Group Theory 0:01:00

The Group Theory Used to Solve the Hardest Differential Equation

Visual Group Theory, 0:22:46

Visual Group Theory, Lecture 6.7: Ruler and compass constructions

Visual Group Theory, 0:38:20

Visual Group Theory, Lecture 6.3: Polynomials and irreducibility

Chapter 1: Symmetries, 0:06:07

Chapter 1: Symmetries, Groups and Actions | Essence of Group Theory

Комментарии
Автор

I don't think that `<=` and `<` are supposed to mean the same. First is for subgroups (which can be of the same size as the original group), and the other is for _proper_ subgroups (which are strictly smaller). A group can be a subgroup of itself, but it is not a _proper_ subgroup.
I have a question though: Is there a formula for the number of all subgroups of a given group? Or a better algorithm of finding all subgroups? From what I see, it can be related in some way to prime factorization and all possible divisors.

scitwi
Автор

G is <= G, but G is not < G. That's a pretty big difference. Otherwise your subgroup lattice at 12:05 would be infinitely recursive.

ernestkirstein
Автор

At 10:40 one of the Cayley's diagram of Z_6 coincides with one of the Cayley diagram of D_3. So they are isomorphic, but Z_6 is cyclic and D_3 is not. Where am I wrong?

laflaca