Visual Group Theory, Lecture 6.3: Polynomials and irreducibility

Visual Group Theory, Lecture 6.3: Polynomials and irreducibility

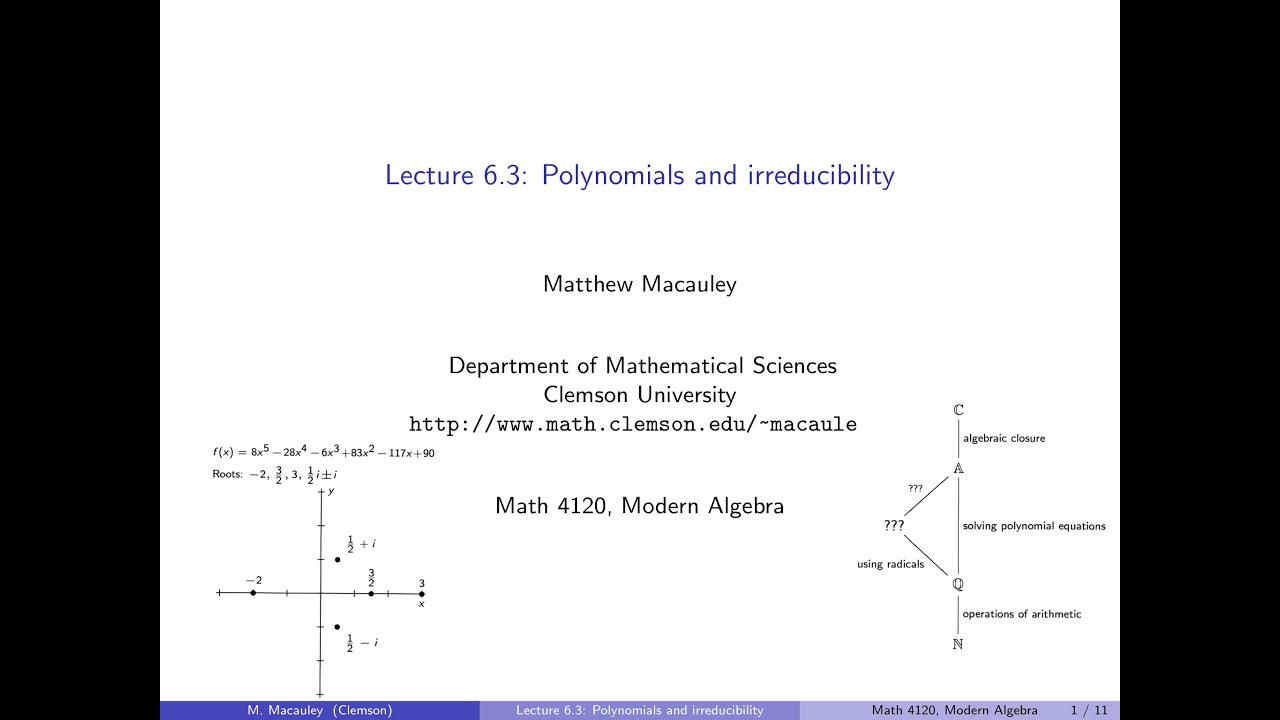
A complex number is algebraic over Q (the rationals) if it is the root of a polynomial with rational coefficients. It is clear that every number that can be written with arithmetic and radicals is rational. Galois' big achievement was proving that the converse fails. In fact, there are roots of degree-5 polynomials that cannot be expressed using radicals, which means that there cannot exist a formula for the quintic. Next, we turn to the question of when a polynomial is irreducible over Q, which means that it cannot be factored into a product of two lower-degree polynomials. Though there is no one sure-fire method to answer this, a technique called Eisenstein's criterion works for many polynomials. We conclude by looking at extensions of Q, and define the minimal polynomial of an algebraic number r to be the (unique) monic irreducible polynomial for which f(r)=0. It turns out that the degree of this "minimal polynomial" is equal to the dimension of the extension field Q(r) over Q.

[ub]

I think there is a mistake around 6:30, where phi, the golden ratio, is given as an example of a transcendental number. It is a root of the polynomial x^2 - x - 1, and so it is algebraic.

[MrWorshipMe]

I think you mean that a complex conjugate of a root is also a root in the case that our polynomial is not made of complex coefficients.
A trivial counter example would be f(x)=x+i, where the solution would be x=-i, but not it's complex conjugate.

[davidturner]

14:24 should be: "The roots of f(x) <- R[x] come in conjugate pairs". The roots of C[x] may come any way they like.

[ub]

I'll add that phi is (1+sqrt(5))/2.

[shlokamsrivastava]

This channel deserves more subscribers

[kynnjones]

As Robert Bowman correctly pointed out, the golden ratio Φ is *not* transcendental. These lectures have a lot of great content, but they contain so many errors (like this one) that I think it borders on irresponsible to leave them up without any adequate corrections.

[PhilippeZivanSussholz]

I want to congratulate you for the wonderful lectures.
Interestingly you chose to first of all show the relationship misterious relationship between Aut(G) and Gal(F) and on later lectures you uncovered the terms like irreducible polynomials etc..
BRILLIANT

[alexheaton]

Thank you for these videos! Also, near 11:00 that slide makes it seem like the complex numbers are the algebraic closure of the rationals. But usually when we say complex numbers we mean with real coefficients, so pi is an element of C, but pi is not an element of the algebraic closure of the rationals?

[cf]

At 17:30, there is a mistake: all linear polynomials x-c are clearly irreducible over C.

[StefanHoffmann]

At 10:50 the field Q(sqrt 2) should sit between all numbers that are expressible with radicals and Q as obviously every expression a + b sqrt(2) is an expression in radicals, but you write it as if they where incomparable in the Hasse diagram. But nice lecture overall, thanks for the effort!

[sftw]

8:18, Third double arrow is not an arrow to the left

[imcruel]

pi is a computable number, while it is true that there are uncomputable numbers, not all irrationals and not even all nonalgebraic numbers an uncomputable.

[gaaraofddarkness]

According to me, Phee is NOT transcendental sir.

[humamsebai]

6:37 the golden ratio is an irrational number but it is not a transcendental number.

[hyperduality]

Conjugate root theorem implies duality, roots come in pairs!
Conjugate = Duality!
"Sith lords come in pairs (duals)" -- Obi Wan Kenobi.
"Always two there are" -- Yoda.

[pmccarthy]

14:30 As I believe some other posters have pointed out: Roots of the polynomial f(x), all of whose coefficients are an element of C[x] come in conjugate pairs is false. Roots of the polynomial f(x), all of whose coefficients are an element of R[x] come in conjugate pairs is true, however.

[tourneriealexandre]

There is a mistake at 14:20, for example : f(X) =X-i admit one root i but - i is not a root.
The solution is f is polynomial with REAL coefficient.

[miloszforman]

"Even weirder, since a computer program is just a string of 0s and 1s, there are only countably infinite many possible programs."

Now wait a minute ... there is no way to count all possible (i. e. "functioning" programs), as there is no such algorithm (this is somehow related to the "halting problem"). So the set of all (functioning) programs is a subset of a countable set, but in itself not countable - which means that Cantor's diagonal argument does not work. So where are the "uncomputable numbers"?

There is even a thing called "Computable Analysis". Seems that I have to dig deeper into these concepts.

[ithruyou]

If all algebraic numbers can be expressed using radicals, why should we have a formual for the roots of any degree n polynomial? Can anybody prove it?

[secretsecret]

Golden ratio is root of x^2-x-1 why it is transcendental?