Permutations, Latin squares, number systems | Group theory episode 3

#abstractalgebra #grouptheory #numbersystem #permutations #sudoku

Groups are closely related to permutations, Latin squares, and sudoku puzzles. We explain where these permutations come from. We also look at the complex numbers and other number systems again, this time from the perspective of group theory. But first we spend a few moments looking at modular arithmetic.

I need to clarify something at 6:00. On the left, you see a general-purpose equation that works for any group. When talking abstractly about all possible groups, mathematicians use the word "multiplication", and they write it as a dot. So the word "multiplication" can stand for many things such as "concatenation", "composition", or, yes, even "addition". On the right, we have a specific group: addition modulo 4. So even though the group operation is *addition*, I also refer to it as *multiplication* at the same time. This may be confusing. But it pays to get used to this, because you will encounter it all the time when studying group theory. Thanks to one of our patrons for pointing this out.

Here are some interesting links:

A good, short introduction to group theory, and a connection to complex numbers. This is a good example of looking at the complex numbers through the lens of group theory.

A nice introduction to modular arithmetic, using a clock. Also talks about generators.

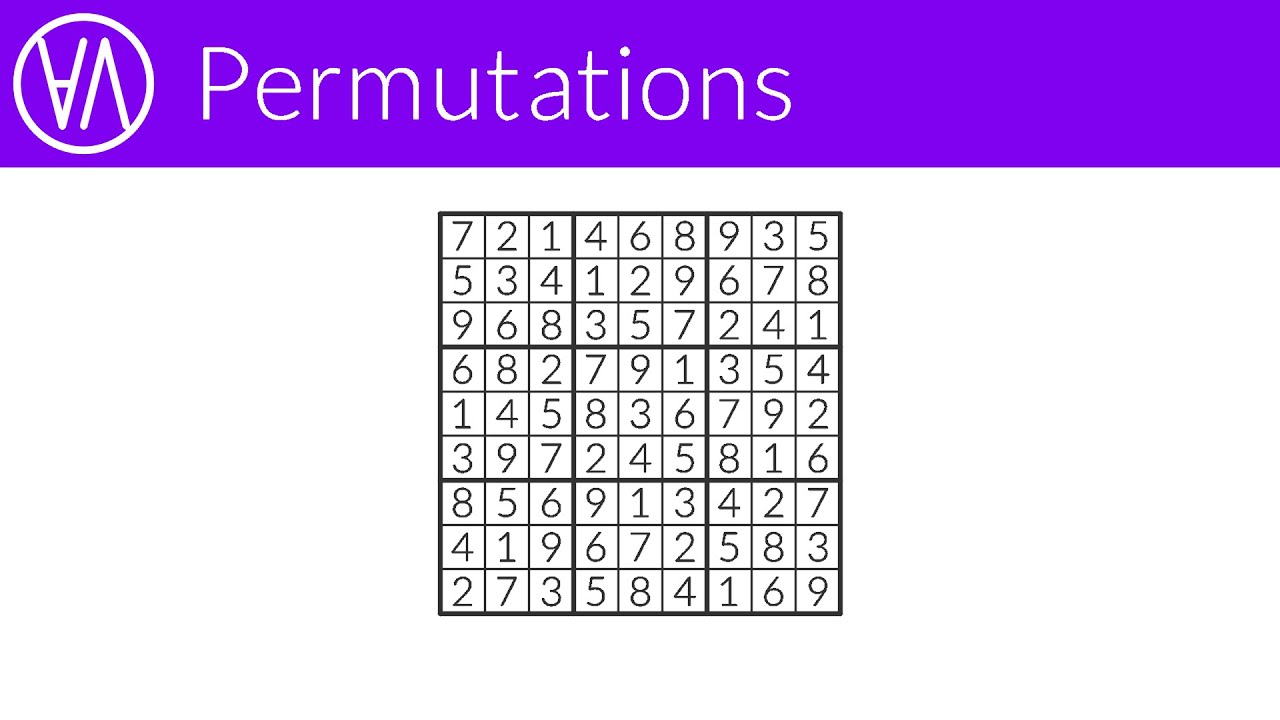
Here you can play the sudoku I showed you in the video. No cheating now: don't look at the solution before you try it yourself ;-)

0:00 Introduction
0:32 Modular addition
2:51 The addition table
4:51 Latin squares
7:26 Permutations
10:22 Number systems
12:34 Rational numbers
15:06 Complex numbers
16:15 The complex roots of unity

This video is published under a CC Attribution license

[StratosFair]

Nice stuff, looking forward to the following episodes of this series :)

[jaytea]

i really appreciate the dense summary about which groups exist under the different number systems, all in about 1 minute

[Laura-equv]

Given that there's an identity element within any group and since you've shown that there are no duplicate elements in any row; it follows that for any pair of group elements, none being an identity element, the operation taking this pair as its arguments may not return any of its input values. I don't think there's much use for this observation, but I'm happy to see that your series made me understand the group theory better, more intuitively, something I was aiming to achieve :)

[ohhhleonid]

Great video! Thanks for creating this.

[enpeacemusic]

I cannot describe the joy Latin Squares give me, they are such beautiful objects to study using group theory (which is pretty ironic lol)

[dan_nad]

Love this series, can't wait to see more about groups

[diribigal]

A nitpick, but the typesetting is a little distracting. "+>" has the plus halfway inside the angle bracket, letters for sets like C and Z and Q look odd as regular letters, and C0 and Q0 (without using a superscript or subscript) seem like they might be typos for ℂ\{0}, etc.

[alegian]

<Z, x> is not a group because there are no multiplicative inverses indeed. But the explanation that "1/5 is the inverse of 5, and 1/5 is not an integer" is not that helpful to me... What is 1/5? It belongs to another number system. There might be a nice definition of multiplication on Z that allows for inverses like that. I understand it would be beyond this video, but what would help me more is a proof like "5 has no multiplicative inverse in Z".

That being said, the logic behind the 1/5 reasoning (extending to Q and showing that Z is not enough) is probably correct and it sounds very interesting and raises many questions :D

[DeathSugar]

I wonder if there's some kind of test to verify whether permutation is a part of group or not, since theres fac(n) permutations in total and only few are within valid group.