Differential Equations: Direction Fields

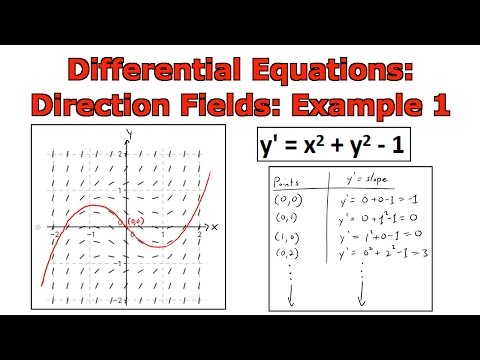
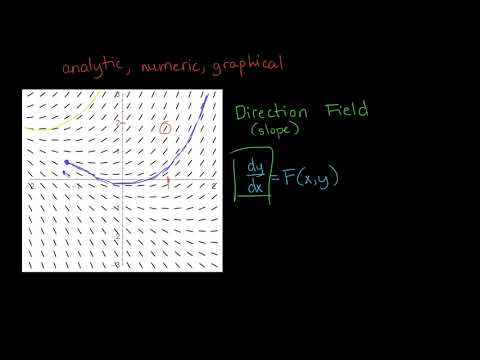
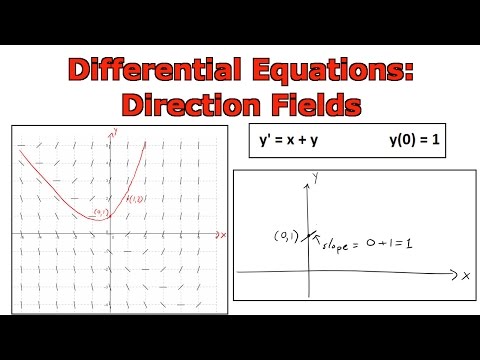
As explained in my earlier videos, most differential equations can't be solved explicitly which thus forces us to find different ways of estimating the solution; and one of those is in the concept of direction fields. For differential equations of the form y' = F(x, y), a direction field (or slope field) is any number of points in which the slope of the line segment near that point is plotted out. This allows us to get a general idea of the shape of the curve. Direction fields are very useful to visually see the solution of a differential equation without actually having to know the precise solution. This is a very important concept to understand so make sure to watch this video!

Related Videos:

------------------------------------------------------

[mes]

I don't always determine the solution of a differential equation but when I do I usually can't so I have to resort to direction fields ;)