filmov
tv
Differential Equations Direction Fields: Example 2: Electric Circuit
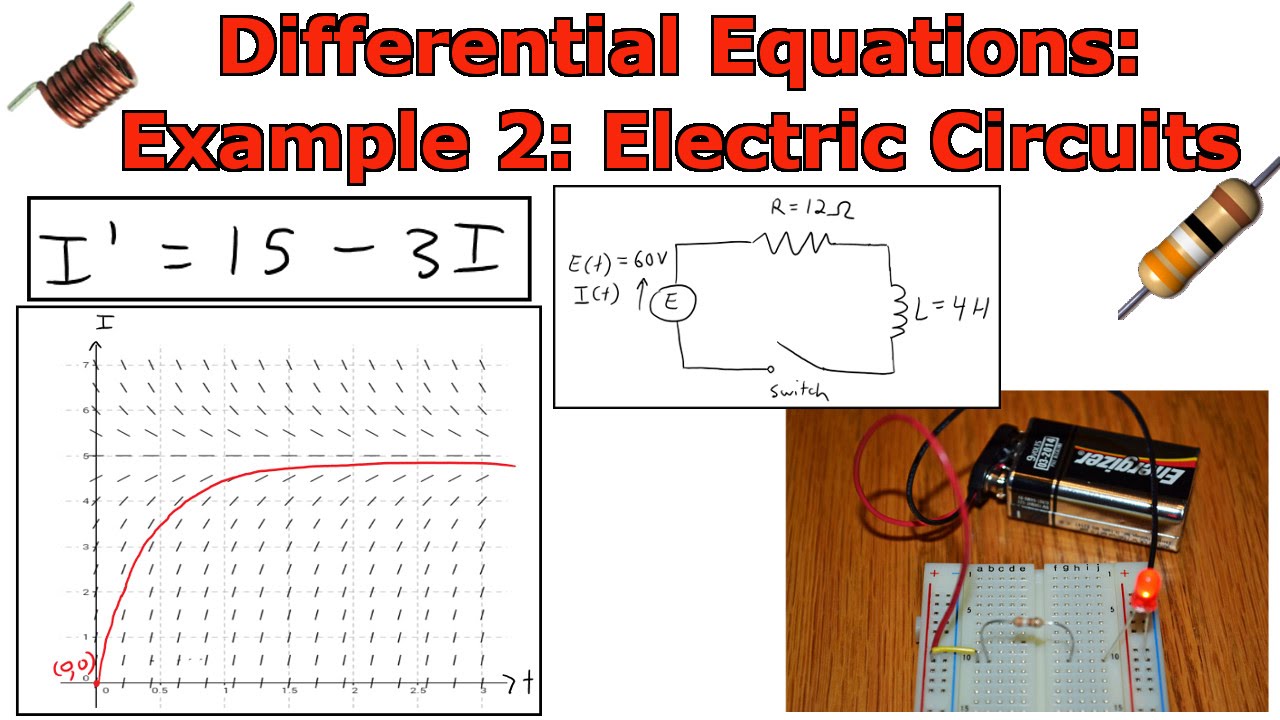
Показать описание
In this video I go over an example on drawing direction fields for the differential equation that I covered in my last video that relates the voltage drops due to an inductor and resistor with the voltage and current supplied of an electric circuit. The direction field in this example is very interesting in that, since the differential equation does not depend on the time variable but only on the current, this makes every line segment parallel to every other line segment to the left or the right of it, assuming they are at the same currents. This type of differential equation is called autonomous and thus if we know one solution to the differential equation, we can obtain infinitely many, simply by shifting the solution left or right.
This video relies heavily on the definitions and concepts involved in electric circuits so make sure to watch last video before watching this if you haven't covered electric circuits before.
Related Videos:
------------------------------------------------------
This video relies heavily on the definitions and concepts involved in electric circuits so make sure to watch last video before watching this if you haven't covered electric circuits before.
Related Videos:
------------------------------------------------------
Differential Equations: Direction Fields: Example 1
Slope Fields | Calculus
Differential equation from slope field | First order differential equations | Khan Academy
Sketching direction fields (KristaKingMath)
Drawing Slope Fields from Differential Equations - Calculus 2
Introduction to Slope Fields
Creating a slope field | First order differential equations | Khan Academy
The Geometric Meaning of Differential Equations // Slope Fields, Integral Curves & Isoclines
Slope field to visualize solutions | First order differential equations | Khan Academy
Introduction to Slope Fields (Differential Equations 9)
Direction Fields (Differential Equations)
Slope Field Example
Matching Slope Fields with Differential Equations
Direction Field Sketch Graph of f'=x+y Solution of Difference Equation
Differential Equation - Introduction (10 of 15) What Are Slope Fields and Solution Curves - 1?
Differential Equations: Quick and Easy Way to Create Direction Fields
Differential Equations: Direction Fields Introduction
Sketch the slope field of the differential equation
Direction Field Concept to Sketch Graph of Solution of Differential Equation
Differential Equations Direction Fields: Example 2: Electric Circuit
Differential Equations - 3 - EXAMPLE - Slope Field and Solution for y'=xy
Differential Equations - 2 - Slope Fields
MA451 1.3 Direction Fields
Isoclines: A Way to Analyze Slope Fields
Комментарии
 0:05:47
0:05:47
 0:21:12
0:21:12
 0:06:10
0:06:10
 0:08:37
0:08:37
 0:08:01
0:08:01
 0:09:35
0:09:35
 0:05:51
0:05:51
 0:09:52
0:09:52
 0:06:55
0:06:55
 0:34:18
0:34:18
 0:11:36
0:11:36
 0:01:15
0:01:15
 0:08:39
0:08:39
 0:07:07
0:07:07
 0:04:38
0:04:38
 0:13:58
0:13:58
 0:06:36
0:06:36
 0:04:00
0:04:00
 0:08:29
0:08:29
 0:14:46
0:14:46
 0:03:54
0:03:54
 0:11:15
0:11:15
 0:16:36
0:16:36
 0:08:14
0:08:14