Deriving the Black-Scholes Pricing Equation

preview_player
Показать описание
This video is part of my series on the Black-Scholes model.
Disclaimer: I do not know, why we use the normal pdf - shouldn't we use the lognormal pdf as the random variable is lognormal? If anybody knows, please leave me a comment.
  1. FinanceAndEconomics
Рекомендации по теме
Introduction to the 0:10:24

Introduction to the Black-Scholes formula | Finance & Capital Markets | Khan Academy

Deriving the Black-Scholes 0:10:41

Deriving the Black-Scholes Pricing Equation

The Easiest Way 0:09:53

The Easiest Way to Derive the Black-Scholes Model

Warren Buffett: Black-Scholes 0:15:54

Warren Buffett: Black-Scholes Formula Is Total Nonsense

Deriving the Black 0:13:34

Deriving the Black Scholes Call Formula

Black-Scholes PDE Derivation 0:04:30

Black-Scholes PDE Derivation in 4 minutes

Black Scholes Explained 0:14:03

Black Scholes Explained - A Mathematical Breakdown

Derivation of Black-Scholes 0:00:12

Derivation of Black-Scholes Equation |FULL|

19. Black-Scholes Formula, 0:49:52

19. Black-Scholes Formula, Risk-neutral Valuation

Black Scholes Formula 0:03:40

Black Scholes Formula explained simply

Measure change approach 0:05:44

Measure change approach to the derivation of Black Scholes

Deriving Black Scholes 0:17:24

Deriving Black Scholes

Deriving Black-Scholes Theorem 0:00:12

Deriving Black-Scholes Theorem on Live

Derivation of the 0:15:04

Derivation of the Black-Scholes equation

Black Scholes Derivation 0:16:55

Black Scholes Derivation

Charlie Munger on 0:00:39

Charlie Munger on Black–Scholes

Unlocking the Black-Scholes 0:00:59

Unlocking the Black-Scholes Model: Visualizing the Power of Options Trading in 60 Seconds

An intuitive explanation 0:05:48

An intuitive explanation the Black Scholes' formula

Black Scholes Option 0:09:23

Black Scholes Option Pricing Model Explained In Excel

Gamma of Black 0:08:33

Gamma of Black Scholes Price: Derivation and Intuitive Explanation

Mastering the Black-Scholes 0:00:59

Mastering the Black-Scholes Model: Essential Knowledge for Options Traders

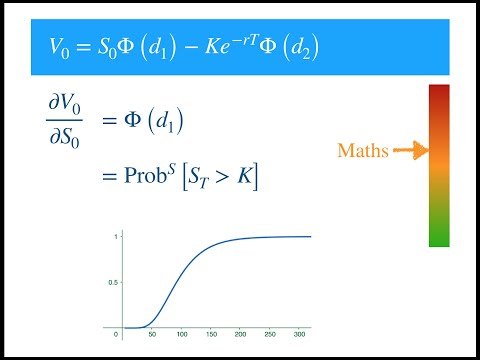
Delta of Black 0:11:38

Delta of Black Scholes Price: Derivation and Intuitive Explanation

Options Pricing Full 0:40:22

Options Pricing Full Derivation (European call and put using Black Scholes formula)

Derivation Of the 0:03:24

Derivation Of the Black-Scholes Equation (SDE)

Комментарии
Автор

Hi Could you make video about Monte Carlo option pricing?

michanieznanski
Автор

When you're calculation the Expected value by an integral that is f(x) * x where f(x) is your PDF of Normal Distribution and x is your payoff.
How come that if x = f(Ste^(mu-1/2sigma^2)(T-t) + sigma^2(SQRT(T-t)Y)) with f(x) i.e. the PDF you only look at the Y variable. You completely ignore mu, sigma, T-t, St.

You're basically saying when calculating f(x) i.e. the PDF that x is effectively just Y the normally distributed variable, completely ignore all other variables that come into the ST calculation

Nenormalnika
Автор

we know that the term inside f is lognormally distributed but you haven't specified any assumption on what f is, so I guess something is not right there! I mean how would we know pdf of f without knowing what function f is?

Appreciate your content overall though, it is very helpful. Just here I am not clear!

harbirkaur