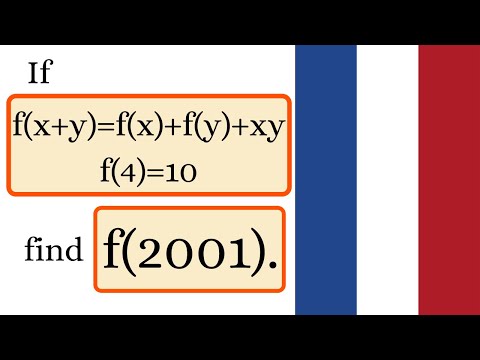
A Basic Functional Equation

In this video, I solved a functional equation by algebraic manipulation. By observation, it was possible to rewrite the output in terms of the input. The constraint given appeared to be irrelevant since my first strategy did not use a dummy variable.

[sjmousavi]

Thanks. After 30 years I'm beginning to understand the functional equations.

[JASONKINGMATHK]

Great. Math teacher. You are desereved

[e.d.]

Just to clarify a point:
The reason that when finding the function f(ꭕ) we demand |ꭕ|≥2:, is:
If we look at the function x+1/x and investigate it properties we will see that its range is -ꝏ≤(x+1/x)≤-2 and 2≤(x+1/x)≤+ꝏ.
Thus, the functional equation in the problem *does not by itself gives us any information* about the properties of the function for -2<ꭕ<2.
If, for example, in addition to the functional equation we were told that f(ꭕ) is continuous and differential n times at any point (for any positive integer n), than we could have concluded the the solution f(ꭕ)=(ꭕ^2)-2 is valid in the domain -2<ꭕ<2 *as well*.

[beastrule]

Hi, I am a huge fan of your channel. Here is a question I really liked:
Let Q+ be the set of positive rational numbers. Construct a function f : Q+ → Q+ such that f(xf(y)) = f(x) y for all x, y in Q+.
Hope it helps!

[epd]

Love your videos! You are a genius and a great teacher! Plus, you have perfect penmanship!

[hookahsupplier.]

Spectacular problem! So much fun tacit and palpable beauty in the question.❤

[skwbusaidi]

If the condition is only x>2, we can use AM-GM inwuality
(x+1/x)/2>= sqrt(x.1/x)
x+1/x >=2
Since x+1/x is the orignal input, when we change it to x, it shiuold have the same restrictions

[WahranRai]

Let x + 1/x = t ---> (x+1/x)^2 = t^2 ---> x^2 + 1/x^2 + 2 = t^2 --->
x^2 + 1/x^2 = t^2 - 2 ---> f(t) = t^2 - 2 ---> f(x) = x^2 -2 without condition on x

[spoon_s]

my solution:
1. find the inverse of g(x)=x+1/x by multiplying both sides by x and use the quadratic formula
2. let x -> g⁻¹(x) in x² + 1/x², and after some simplifications, the result is x²-2

very fast and convenient

[juliovasquezdiaz]

Excelente vídeo. Gracias por compartir. Saludos desde Chiclayo Norte del Perú.

[giorgiobarchiesi]

Great video, thanks. Regarding the final statement that the solution is valid for any value of x, I would exclude 0.

[himanshuhani]

You can also try it with a way like consider y=f(x+1/x) and y=x²+1/x²
Now do partial differentiation with respect to either x or y and then use given condition |x|≥2

[patk]

Quite clever, we don't see a lot of these type of math problems in text books...
I get to understand: that the aim is to formed (x^2 + 1/x^2) into (x + 1/x)

[kykvjes]

You need t>=2, not x>=2
If t>=2 then x+1/x >=2
We assume x>0 and multiply both terms by x
So x^2 -2x +1 >=0
But x^2-2x+1 is (x-1)^2, a perfet square that allways is greather than 0
If x<0 we have x^2-2x+1<=0 then (x-1)^2 <=0. A perfet square can't be less than 0. So x can't be less than 0
x can't be 0 because we have 1/x

I think the restriction is x>0

[Antagon]

Btw... You can also get the answer just by dividing the result by the input... After that you can be 100% sure the result is equal to the input* factor
The factor in this case is (x4 + 1) / (x3 + x)
The function itself is then just equal to:
f(x) = factor * x

[archananethi]

I think another way we could solve this is
Let T = (x+1/x)
T^2 -2 = x^2 + 1/x^2
So f(t) = t^2 - 2
Hence f(x) = x^2 - 2

[dougaugustine]

Another video I had to watch twice to begin to understand.

[Hobbitangle]

f(x) = x²-2
It's almost obvious if we note that
(x+1/x)²=x²+1/x²+2

[compiling]

So what's going on is that the range of x + 1/x for real x is R excluding (-2, 2). So f(x) is fully defined for all x in the given range, but the definition doesn't cover what happens when |x| < 2 (although it probably follows x^2-2).

[justcommenting]

I did both at the same time
I did call x+1/x as t, but then I squared both sides and the development was the same as the 1st one so that f(t)=t²-2, therefore, f(x)=x²-2