filmov
tv
A cubic system of equations

Показать описание
This video is about a cubic system of equations
If you need to post a picture of your solution or idea:
#ChallengingMathProblems #PolynomialSystems
LINKS:
EXPLORE:
If you need to post a picture of your solution or idea:
#ChallengingMathProblems #PolynomialSystems
LINKS:
EXPLORE:
A Cubic System of Equations
A cubic system of equations
Solving a Cubic System
Germany Math Olympiad, a system of cubic equations
Solving a cubic system
Solving a cubic system for real solutions
Solving a Cubic System for Real Solutions
Solving a cubic equation
A nice cubic equation | Solve for x | Math Olympiad
Solving a Nice Cubic System in Two Ways
A Cubic Polynomial System
Solving a Cubic Polynomial in Two Ways
Solve the Nonlinear System: A Cubic Polynomial and a Line
Solving a cubic system of equation
Let's Solve A Cool Cubic System
Solving a Cubic System in a Non-polynomial Way
The Linear-Cubic System. THE MOST GENERAL SOLUTION. | System of Equations
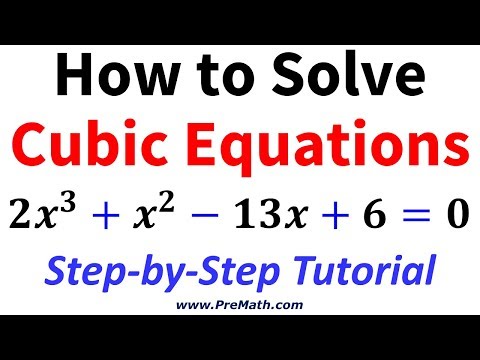
How to Solve Advanced Cubic Equations: Step-by-Step Tutorial
Solving A Special Cubic System in Two Ways
Solving A Cubic Equation With An Irrational Root
Solving a Cubic Equation - √(X^3) = 1
German Math Olympid | How to solve this cubic system of equations | Simple and easy solution
A Nice Cubic Equation - Number Theory | Advanced Math
Can You Solve A Cubic System? #algebraequations #polynomials
Комментарии
 0:13:25
0:13:25
 0:13:25
0:13:25
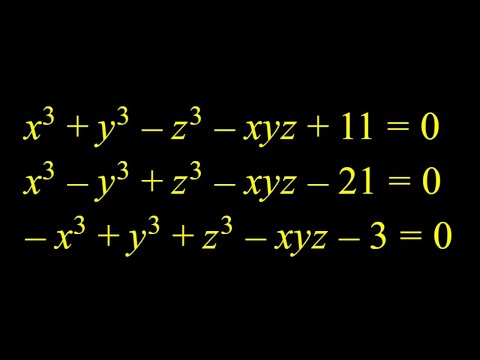 0:14:14
0:14:14
 0:11:36
0:11:36
 0:14:14
0:14:14
 0:09:15
0:09:15
 0:09:15
0:09:15
 0:07:26
0:07:26
 0:11:09
0:11:09
 0:09:13
0:09:13
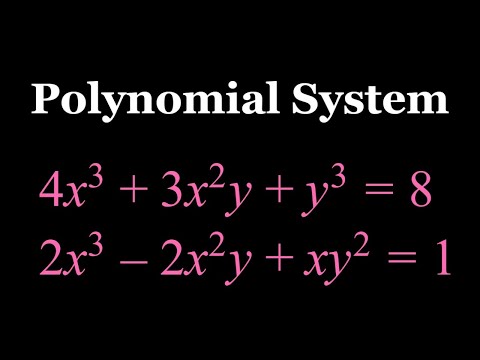 0:10:31
0:10:31
 0:09:55
0:09:55
 0:08:17
0:08:17
 0:10:10
0:10:10
 0:09:13
0:09:13
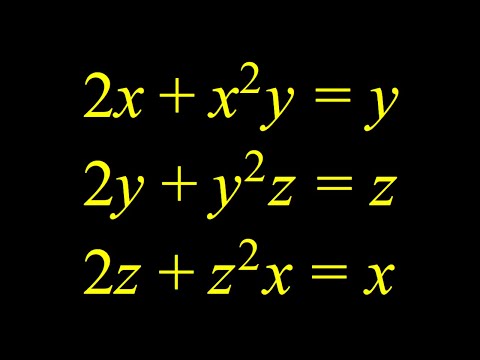 0:09:29
0:09:29
 0:08:54
0:08:54
 0:10:52
0:10:52
 0:14:48
0:14:48
 0:16:23
0:16:23
 0:00:58
0:00:58
 0:05:33
0:05:33
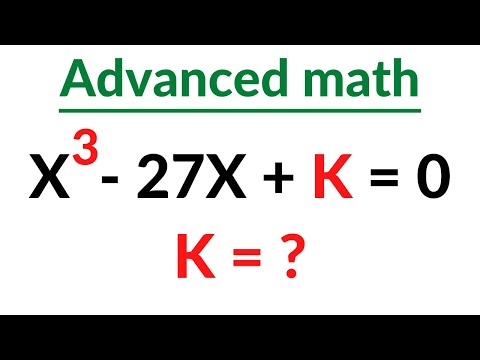 0:08:36
0:08:36
 0:00:29
0:00:29