filmov
tv
Solving A Cubic Equation With An Irrational Root

Показать описание
🤩 Hello everyone, I'm very excited to bring you a new channel (SyberMath Shorts).
Enjoy...and thank you for your support!!! 🧡🥰🎉🥳🧡
If you need to post a picture of your solution or idea:
#ChallengingMathProblems #Polynomials
via @YouTube @Apple @Desmos @NotabilityApp @googledocs @canva
PLAYLISTS 🎵 :
Enjoy...and thank you for your support!!! 🧡🥰🎉🥳🧡
If you need to post a picture of your solution or idea:
#ChallengingMathProblems #Polynomials
via @YouTube @Apple @Desmos @NotabilityApp @googledocs @canva
PLAYLISTS 🎵 :
Simple way to solve a cubic equation
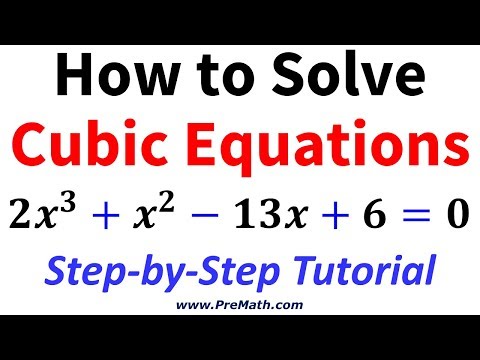
How to Solve Advanced Cubic Equations: Step-by-Step Tutorial
Solve any Cubic Equations | Cardano's Method |
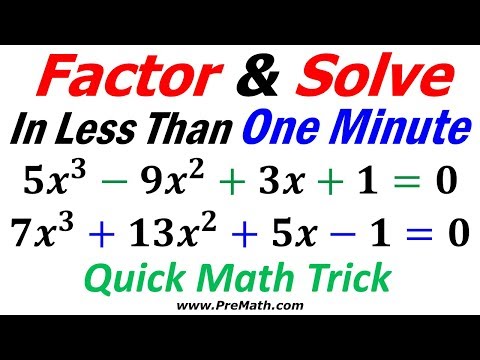
Factor and Solve Cubic Equations in Less Than One Minute! - Super Simple Trick
Solving a Cubic equation Using an Algebraic Trick
Solving Cubic Equations (factoring)
500 years of NOT teaching THE CUBIC FORMULA. What is it they think you can't handle?
solving a cubic equation by completing the cube
Algebraic Identities - Concepts & Examples (Part 1) Polynomials | Class 9 Maths Chapter 2 #cbse
CUBIC EQUATION FACTORIZATION SHORTCUT/ SOLVING CUBIC EQUATIONS IN 10 SECONDS/ Math Tricks.
How To ACTUALLY Solve a Cubic Equation using Trigonometry
Solving a simple cubic equation. A trick you should know!
How to Solve Advanced Cubic Equations: Easy-to-Understand Explanation
Solving Simple Cubic Equations
Factorise Cubic Equation Grade 12: Introduction
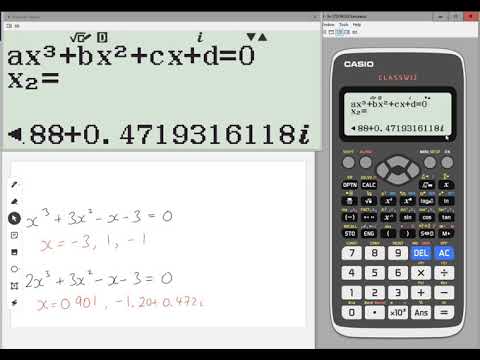
Classwiz How-To: Solving a Cubic Equation
Solve Cubic Equations - Total Sum Method
The Cubic Formula: Roots of the General Cubic Equation
How To Solve Cubic Equations
so you want to see the cubic formula
Solve Cubic Equations - Synthetic Division Method
Factorising cubic functions: The kx method
Find Factors and Solve Cubic Equations in Less Than ONE Minute! - Leading Coefficient Is Not One
So you want to solve a cubic equation?
Комментарии
 0:04:56
0:04:56
 0:10:52
0:10:52
 0:04:20
0:04:20
 0:07:18
0:07:18
 0:11:45
0:11:45
 0:03:32
0:03:32
 0:36:58
0:36:58
 0:07:28
0:07:28
 0:50:06
0:50:06
 0:12:06
0:12:06
 0:14:58
0:14:58
 0:06:41
0:06:41
 0:11:25
0:11:25
 0:09:13
0:09:13
 0:06:17
0:06:17
 0:03:14
0:03:14
 0:09:51
0:09:51
 0:04:50
0:04:50
 0:09:29
0:09:29
 0:40:16
0:40:16
 0:09:30
0:09:30
 0:10:22
0:10:22
 0:09:10
0:09:10
 0:02:04
0:02:04