filmov
tv
Solving a Nice Cubic System in Two Ways
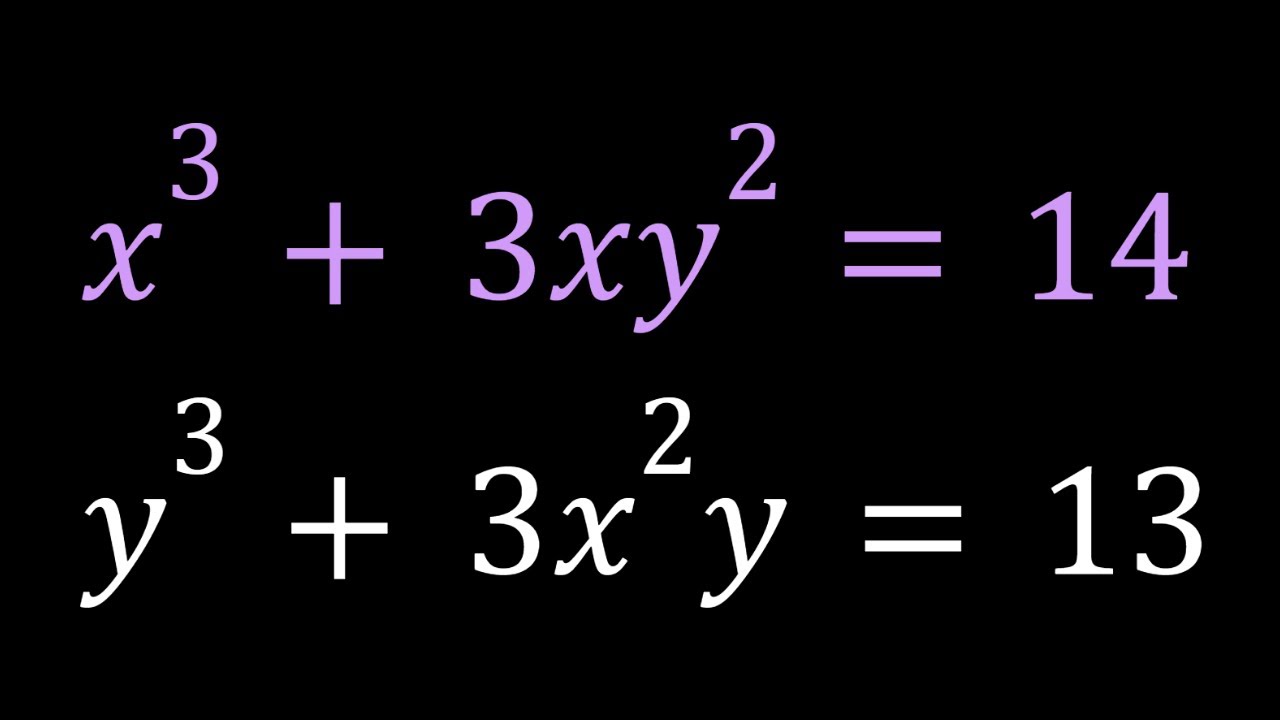
Показать описание
If you need to post a picture of your solution or idea:
#ChallengingMathProblems #PolynomialSystems
PLAYLISTS 🎵 :
#ChallengingMathProblems #PolynomialSystems
PLAYLISTS 🎵 :
Solving a Nice Cubic System in Two Ways
Solving A Nice Cubic System in Two Ways
Solving A Nice Cubic Equation | #Factoring #Algebra
Solving a cubic system
Solving A Nice Cubic Equation | Math Olympiads
A Cubic System Solved in Two Ways
A Nice Polynomial Equation | Cubic Formula
A Nice Cubic | x^3-3x=2
Square, Square Root Cube, Cube root Exercise 4.3 Question No.1 a- e class 8 KPK Math
Solving A Special Cubic System in Two Ways
new magic trick repeat 10 times solve rubik's cube #shortc #viral #respect #rubik
Solving a cubic system for real solutions
A Nice Cubic Equation | Cubic Formula | #PolynomialEquations
solving a cubic equation by completing the cube
This trick can make your rubik's cube 2x faster😱🔥#ytshorts#shorts#drcuber
Solving a Cubic System in a Non-polynomial Way
A Cubic Functional Equation With Nice Results 😄
Octopus solves rubiks cube
Hard Rubik’s Cube Solving
Can You Factor and Solve This Cubic Equation In Under a Minute? - Simple Math Trick
Using maths to solve the Rubik's Cube
The new Rubik’s cube Company!! MoreTry 🌟
A cubic system of equations
A Beautiful Cubic Diophantine Equation
Комментарии
 0:09:13
0:09:13
 0:09:41
0:09:41
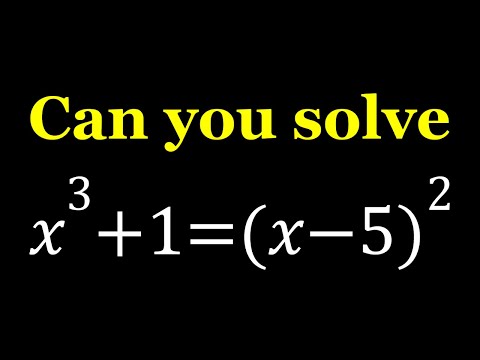 0:09:20
0:09:20
 0:14:14
0:14:14
 0:00:37
0:00:37
 0:08:41
0:08:41
 0:10:56
0:10:56
 0:08:50
0:08:50
 0:11:33
0:11:33
 0:14:48
0:14:48
 0:00:29
0:00:29
 0:09:15
0:09:15
 0:09:38
0:09:38
 0:07:28
0:07:28
 0:00:16
0:00:16
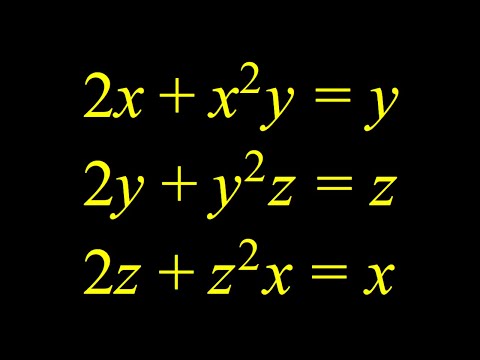 0:09:29
0:09:29
 0:09:31
0:09:31
 0:00:19
0:00:19
 0:00:14
0:00:14
 0:08:46
0:08:46
 0:20:59
0:20:59
 0:00:51
0:00:51
 0:13:25
0:13:25
 0:10:20
0:10:20