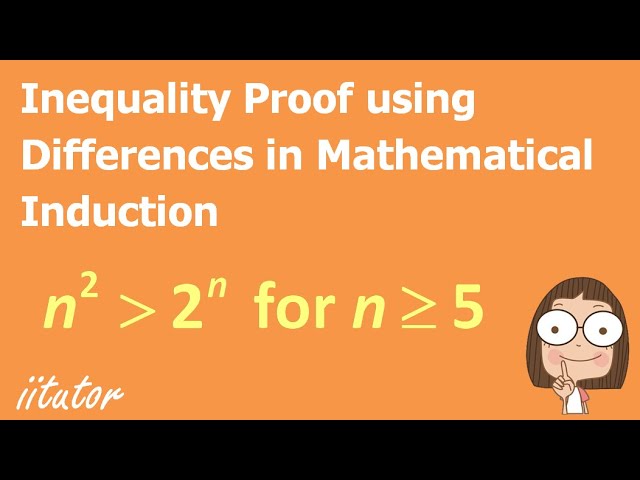
💯 The Inequality Proof using the Difference by Mathematical Induction. Watch this video to find out!

📌 Receive Comprehensive Mathematics Practice Papers Weekly for FREE

Proving an inequality using difference by mathematical induction involves the following steps:

1. Basis Step: Prove the statement is true for the first value of n, typically n = 1.
2. Inductive Step: Assuming the statement is true for n=k, prove that it is true for n=k+1.
3. Conclusion: Since the statement is true for n=1 and can be shown to be true for n=k+1 whenever it is true for n=k, it follows that the statement is true for all positive integers n.

In this method, you first assume that the inequality holds true for some value of n, and then you use that assumption to show that the inequality also holds true for n+1. Repeat this process until you prove the inequality for all positive integers.

The key idea is to express the difference between the two sides of the inequality for n+1 in terms of the difference for n and then show that the difference for n+1 is always positive or zero.