Visual Cauchy-Schwarz Inequality

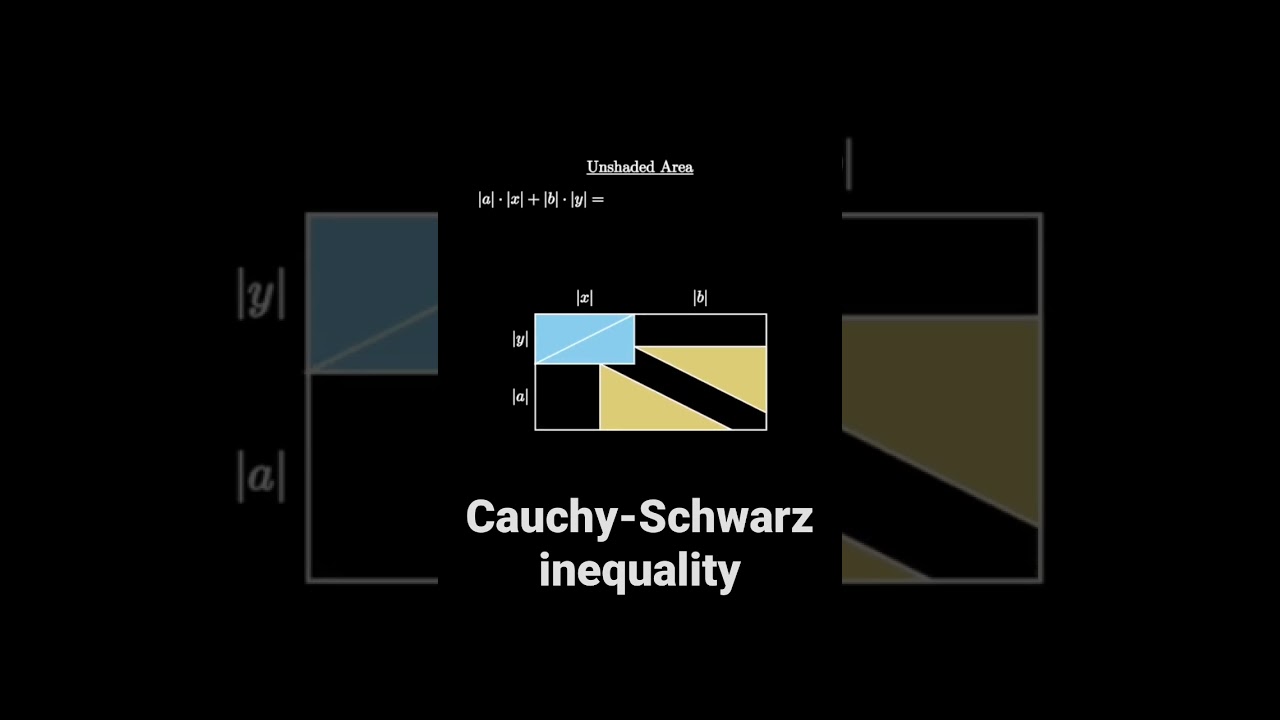
This is a short, animated visual proof of the two-dimensional Cauchy-Schwarz inequality (sometimes called Cauchy–Bunyakovsky–Schwarz inequality) using the Side-angle-side formula for the area of a parallelogram.

To learn more about animating with manim, check out:

#math​ #inequality ​ #manim​ #animation​ #theorem​ #pww​ #proofwithoutwords​ #visualproof​ #proof​ #iteachmath #algebra #areas #mathematics #cauchyschwarz #algebraicidentity #mathshorts​ #mathvideo​ #mtbos

[ruthvikas]

I love the graphical representation of maths. It just feels different and more clear.

[realcontentgamer]

This man is literally better than my school teachers

[oliverpackham]

I always loved dot and cross product equalities. Michael Penn has a linear algebra playlist will all sorts of cool ones. The vector dotted with itself = it's magnitude^2 is a fun and easy one you could do a short on.

[Tiago-]

I didn't get any of this, but the shapes were pretty

[asparkdeity]

This is so beautiful! Every linear algebra proof of Cauchy-Schwarz that I have come across is so messy and always involves the determinant of some quadratic, but u couldn’t have explained this in any simpler way, using nothing more than simple trigonometry. Thank you so much

[Zangoose_]

This is what I needed back in college. Thanks, man

[abdullahyasinyagmur]

This is just amazing. It's always nice to see the intuition geometrically

[SeanSkyhawk]

When will you be posting the Pythagoreran Theorem proof as recently published by Jackson and Johnson?

[matthewbell]

My main takeaway was how shapes can be really pretty

[alvargd]

when I saw Cauchy in the title got scared 😅 but it wasnt nearly as bad as I expected

[TheCyanKiller]

The number of times the word absolute was said was more than the number of times a word that was not absolute was said

[StratosFair]

Ok, I have to subscribe to your channel after this

[flamurtarinegjakyt]

I needed this for calculus II, thank you

[viking]

Lovely just lovely I wish I had you in my school

[Joffrerap]

prerequisite:
- pythagorean theorem
- area of a parallelogram

- triangle inequality and absolute value property
ok not as much as i thought.

[mathematicsman]

You are doing great like mind your descision

[Rócherz]

Now animate the 3D inequality *with cubes.*

(It’s the meme of “now draw her stealling the Chaos Emeralds”, I’m neither actually asking nor demanding it.)

[jupjup]

You can also prove this using the dot product formula:
abs(a•b=|a||b|cosθ)
Since |cosθ| is less than or equal to 1 this also proves the inequality. However the proof for the dot product formula is more complicated than the one on the video.

[arvindsrinivasan]

I feel like this is better visualized with the vectors and using projections defined using dot products

[srikrishnaghosh]

I have become fan of yours. Assuming this particular equation will be dealt with only after having the idea of dot product, it is quite straight forward that,

||(a, b)•(x, y)||=||(a, b)||•||(x, y)|||cos(z)|
<= ||(a, b)||•||(x, y)||, since |cos(z)|<=1.

It would be very nice if you kindly put such video on dot product itself. As a physics teacher i try to give a pictorial representation of the dot product but i am sure your way of presenting the same would be unique and very intriguing. I would also request you to provide a demo on how you make such beautiful animations with figures!!!