filmov
tv
Solving For A Nice Function
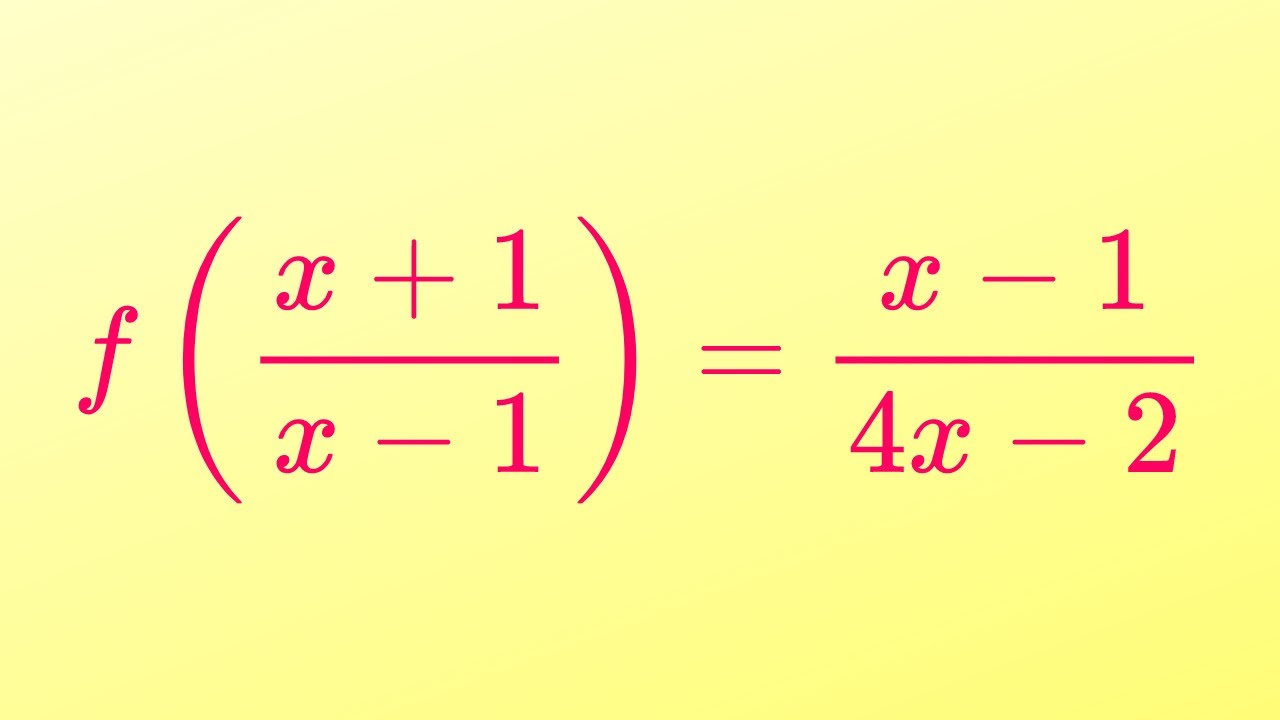
Показать описание
🤩 Hello everyone, I'm very excited to bring you a new channel (aplusbi)
Enjoy...and thank you for your support!!! 🧡🥰🎉🥳🧡
When you purchase something from here, I will make a small percentage of commission that helps me continue making videos for you.
If you are preparing for Math Competitions and Math Olympiads, then this is the page for you!
CHECK IT OUT!!! ❤️ ❤️ ❤️
If you need to post a picture of your solution or idea:
#radicals #radicalequations #algebra #calculus #differentialequations #polynomials #prealgebra #polynomialequations #numbertheory #diophantineequations #comparingnumbers #trigonometry #trigonometricequations #complexnumbers #math #mathcompetition #olympiad #matholympiad #mathematics #sybermath #aplusbi #shortsofsyber #iit #iitjee #iitjeepreparation #iitjeemaths #exponentialequations #exponents #exponential #exponent #systemsofequations #systems
#functionalequations #functions #function #maths #counting #sequencesandseries
#algebra #numbertheory #geometry #calculus #counting #mathcontests #mathcompetitions
via @YouTube @Apple @Desmos @NotabilityApp @googledocs @canva
PLAYLISTS 🎵 :
Enjoy...and thank you for your support!!! 🧡🥰🎉🥳🧡
When you purchase something from here, I will make a small percentage of commission that helps me continue making videos for you.
If you are preparing for Math Competitions and Math Olympiads, then this is the page for you!
CHECK IT OUT!!! ❤️ ❤️ ❤️
If you need to post a picture of your solution or idea:
#radicals #radicalequations #algebra #calculus #differentialequations #polynomials #prealgebra #polynomialequations #numbertheory #diophantineequations #comparingnumbers #trigonometry #trigonometricequations #complexnumbers #math #mathcompetition #olympiad #matholympiad #mathematics #sybermath #aplusbi #shortsofsyber #iit #iitjee #iitjeepreparation #iitjeemaths #exponentialequations #exponents #exponential #exponent #systemsofequations #systems
#functionalequations #functions #function #maths #counting #sequencesandseries
#algebra #numbertheory #geometry #calculus #counting #mathcontests #mathcompetitions
via @YouTube @Apple @Desmos @NotabilityApp @googledocs @canva
PLAYLISTS 🎵 :
Solving For A Nice Function
A nice Math Olympiad Logarithmic Equation | Lambert W function | Can you solve logx+64^logx=1/3 ?
Solving a Nice Functional Equation
USA Nice Olympiad Exponential Equation: solve for a!
A Nice and Easy Functional Equation
Solving a Nice Functional Problem from Russia | Math Olympiad Problem | Simple Functional Question
Solving a Nice Functional Equation
Problem-Solving for Developers - A Beginner's Guide
SOLVE FOR X, A VERY NICE EXPONENTIAL EQUATION
Solving A Nice Exponential Equation
A Nice Equation To Solve | A Nice Algebra problem | Can you solve?
Solving A Nice Differential Equation
A nice sum from France solved in 3 ways!
Solving a Nice Polynomial System in Two Ways
Solving a Nice Exponential Equation in Two Ways
Solving Exponential Equation
Norway Math Olympiad Question | You should be able to solve this!
How To Solve Exponential Equation x^5=9^x || Solving Exponential Equations.
Solving a Nice Cubic System in Two Ways
How To Solve Math Percentage Word Problem?
[ASMR] Solving a Maths Problem #Shorts
Solving a Beautiful Equation!
Math Olympiad Question | Equation solving | You should learn this trick to pass the exam
Solving 3^x+4^x=5^x
Комментарии
 0:08:39
0:08:39
 0:14:49
0:14:49
 0:06:58
0:06:58
 0:08:05
0:08:05
 0:08:14
0:08:14
 0:02:46
0:02:46
 0:06:28
0:06:28
 0:10:44
0:10:44
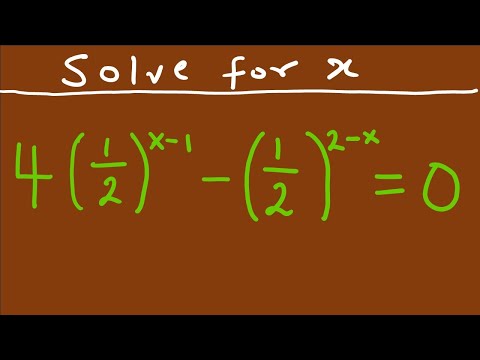 0:02:52
0:02:52
 0:06:30
0:06:30
 0:03:23
0:03:23
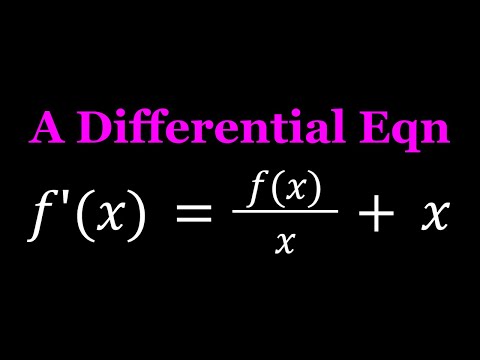 0:09:28
0:09:28
 0:09:04
0:09:04
 0:09:27
0:09:27
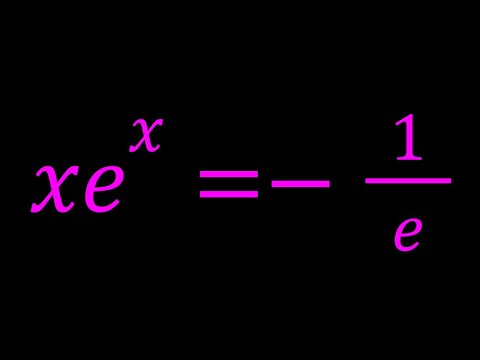 0:11:14
0:11:14
 0:00:56
0:00:56
 0:03:21
0:03:21
 0:11:56
0:11:56
 0:09:13
0:09:13
 0:00:29
0:00:29
![[ASMR] Solving a](https://i.ytimg.com/vi/TAqYga-ABkI/hqdefault.jpg) 0:00:53
0:00:53
 0:00:25
0:00:25
 0:01:54
0:01:54
 0:00:31
0:00:31