filmov
tv
Solving A Nice Exponential Equation
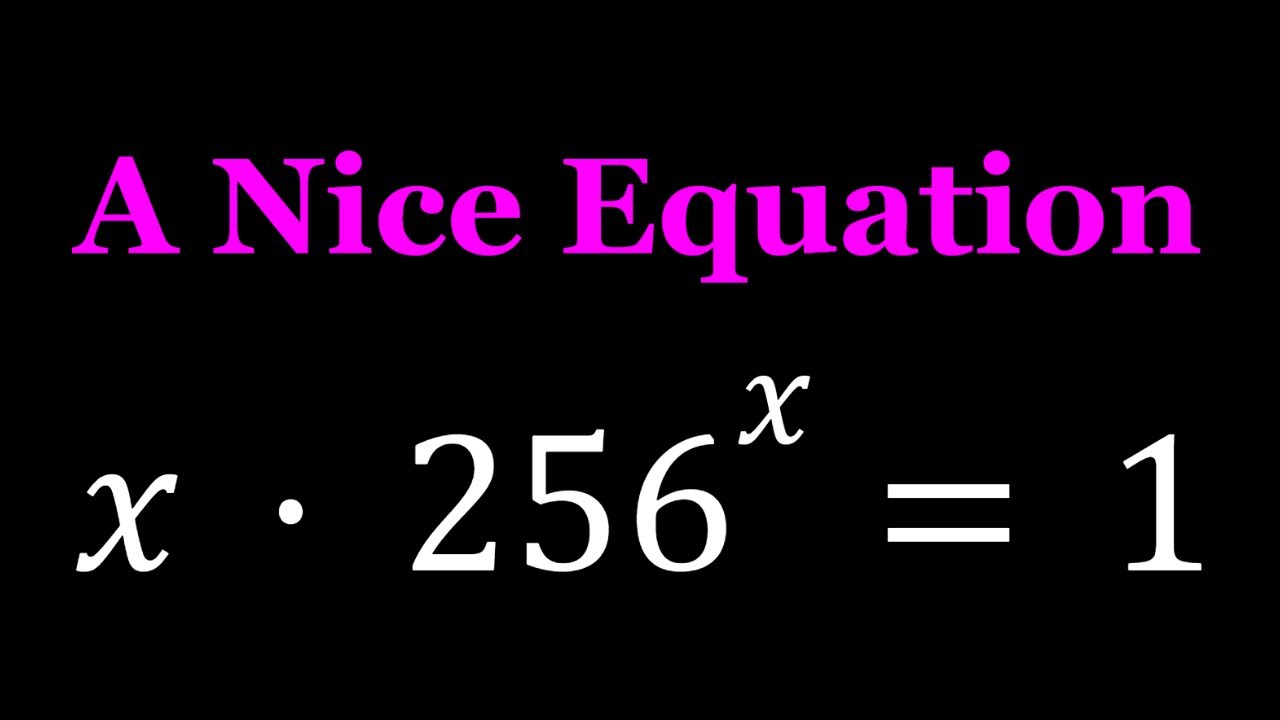
Показать описание
🤩 Hello everyone, I'm very excited to bring you a new channel (SyberMath Shorts)
Enjoy...and thank you for your support!!! 🧡🥰🎉🥳🧡
If you need to post a picture of your solution or idea:
#ChallengingMathProblems #ExponentialEquations #Exponentials
via @YouTube @Apple @Desmos @NotabilityApp @googledocs @canva
PLAYLISTS 🎵 :
Enjoy...and thank you for your support!!! 🧡🥰🎉🥳🧡
If you need to post a picture of your solution or idea:
#ChallengingMathProblems #ExponentialEquations #Exponentials
via @YouTube @Apple @Desmos @NotabilityApp @googledocs @canva
PLAYLISTS 🎵 :
Solving A Nice Exponential Equation
Solving Exponential Equations
Solving A Nice Exponential Equation | Real and Complex
United Kingdom - A Nice Exponential Equation | Math olympiad Question
A Nice Exponential Equation (5^x-3^x=16)
A Nice Math Olympiad Exponential Equation 3^x = X^9
A Nice and Interesting Exponential Equation
A Very Nice Exponential Equation | Math Olympiads
।। maths olympiad ।। A nice exponential equation ।। find the value of x @mathscuriosity494...
A Nice Exponential Equation
Solving A Nice Exponential Equation
A nice exponential equation.
Solving a Nice Exponential Equation in Two Ways
A Nice Exponential Equation
A Nice Exponential Equation, x²=2ˣ
A Nice Exponential Equation | Math Olympiads
Solving Exponential Equation
A Nice Exponential Equation
Imagine Solving A Nice Exponential Equation!
A Very Nice Exponential Equation | Can you solve?
Solving an exponential equation with different bases
Solving an exponential equation
A Nice Homemade Exponential Equation
Solving A Nice Exponential Equation | x^x^5=100
Комментарии
 0:06:30
0:06:30
 0:16:36
0:16:36
 0:10:28
0:10:28
 0:09:19
0:09:19
 0:06:37
0:06:37
 0:02:34
0:02:34
 0:09:46
0:09:46
 0:09:07
0:09:07
 0:07:26
0:07:26
 0:08:50
0:08:50
 0:09:56
0:09:56
 0:00:55
0:00:55
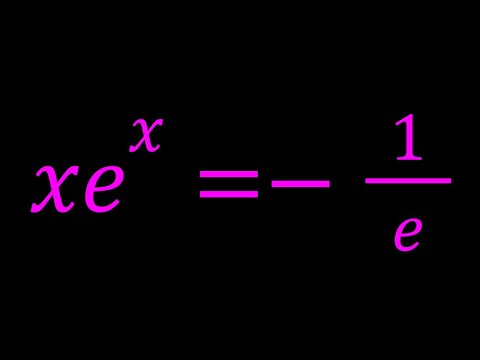 0:11:14
0:11:14
 0:00:48
0:00:48
 0:10:34
0:10:34
 0:06:50
0:06:50
 0:00:56
0:00:56
 0:09:32
0:09:32
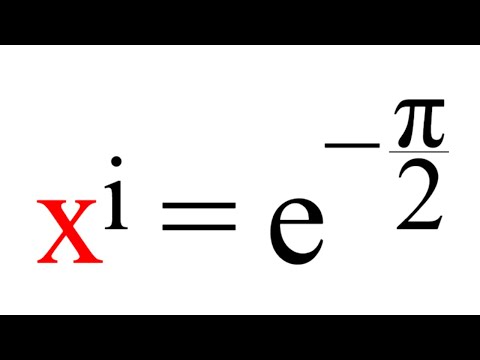 0:04:52
0:04:52
 0:09:21
0:09:21
 0:03:01
0:03:01
 0:03:14
0:03:14
 0:05:31
0:05:31
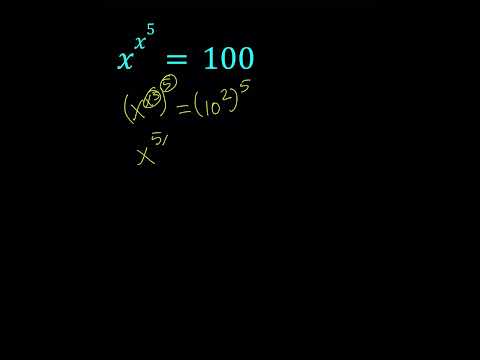 0:00:48
0:00:48