filmov
tv
Prove (dy/dx) = (d/dx)(√u) = (du/dx)/(2√u), if y = √u

Показать описание
The derivative of square root of u. Prove (dy/dx) = (d/dx)(√u) = (du/dx)/(2√u), or the derivative of y with respect to x is equal to the derivative of square root of u with respect to x is equal to the derivative of u with respect to x divided by 2 times the square root of u, if y = √u. √u = u^(1/2). Hence, y = √u = u^(1/2) = u^n, where n = 1/2. To prove this, we will employ the chain rule, and the power formula.
Mharthy's Channel's Playlists:
Mharthy's Channel's Playlists:
 0:08:48
0:08:48
 0:06:23
0:06:23
 0:09:17
0:09:17
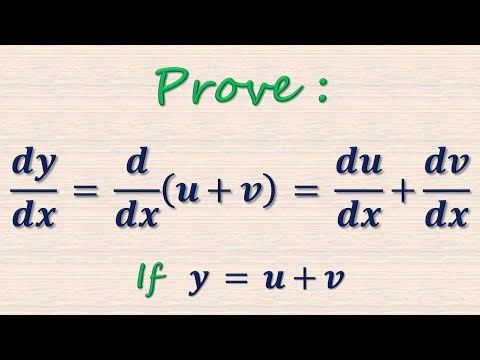 0:07:29
0:07:29
 0:09:25
0:09:25
 0:06:01
0:06:01
 0:01:44
0:01:44
 0:09:26
0:09:26
 0:05:52
0:05:52
 0:05:31
0:05:31
 0:05:39
0:05:39
 0:00:35
0:00:35
 0:02:14
0:02:14
 0:03:58
0:03:58
 6:38:13
6:38:13
 0:12:13
0:12:13
 0:10:58
0:10:58
 0:04:54
0:04:54
 0:05:15
0:05:15
 0:03:11
0:03:11
 0:30:06
0:30:06
 0:01:29
0:01:29
 0:00:50
0:00:50
 0:18:08
0:18:08