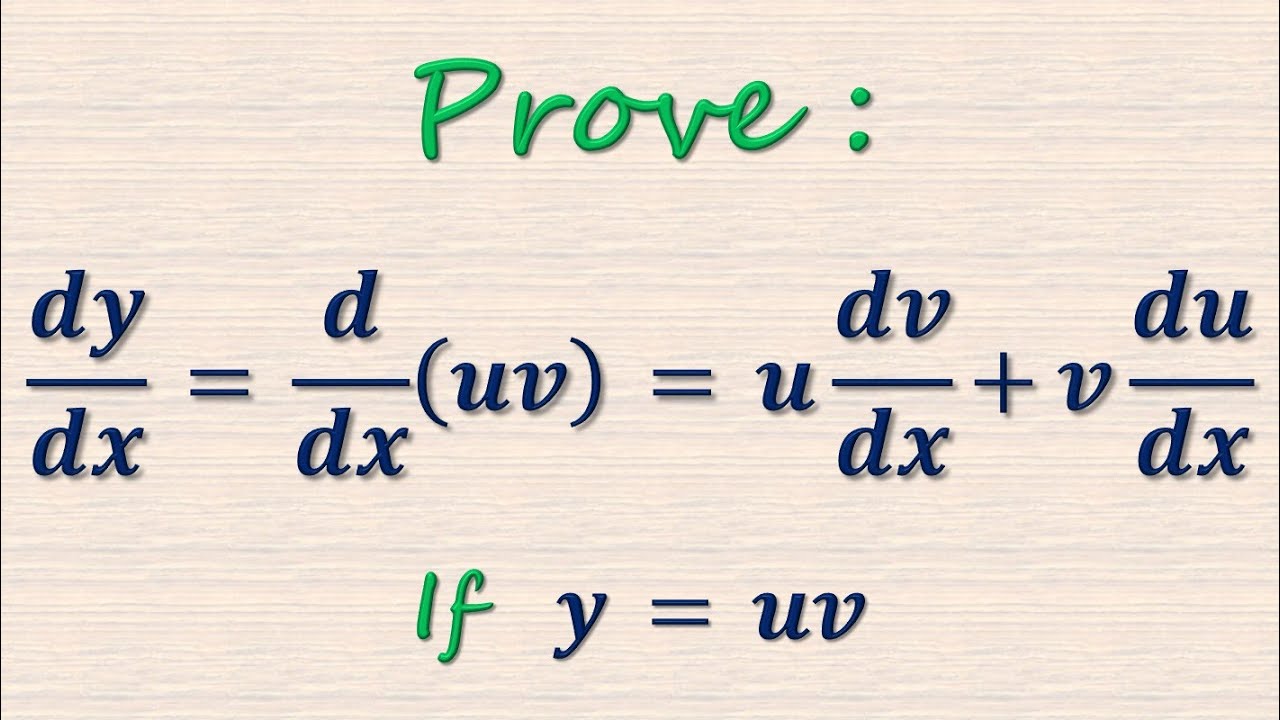
Prove (dy/dx) = (d/dx)(uv) = u(dv/dx) + v(du/dx), if y = uv

preview_player
Показать описание
The derivative of the product of two functions formula. Prove (dy/dx) = (d/dx)(uv) = u(dv/dx) + v(du/dx), if y = uv, or the derivative of y with respect to x of the product of two functions is equal to u times the derivative of v with respect to x plus v times the derivative of u with respect to x, if y = uv.

Proving this formula by means of the method of differentiation involving limits and increments.

Mharthy's Channel's Playlists:

  1. Mharthy's Channel
  2. mharthy’s channel
  3. mharthy’s
  4. mharthy
  5. channel
  6. mathematics
Рекомендации по теме
Prove (dy/dx) = 0:09:17

Prove (dy/dx) = (d/dx)(uv) = u(dv/dx) + v(du/dx), if y = uv

Product rule proof 0:09:25

Product rule proof | Taking derivatives | Differential Calculus | Khan Academy

Product Rule of 0:10:17

Product Rule of Differentiation/Derivative (Proof) | d/dx (uv) = u dv/dx + v du/dx

Product Rule | 0:09:26

Product Rule | Differencial Calculus

Quotient Rule of 0:08:02

Quotient Rule of Differentiation/Derivative (Proof) | d/dx (u/v) = (v du/dx - u dv/dx)/v^2

Basics of Differentiation 0:14:59

Basics of Differentiation Part - 5 | d/dx(U.V)

Prove (dy/dx) = 0:07:29

Prove (dy/dx) = (d/dx)(u+v) = (du/dx)+(dv/dx), if y = u+v

Chain Rule Method 0:06:01

Chain Rule Method of Differentiation | Derivatives

Difference Between Partial 0:01:44

Difference Between Partial and Total Derivative

If u, v 0:10:01

If u, v and w are functions of x, then show that d/dx (u. v. w) = du/dx v. w + u .dv/dx . w + u . v

Derivatives : d/dx(u+v) 0:00:55

Derivatives : d/dx(u+v) and d/dx(u-v) #shorts #Maths #Differentiation

Differentiation Tricks || 0:00:17

Differentiation Tricks || u.v and u/v rules #derivatives #differentiation

d/dx (u/v) = 0:02:14

d/dx (u/v) =

Differentiation Rules | 0:18:08

Differentiation Rules | Power Rule, Product Rule, Quotient Rule, Chain Rule | Derivative Basic Rules

part165. prove that 0:12:14

part165. prove that d(u.v.w)/dx=du/dx.v.w+u.dv/dx.w+u.v.dw/dx. In three different ways

dy/dx for y= 0:00:25

dy/dx for y= u/v form 🔥🔥💯😱 #youtubeshorts #trendingshorts #ytshorts #maths #calculus

rule of differentiation 0:00:59

rule of differentiation d(u.v)/dx | #shorts | ALIG Institute | #mathstrick #comment

How to find 0:01:24

How to find the derivative of square root of x (steps)

d/dx (x^n) = 0:13:52

d/dx (x^n) = nx^(n-1) | Differentiation of x^n | Proof in detail

UV RULE ( 0:04:16

UV RULE ( PRODUCT RULE)

If u, v 0:10:50

If u, v & w are Functions of x then Prove that d(u•v•w)/dx = du/dx•v•w + u•dv/dx•w + u•v•dw/dx...

Class 12- Differentiation-IF 0:03:34

Class 12- Differentiation-IF y= { x + (x^2 + a^2)}^n, prove that dy\dx= ny/ (x^2+a^2)^1/2

Differentiation Product Rule 0:00:53

Differentiation Product Rule #Shorts

Cosplay by b.tech 0:00:15

Cosplay by b.tech final year at IIT Kharagpur