filmov
tv
Chain rule proof | Derivative rules | AP Calculus AB | Khan Academy
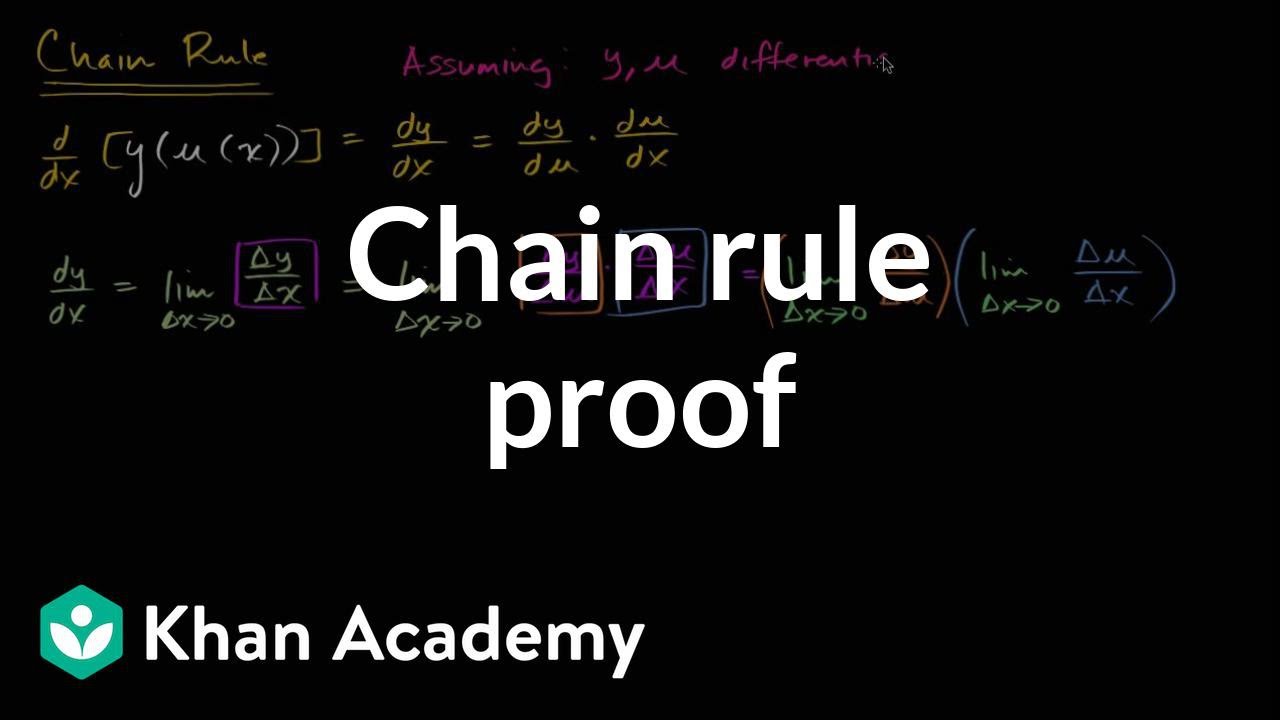
Показать описание
Here we use the formal properties of continuity and differentiability to see why the chain rule is true.
AP Calculus AB on Khan Academy: Bill Scott uses Khan Academy to teach AP Calculus at Phillips Academy in Andover, Massachusetts, and heÕs part of the teaching team that helped develop Khan AcademyÕs AP lessons. Phillips Academy was one of the first schools to teach AP nearly 60 years ago.
For free. For everyone. Forever. #YouCanLearnAnything
Chain rule proof | Derivative rules | AP Calculus AB | Khan Academy
Proof of the Chain Rule
Visualizing the chain rule and product rule | Chapter 4, Essence of calculus
Derivative Rules: Proof of Chain Rule
CHAIN RULE PROOF (DERIVATIVE OF COMPOSITE FUNCTION) #40
Chain Rule For Finding Derivatives
Chain rule | Derivative rules | AP Calculus AB | Khan Academy
Chain Rule Method of Differentiation | Derivatives
Vive Le Difference Quotient: Tabular, Graphical, and Analytic
Proof of a chain rule for partial derivatives
The Multi-Variable Chain Rule: Derivatives of Compositions
Leibniz's Derivative Notation (3 of 3: Introducing the chain rule)
Real Analysis | Derivative Rules
Visual Derivative Definition!
Derivatives of Composite Functions: The Chain Rule
Proof of the Multivariable Chain Rule
Chain Rule
chain rule for derivative but why do we need it, calculus 1 tutorial
Chain Rule: the Derivative of a Composition
The Chain Rule
Quotient rule from product & chain rules | Derivative rules | AP Calculus AB | Khan Academy
Proof of the Power Rule
Real Analysis 36 | Chain Rule
Multivariable chain rule
Комментарии
 0:05:39
0:05:39
 0:07:29
0:07:29
 0:15:56
0:15:56
 0:12:27
0:12:27
 0:07:17
0:07:17
 0:18:40
0:18:40
 0:05:07
0:05:07
 0:06:01
0:06:01
 1:02:10
1:02:10
 0:11:06
0:11:06
 0:10:47
0:10:47
 0:12:37
0:12:37
 0:14:24
0:14:24
 0:01:00
0:01:00
 0:12:29
0:12:29
 0:09:32
0:09:32
 0:05:27
0:05:27
 0:10:47
0:10:47
 0:05:28
0:05:28
 0:18:24
0:18:24
 0:05:15
0:05:15
 0:06:10
0:06:10
 0:06:17
0:06:17
 0:09:33
0:09:33