Understanding vector spaces in quantum mechanics

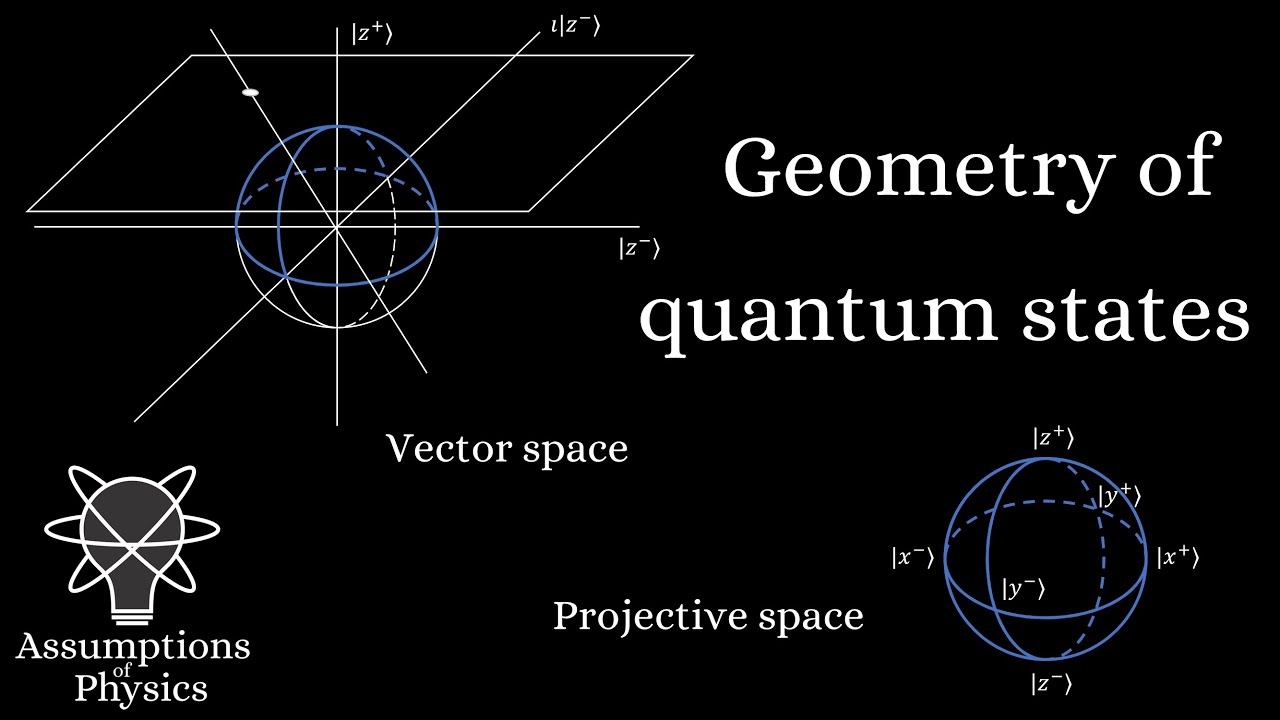
In this video we will explore the geometry of quantum states. While these are usually represented by vectors in Hilbert spaces, the true space is the projective space (the space of the "rays", one-dimensional sub-spaces). We will also see what is the difference between a complex vector space and a real vector space with double dimensions.

Intro (0:00)
States are "rays" (1:11)
Real projective line (3:08)
Real projective plane (8:44)
Real vs Complex vector spaces (13:20)
Complex projective line (18:40)
Bloch sphere (23:40)
Superposition (29:35)
Time evolution (32:06)
Physicality of superposition (38:16)
Conclusion (45:00)

[chrisscott]

The best explanation of the math in qm that I've ever found. Immediately cleared up YEARS of misconceptions.

[eternaldoorman]

This kind of janitorial cleaning-up is desperately needed! I sometimes get the feeling that physics is mainly about inter-generational trauma being passed from teachers to students, and it has epigenetic effects on the population of physicists a whole.

[nzuckman]

Thank you so much for making this!! Projective geometry seems to show up flippin *everywhere* in physics, especially the duality between circles and lines: I see it in kinematics, special relativity, electric and magnetic fields, atomic physics, and maybe even electrons and photons themselves! But this is one of the most beautiful and concrete examples I've come across. I can't shake the feeling that the pervasiveness of PG is a clue that will lead to some kind of deeply unifying principle.

[fawzibriedj]

I just did 2 years of "real" physics in university and it was very clear for me! It's beautifully put together.

[StefSubZero]

Guardando questo video mi è venuto in mente che qualche anno fa, al corso di meccanica quantistica qui ad UniTS, ci è stato fatto qualche accenno "geometrico" sulla struttura degli spazi di Hilbert e abbiamo citato anche gli spazi proiettivi, infatti è da lì che mi ricordo la rappresentazione di Bloch e la sfera di Bloch e che sulla superficie della sfera "vivono" proprio i Proiettori associati agli stati. Se non ricordo male mi erano state definite a quel punto le "miscele" come combinazioni convesse di autoproiettori associati ai rispettivi autovalori. Video molto interessante!

[GeoffryGifari]

some questions!
1. Does real projective line maps a semicircle into a circle?

2. Is there an analogous use of vector spaces in classical mechanics,
to make it easier to connect to quantum mechanics? just the (obviously) euclidean space?

3. On the inner product discussion 16:53, is one angle the phase angle of the complex plane "vector", and the other angle is the angle between two planes (because one complex number needs an entire plane. I imagine it like folding a piece of paper and looking at the angle between two sides)

4. In saying that the "complex ray" is the complex plane that passes through the origin, how do you define the "origin" when it comes to planes? It is unlike the (0, 0) point of the complex plane itself right?

5. Can we say the "the projective space is what matters" in quantum mechanics, because to link it to experimental data we always need an inner product/matrix element (the state itself is not sufficient)?

6. If superposition is not "fully" physical due to it not being the property of the projective space, what is the implication? possible violation of superposition/linearity that we can measure?

[oDonglero]

This is the most cogent explanation on this topic that I have seen and helped me sort the pieces I had into a whole picture. Awesome stuff! Thanks and keep going!

[FunkyDexter]

How does the born rule enter the picture? Is it something we can extract from the geometry, or is the geometry set by the acceptance of the validity of the born rule?

[GeoffryGifari]

for this material, do you happen to have a lecture note version?

[paolofreuli]

"Linearity is a property of the vector space, not of the projective space": this is very interesting and I was not aware. I am just wondering: if linearity is not a property of the projective space (and if I understood, it was sort of "pushed in" to make the math easier to deal with) and if the "physics is in the projective space" (where linearity is not required, based on your example which preserved the born rule results), how can we be sure\adfirm "quantum reversibility"? Sorry, probably a naive question, I just a curious one! thanks for this great stuff.

[sashadellorto]

I love your videos and explenations, I always find them well motivated and exposed. I was wondering if you have any textbook suggestions for a beginners introduction to quantum mechanics with all the completeness and clarity you offer on your channel.

Also - If I'm not indiscreet - I know you went from an engineering background to working with physics; I myself am studying engineering (al Polimi! come ho scoperto che ha fatto anche Lei) and also have a strong passion for physics. I hope to combine these two interests of mine in the future and was wondering if you had any suggestions for doing so (unfortunately not a lot of fundamental physics is taught at engineering!). Thank you!

[wheredowegofromhere]

I’ve never hit subscribe so fast after starting a video

[congtymienbac]

Your videos are great!
I have a small suggestion, because we use subtitles, white text, can you use your text in green or yellow? It will help us grasp ideas more clearly and easily.

[postbodzapism]

Will you talk about your modified gravity theory

[parthasarathikondapure]

I kid you not, I never seen anything like this. I am a graduate student in physics and this is the first time I've seen the geometry of quantum mechanics. Do you have any suggestions for books on this topic?

[christophertinklerart]

Thanks this is great and has helped tremendously

[angelamusiema]

Bellissima appossizione, e stupendo diverbio, professore degli azzurri. Un bel fiore narciso. "Io dico seo chi ne va di lusso ne va di galera", ma questa volta dico "chi na va di lusso e un bere da gioco! " Veramente, è ideale e stupenda spiegazione. Bye bye!

[Verlamian]

The main difference in quantum mechanics is the use of projective rather than non-projective (vector) spaces? No. If that were true then "Koopman-Von Neumann classical mechanics" - that well known reformulation of CM in the exact same mathematical formalism as QM - wouldn't exist! In reality the main difference is that in QM the natural incompatibility [noncommutativity] among certain "observables" is respected, and since classical (Kolmogorovian) probability can't accommodate noncommuting "random variables" or "observabes" it's _necessary_ to use the algebraic generalisation of probability ( arXiv:quant-ph/0601158 ).