filmov
tv
To Understand the Fourier Transform, Start From Quantum Mechanics

Показать описание
The Fourier transform has a million applications across all sorts of fields in science and math. But one of the very deepest arises in quantum mechanics, where it provides a map between two parallel descriptions of a quantum particle: one in terms of the position space wavefunction, and a dual description in terms of the momentum space wavefunction. Understanding this connection is also one of the best ways of learning what the Fourier transform really means.
We'll start by thinking about the quantum mechanics of a particle on a circle, which requires that the wavefunction be periodic. That lets us expand it in a Fourier series---a superposition of many sine and cosine functions, or equivalently complex exponential functions. We'll see that these individual Fourier waves are the eigenfunctions of the quantum momentum operator, and the corresponding eigenvalues are the numbers we can get when we go to measure the momentum of the particle. The coefficients of the Fourier series tell us the probabilities of which value we'll get.
Then, by taking the limit where the radius of this circular space goes to infinity, we'll return to the quantum mechanics of a particle on an infinite line. And what we'll discover is that the full-fledged Fourier transform emerges directly from the Fourier series in this limit, and that gives us a powerful intuition for understanding what the Fourier transform means. We'll look at an example that shows that when the position space wavefunction is a narrow spike, so that we have a good idea of where the particle is in space, the momentum space wavefunction will be spread out across a huge range. By knowing the position of the particle precisely, we don't have a clue what the momentum will be, and vice-versa! This is the Heisenberg uncertainty principle in action.
0:00 Introduction
2:56 The Fourier series
16:08 The Fourier transform
25:37 An example
About me:
Комментарии
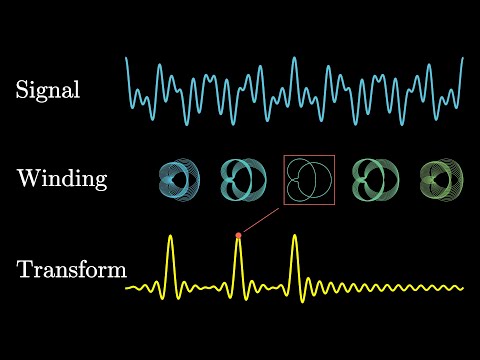 0:20:57
0:20:57
 0:31:37
0:31:37
 0:06:26
0:06:26
 0:14:48
0:14:48
 0:18:00
0:18:00
 0:19:07
0:19:07
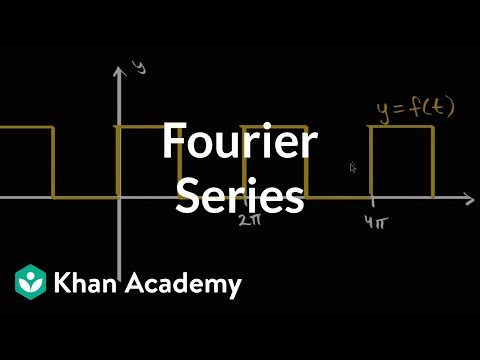 0:05:12
0:05:12
 0:19:20
0:19:20
 0:09:16
0:09:16
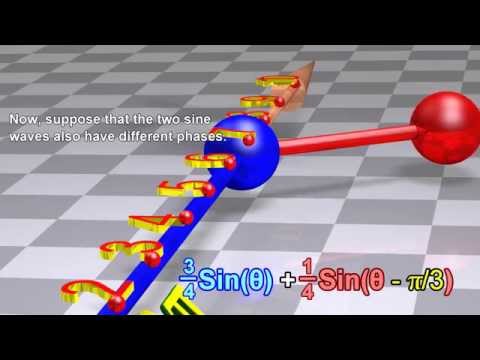 0:15:46
0:15:46
 0:01:47
0:01:47
 0:10:35
0:10:35
 0:03:20
0:03:20
 0:21:54
0:21:54
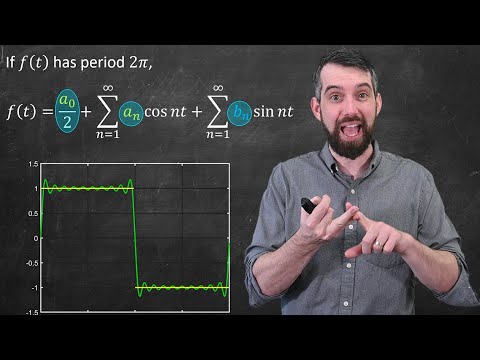 0:13:16
0:13:16
 0:06:22
0:06:22
 0:28:44
0:28:44
 0:10:44
0:10:44
 0:37:05
0:37:05
 0:03:01
0:03:01
 0:16:36
0:16:36
 0:13:03
0:13:03
 0:00:24
0:00:24
 0:24:47
0:24:47