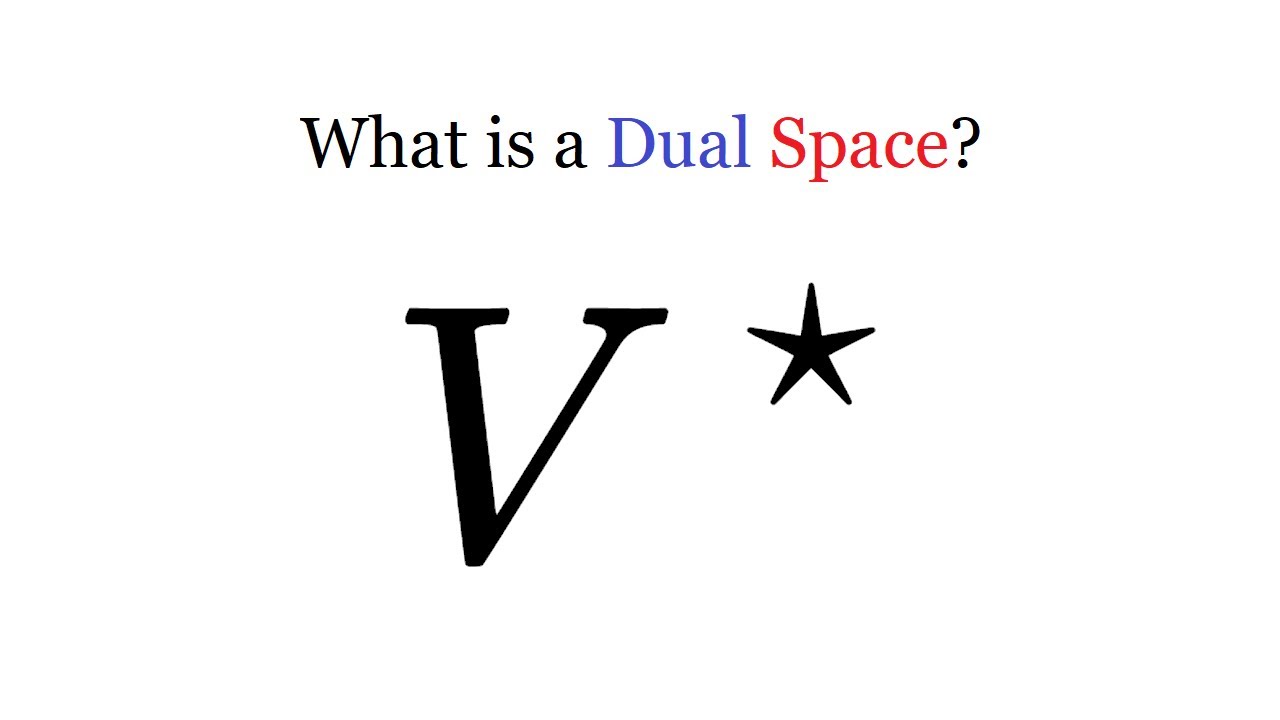
Dual Space

Dual spaces and linear functionals

In this video, I introduce the concept of a dual space, which is the analog of a "shadow world" version, but for vector spaces. I also give some examples of linear and non-linear functionals. This seems like an innocent topic, but it has a huge number of applications, which you'll see in subsequent videos (or check out the playlist below)

[OmnipotentEntity]

Hello comments readers! For clarity, it seems this series is already done, not a work in progress. There is a playlist link in the description to the other 14 (unlisted) videos. Hope you have a free afternoon.

[minymaker]

I want to thank you and the entire mathematics community. We are so lucky we have people like you teaching this for free

[WerIstWieJesus]

When I was much younger it was difficult for me to understand that the evaluation of polynomials at a single place was a linear functional. But when you evaluate at a single place all the monoms become constant and only the coefficients are variable. You get a linear functional. That was a very important step for me. Later I learned that this was true also with other base functions, not only monoms. And it is also true with defined integrals and linear functions on matrices to the real numbers . Linear vector spaces are very powerful and the heard of mathematics.

[apappas]

Finally, someone who can explain linear functionals. Thank you for providing so many examples to make it all very clear. I truly appreciate you!

[LuisFlores-mujc]

I came here because I am starting to get into Functional Analysis, and Operator Algebras. The video begins with an analogy about Legend of Zelda. Yes, I am in the correct place. I love your videos. They really bring the topics into perspective.

[mdforbes]

This answered my questions perfectly. I have been using O'Neill's "Elementary Differential Geometry" to teach myself, and he just referred to "dual space" in his definition of one-forms. After checking my linear algebra text (no dice) and prowling the internet's math forums for an hour of wasted time, I came here. Perfect explanation for what I needed without being overly complicated (unlike Stack Exchange). Thank you!

[jackhanson]

It is very pleasant to learn from someone who seems as excited to be teaching as I am to be listening.

[hiranabe]

Today, I found this video and WOW, i finally getting understanding of duality after 30 years!!!!

[David-ldus]

Thank you! I've been struggling to have an intuitive explanation of a dual space and you just gave it to me, with examples and everything. And the dimensionality and isomorphic explanation was great!

[TheLifeSpice]

Immediately won me over with the Zelda reference. Good stuff professor. Good stuff.

[juliankulshammer]

Regarding your last comment about the inequality of dimensions of an infinite dimensional vector space and its dual: The precise statement is that the dimension of the dual space of an infinite dimensional vector space is the cardinality of the field to the power of the dimension of V. This is called the Erdös-Kaplansky theorem. Since a field has at least two elements this is at least 2^(dim V), which is definitely bigger than dim V.

[LarryRiedel]

I'm looking forward to getting an intuition about transposes, and maybe a little about tensors and contravariant components.

[anthonysegers]

Thank you VERY much! You have opened the door to differential forms and Exterior Calculus for me.

[joshisushant]

love the level of enthusiasm, and the content is informative too.

[peppybocan]

Dual space is really interesting when it comes down to optimization problems (looking for min/max...)

[oitingcheung]

Those examples assist me a lot in understanding what a functional means. Thanks a lot!!

[jarodlee]

thanks very much. Best course for dual space ever!

[santiagosanz]

Is the 'scalar space' of a vector space reduced only to complex and real numbers? In 8:11 you said that a transformation wasn't a lineal fuctional because it didn't map to R nor C. But could it? Could and operation be defined so we could make elements from R2 scalars(I suppose it's possible since you could make them look like complex numbers)? But I'd like something more general.

I've had this question in the back of my mind since I started to learn about vector spaces. In some exercises we were told to see if a transformation was linear or not, so we had to see if f(x+y) = f(x) + f(y), and if f(kx) = kf(x), for all scalars k and all vectors x y.


But taking the first 'axiom'(?) of linearity, if we let x = y, then f(2x) = f(x+x) = f(x) + f(x) = 2f(x). This tells us that sometimes the second axiom doesn't have to be proven. Then I discovered it is valid also for negative and rational numbers, but it wasn't for irrational numbers. My guess is that it is valid only for non enumerable sets. Of course I'm only talking about numbers, but, as I said before, can we consider some scalar set that weren't numbers?



I don't know if I worded it right, I'm just very curious! Thanks!


By the way, I love your videos.

[ardalanardalan]

Thank you very much for this series. I'm always glad when I find out you have videos on the topic I am trying to learn. Keep it up.

[Osama-Bon-Jovi-]

I clicked on this this video when it had 314 views, must be a video by Dr πm.