filmov
tv
Linear combinations, span, and basis vectors | Chapter 2, Essence of linear algebra

Показать описание
The fundamental concepts of span, linear combinations, linear dependence, and bases.
An equally valuable form of support is to simply share some of the videos.
Future series like this are funded by the community, through Patreon, where supporters get early access as the series is being produced.
Thanks to these viewers for their contributions to translations
Arabic: @Cewkins, Hazem
Hebrew: Omer Tuchfeld
Spanish: Juan Carlos Largo
Vietnamese: @ngvutuan2811
------------------
3blue1brown is a channel about animating math, in all senses of the word animate. And you know the drill with YouTube, if you want to stay posted about new videos, subscribe, and click the bell to receive notifications (if you're into that).
Various social media stuffs:
An equally valuable form of support is to simply share some of the videos.
Future series like this are funded by the community, through Patreon, where supporters get early access as the series is being produced.
Thanks to these viewers for their contributions to translations
Arabic: @Cewkins, Hazem
Hebrew: Omer Tuchfeld
Spanish: Juan Carlos Largo
Vietnamese: @ngvutuan2811
------------------
3blue1brown is a channel about animating math, in all senses of the word animate. And you know the drill with YouTube, if you want to stay posted about new videos, subscribe, and click the bell to receive notifications (if you're into that).
Various social media stuffs:
Linear combinations, span, and basis vectors | Chapter 2, Essence of linear algebra
Linear combinations and span | Vectors and spaces | Linear Algebra | Khan Academy
Introducing Linear Combinations & Span
Subspaces and Span
Linear Algebra Example: Span Questions
Basis and Dimension
Span and linear independence example | Vectors and spaces | Linear Algebra | Khan Academy
Linear Combination.
ALADA-24Aug Lecture 07: Solutions to linear equations
1.2 Linear combinations, span, and basis vectors
❖ The Span of a Set of Vectors ❖
Linear Independence
The Vector Space of Polynomials: Span, Linear Independence, and Basis
Lecture 19: What is Linear Combination, Span and Basis set?
Span, basis and dimension | Lecture 18 | Matrix Algebra for Engineers
Linear Combinations Span Linear Independence
Linear Combinations and Span
Proof For Linear Combination Spanning Set
Linear Combinations & Span
Engineering Mathematics 12 | Linear Span, Basis, Dimensions | GATE All Branches
Oxford Linear Algebra: Basis, Spanning and Linear Independence
Independence, Basis, and Dimension
Linear Algebra - Lecture 7 - Linear Combinations and Vector Equations
Linear Algebra Example Problems - Linear Combination of Vectors #2
Комментарии
 0:09:59
0:09:59
 0:20:35
0:20:35
 0:09:34
0:09:34
 0:05:50
0:05:50
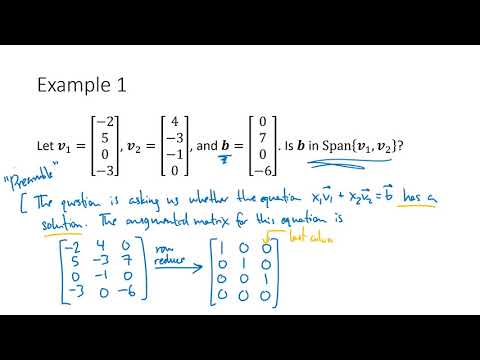 0:12:47
0:12:47
 0:10:06
0:10:06
 0:16:53
0:16:53
 0:00:50
0:00:50
 1:00:00
1:00:00
 0:08:20
0:08:20
 0:17:06
0:17:06
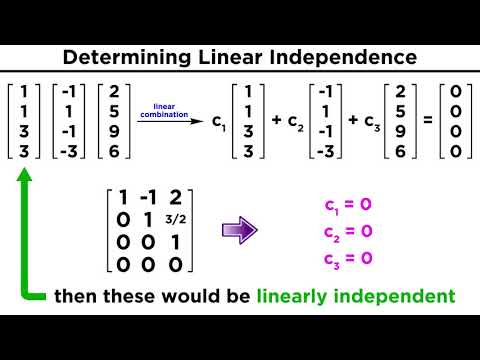 0:12:56
0:12:56
 0:12:50
0:12:50
 0:34:56
0:34:56
 0:10:34
0:10:34
 0:11:06
0:11:06
 0:17:01
0:17:01
 0:05:32
0:05:32
 0:02:31
0:02:31
 1:50:01
1:50:01
 0:41:44
0:41:44
 0:13:20
0:13:20
 0:08:10
0:08:10
 0:03:53
0:03:53