A continuous function on a compact set has compact image, Real Analysis II

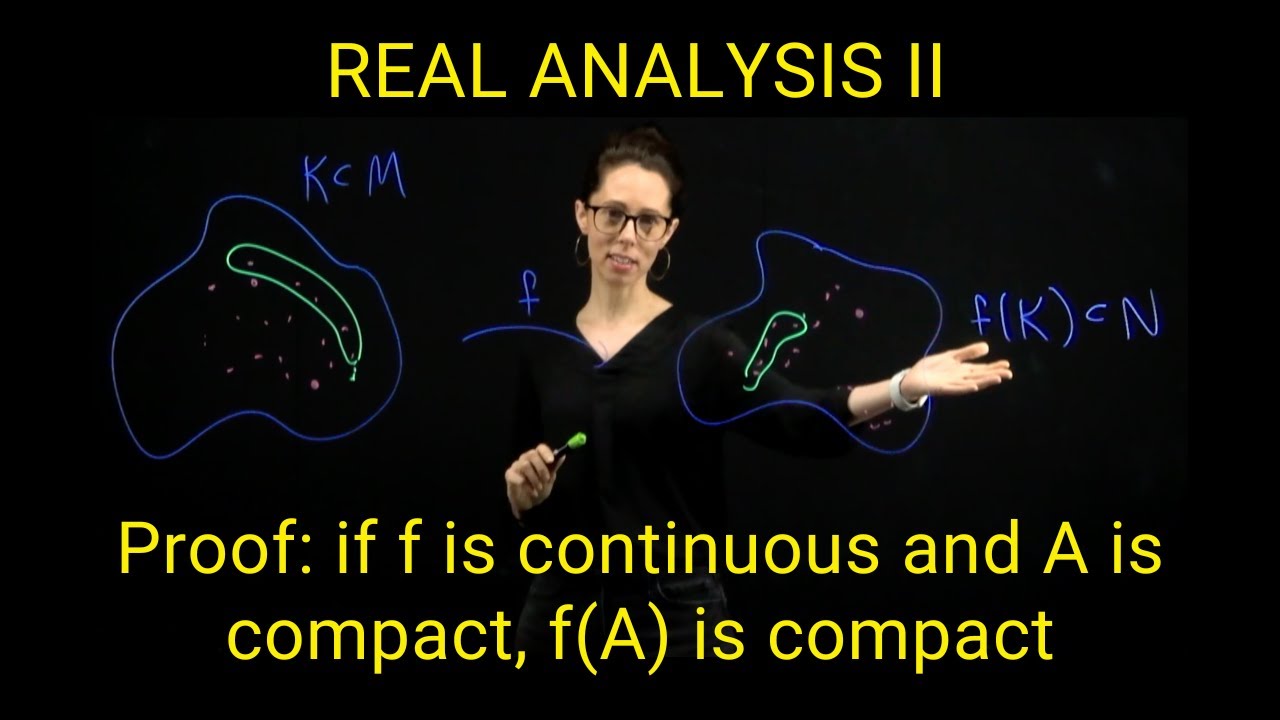
In this video, we prove that if a function f is continuous on a compact set K, then the image f(K) is also compact. We approach this proof in two ways: first by showing that f(K) is sequentially compact, and then by using the open cover definition of compactness.

For the sequential compactness proof, we take a sequence in f(K) and pull it back to K via the preimage, which yields a sequence in K. Since K is compact, we know this sequence has a convergent subsequence in K, and by continuity, its image in f(K) will also converge. This shows that f(K) is sequentially compact.

For the second proof using open covers, we start with an open cover of f(K), pull each open set back to create an open cover of K, and use the compactness of K to find a finite subcover. This finite subcover maps back to give a finite subcover of f(K), confirming the compactness of f(K).

These two proofs, while distinct, follow a similar logical structure and emphasize the core ideas of compactness, sequential compactness, and continuity.

#Mathematics #math #maths #Compactness #MetricSpaces #MathProof #RealAnalysis #STEM #CompactSets #Topology #advancedcalculus

[MikeMagTech]

That was very enjoyable and made perfect sense. Thank you.

[CrazyBunny-ckun]

try to upload a good thumbnail for youtube, than you video will see 1M viewer