filmov
tv
Real Analysis 27 | Continuity and Examples
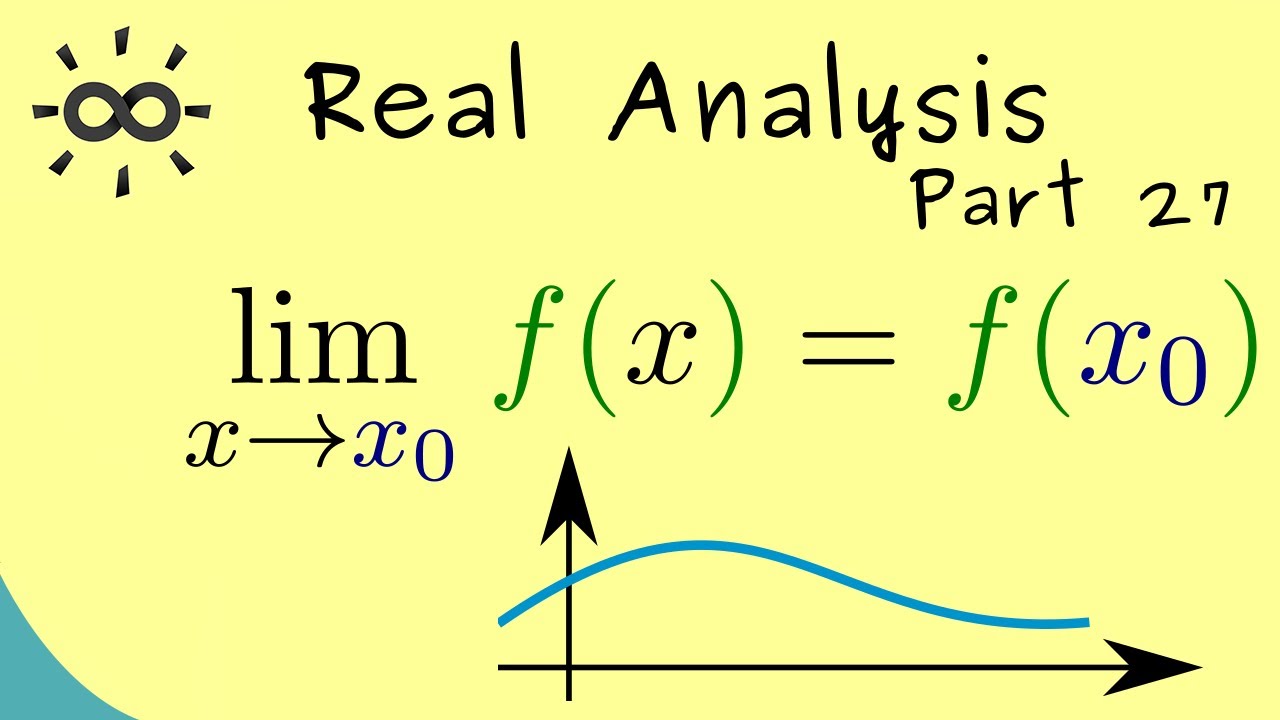
Показать описание
Please consider to support me if this video was helpful such that I can continue to produce them :)
🙏 Thanks to all supporters! They are mentioned in the credits of the video :)
This is my video series about Real Analysis. We talk about sequences, series, continuous functions, differentiable functions, and integral. I hope that it will help everyone who wants to learn about it.
x
00:00 Intro
00:18 Definition Continuity
01:22 Special case (holes in the domain of definition)
02:56 Extended definition of continuity
03:48 Examples
09:28 Credits
#RealAnalysis
#Mathematics
#Calculus
#LearnMath
#Integrals
#Derivatives
I hope that this helps students, pupils and others. Have fun!
(This explanation fits to lectures for students in their first and second year of study: Mathematics for physicists, Mathematics for the natural science, Mathematics for engineers and so on)
Real Analysis 27 | Continuity and Examples
Real Analysis 27 | Continuity and Examples [dark version]
This is the Epsilon Delta Definition of Continuity | Real Analysis
Real Analysis | Continuity
Definition of Continuity with Sequences! | Real Analysis
Application of IVP || Real Analysis || Continuity || Lecture 27 ||
Real Analysis | Intro to uniform continuity.
Introduction to Math Analysis (Lecture 27): Continuity on Abstract Metric Spaces
Real Analysis | Topological continuity
What is Uniform Continuity?
Real Analysis I (lec. 3.1.E) Continuity of a Function at a Point
Continuity of a function at a point
Continuity in Rn
Continuous function| continuity in an interval
Real Analysis | Continuity, connected sets, and the IVT.
Lipschitz Functions and Uniform Continuity
TRB POLYTECHNIC MATHS 2020 - Real analysis|| Continuity and compactness||
What is Continuity? According to a Mathematician.
Intro Analysis (Continuous and Uniform Continuity of Functions)
Real Analysis I (lec. 3.1.B) Continuity of a Function at a Point
Continuity 1 - Real Analysis
IIT JAM 2020 MATH Q.No.33 (Continuity of ONTO and one-one function)
real analysis problem and solution || problem on continuity ||
Intro Real Analysis, Lec 11: Continuity and the Intermediate Value Theorem (climbing Monk Story)
Комментарии
 0:09:59
0:09:59
 0:09:45
0:09:45
 0:12:14
0:12:14
 0:21:41
0:21:41
 0:05:53
0:05:53
 0:10:16
0:10:16
 0:14:17
0:14:17
 1:04:19
1:04:19
 0:09:53
0:09:53
 0:06:23
0:06:23
 0:17:35
0:17:35
 0:00:05
0:00:05
 0:12:46
0:12:46
 0:00:05
0:00:05
 0:14:23
0:14:23
 0:05:26
0:05:26
 0:04:27
0:04:27
 0:16:31
0:16:31
 0:11:00
0:11:00
 0:10:13
0:10:13
 0:20:12
0:20:12
 0:04:06
0:04:06
 0:05:02
0:05:02
 0:56:28
0:56:28