Proof: f(x) = x is Continuous using Epsilon Delta Definition | Real Analysis Exercises

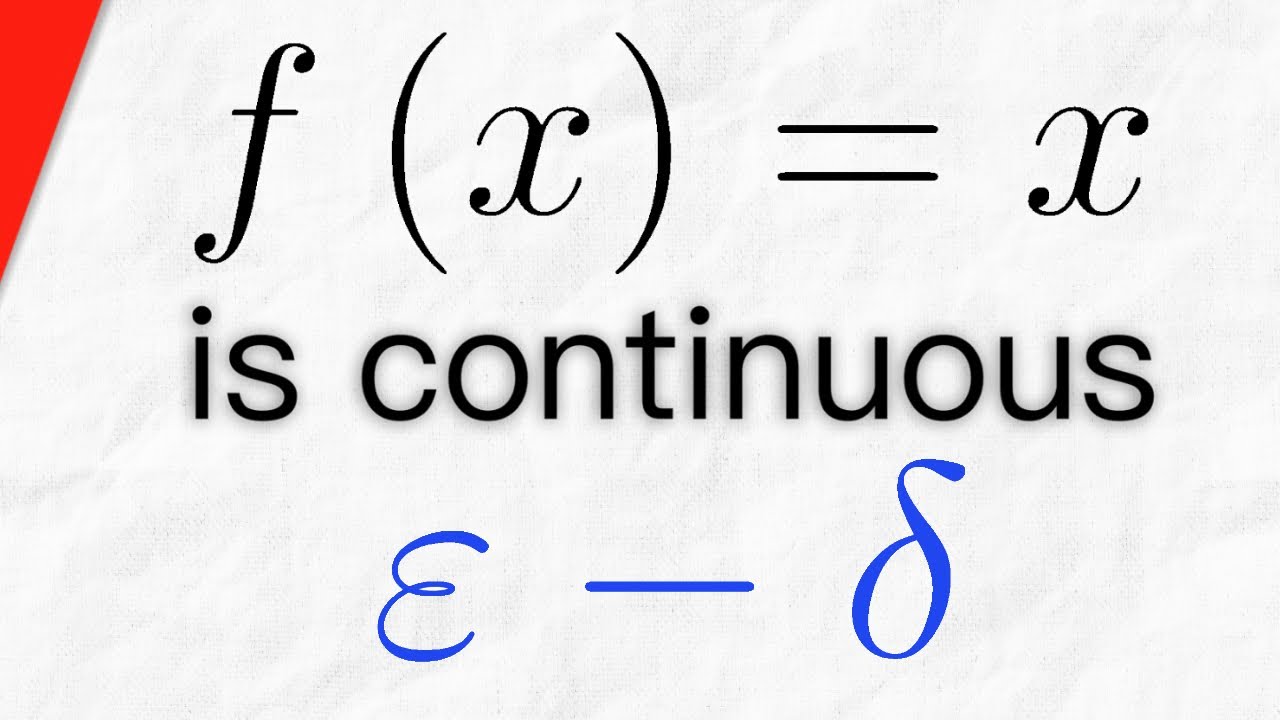
Показать описание

We prove that f(x)=x, the identity function, is continuous on its entire domain D, for any nonempty subset D of the real numbers. We complete this proof using the epsilon delta definition of continuity of a function at a point. To do this, we simply take an epsilon greater than 0 and an arbitrary point c from our domain, then go through the motions of finding a delta greater than 0 so that any x in D that is within delta of c has an image within epsilon of c's image. This of course is much more comprehensible in the video, where we can use mathematical notation. Let me know if there are more epsilon delta continuity proofs you want to see!

#Proofs #RealAnalysis

★DONATE★

Thanks to Robert Rennie, Barbara Sharrock, and Rolf Waefler for their generous support on Patreon!

Follow Wrath of Math on...

Рекомендации по теме

Комментарии

Thanks for watching! Several more continuity proofs are coming soon!

WrathofMath

"You'll be happy to know that they don't get any easier from here." Haha. Short and sweet. Appreciated the time you spent at the end pointing out the interesting implication of the proof for f(x) = x .

AManWithaZ

can you please prove f(x)=5x is continues for all real numbers using epsilon-delta

HMH

can you prove 2^x is continuous using epsilon delta definition please?

楚阑

Sir please make a video on sequential continuity.

kavitaarya

How can we infur x is c from x is not c?

princez

Proof: f(x) = x is Continuous using Epsilon Delta Definition | Real Analysis Exercises

Proof: f(x) = |x| is Continuous using Epsilon Delta Definition | Real Analysis Exercises

Proof: f(x) = x is Continuous using Epsilon Delta Definition | Real Analysis Exercises

Proof that f(x) = 1/x is Continuous on (0, infinity) using Delta-Epsilon

Proof that f(x) = x*|x| is Differentiable at x = 0

Proof formula Integral f(x)dx from 0 to a

Proof that f(x) = x^2 is Uniformly Continuous on (0, 1)

Proof f(x)=sin(x) is Continuous using Epsilon Delta Definition | Real Analysis Exercises

Proof that f(x) = 1/x is not Uniformly Continuous on (0,1)

VLOG | Aggie Hair Art x National Museum of African Art - Hair Show & Community Day

Prove f(x) = x^3 is a bijection. Set Theory. Abstract Algebra

Show that f(x) is continuous at x=4

Prove the function f:Z x Z → Z given by f(m,n) = 2m - n is Onto(Surjective)

Power Rule of Derivative Calculus - Proof f ' (X to the power n) = n.X to the power (n-1)

Injective Functions | Is f(x)=x^2 Injective? | A formal proof | Analysis | Math2Go

Prove that if f(x) = log(x/(x - 1)) then f(y + 1) + f(y) = log((y + 1)/(y - 1))

Determine if f(x) = x + 1/x is an Even or Odd Function

Derivatives - Proof why f'(a^x) = ln(a)a^x

How to Prove the Rational Function f(x) = 1/(x - 2) is Surjective(Onto) using the Definition

Proving the Function f(x) = sqrt(x + 2) is One to One(Injective) using the Definition

Proving a Function is a Linear Transformation F(x,y) = (2x + y, x - y)

Prove that the function `f(x)=x^n`is continuous at `x = n`, where n is a positive integer.

Prove that the identity function on real numbers given by `f(x) = x` is continuous at every real...

Prove f(x + a) - f(x - a) = 2a/(a^2 - x^2) if f(x) = 1/x

#07 prove that f(x)=1/x^2 is uniform contiouous on [a ,infinite) but not UC on (0, infinite)