filmov
tv
Differential Equation - 1st Order: Reducible to Separable Forms (2 of 7) Example 1: 2xyy'-y^2+ x^2=0

Показать описание
In this video I will solve 2xyy'-y^2+ x^2=0.
Next video in the 1st Order: Reducible to Separable Forms series can be seen at:
First Order Linear Differential Equations
Separable First Order Differential Equations - Basic Introduction
First order, Ordinary Differential Equations.
Differential equation introduction | First order differential equations | Khan Academy
First Order Linear Differential Equation & Integrating Factor (introduction & example)
How to Solve First Order Linear Differential Equations
The Method of Integrating Factors for Linear 1st Order ODEs **full example**
How To Solve First Order Homogeneous Differential Equation
Lec-02 | Solving Methods of Differential Equation | Differential Equation | Engineering Mathematics
Separable differential equations introduction | First order differential equations | Khan Academy
4 Types of ODE's: How to Identify and Solve Them
Solving First order linear differential equation
Linear Differential Equations & the Method of Integrating Factors
Differential equations, a tourist's guide | DE1
First Order differential Equations | Introduction on ODEs | Problems | Maths
Calculus 2 Lecture 8.1: Solving First Order Differential Equations By Separation of Variables
Introduction to Linear Differential Equations and Integrating Factors (Differential Equations 15)
Homogeneous Differential Equations
Modelling with First Order Differential Equations - Ordinary Differential Equations | Lecture 5
This is why you're learning differential equations
24 First-Order Differential Equations
Differential Equations Introduction | Differential Calculus Basics #differentialequation
🔵15 - Linear Differential Equations: Initial Value Problems (Solving Linear First Order ODE's)...
First Order Linear Differential Equations Part 1 (Live Stream)
Комментарии
 0:22:28
0:22:28
 0:10:42
0:10:42
 0:48:35
0:48:35
 0:07:49
0:07:49
 0:20:34
0:20:34
 0:10:53
0:10:53
 0:05:07
0:05:07
 0:08:33
0:08:33
 2:35:19
2:35:19
 0:09:05
0:09:05
 0:06:57
0:06:57
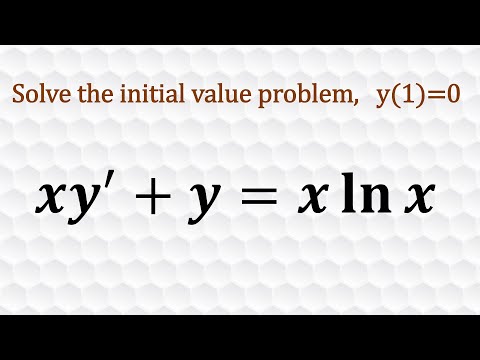 0:11:52
0:11:52
 0:11:36
0:11:36
 0:27:16
0:27:16
 0:07:12
0:07:12
 2:49:22
2:49:22
 1:07:16
1:07:16
 0:26:55
0:26:55
 0:18:22
0:18:22
 0:18:36
0:18:36
 4:56:20
4:56:20
 0:18:50
0:18:50
 0:21:16
0:21:16
 1:36:06
1:36:06