How to Solve First Order Linear Differential Equations

Linear equations - use of integrating factor

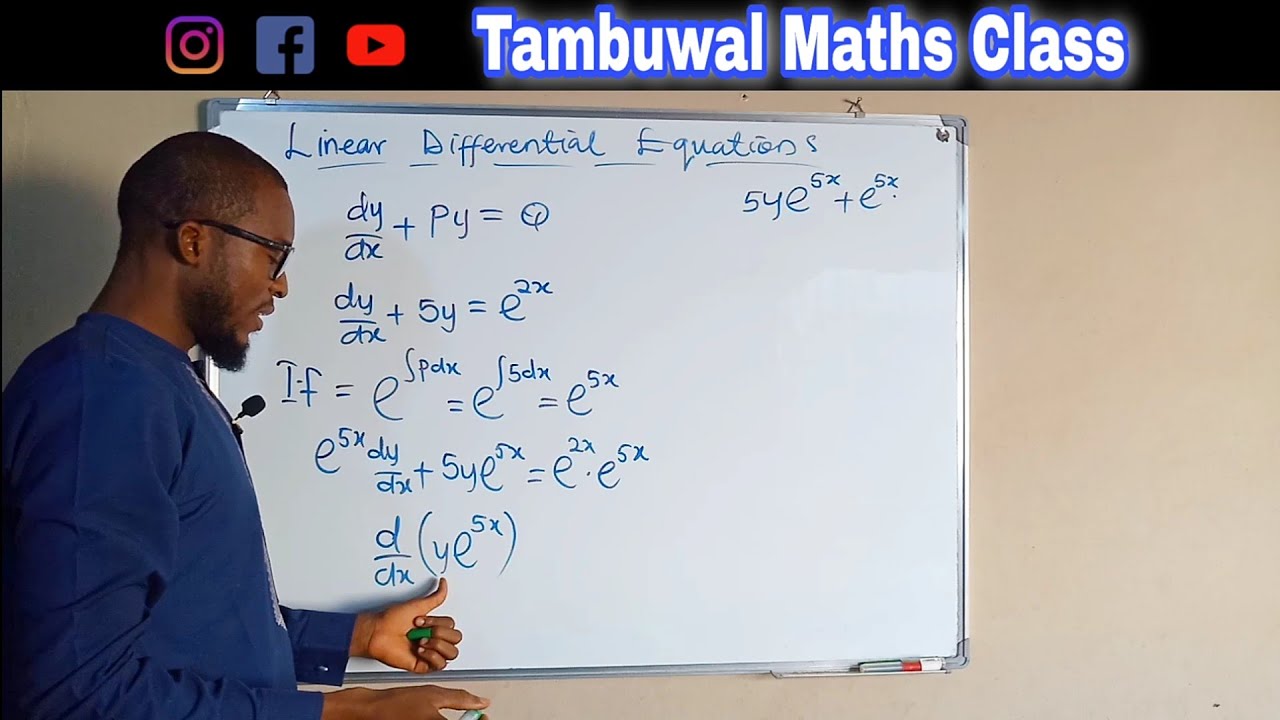
Consider the equation dy/dx + 5y = e²ˣ
This is clearly an equation of the first order , but different from those we have dealt with so far. In fact, none of our previous methods could be used to solve this one, so we have to find a further method of attack.

The above equation can be express in the form dy/dx + Py = Q , where P and Q are functions of x ( or constants ).
This equation is called a linear equation of the first order and to solve any such equation, we multiply both sides by an integrating factor which is always e^∫pdx
This converts the LHS into the derivative of the product of y and the integrating factor.

So to solve dy/dx + 5y = e²ˣ
where P = 5, therefore ∫pdx = 5x and the integrating factor is therefore e⁵ˣ
Note : In determining ∫pdx, we do not include a constant of integration. This omission is purely for convenience, for a constant of integration here would in practice give a constant factor on both sides of the equation, which would cubsequently cancel. This is one of the rare occasions when we do not write down the constant of integration.

So to solve dy/dx + 5y = e²ˣ we will multiply each term by the integrating factor
e⁵ˣ(dy/dx) + 5ye⁵ˣ=e⁷ˣ
The LHS can be express as d/dx(ye⁵ˣ)
Hence:
d/dx(ye⁵ˣ) = e⁷ˣ
By integrating both sides, we have
ye⁵ˣ= ⅐e⁷ˣ +c
and y = ⅐e²ˣ+ce⁻⁵ˣ

If you find this video interesting, kindly subscribe to my channel for more exciting Maths tutorials.

[zaibshaukat]

My exam is in a few hours and sir you helped me to understand this❤

[Maggio]

THANK YOU ! just started my differentials! will be watching you for the next 3 months ! love from Canada

[gerben]

Thank you sir! You are the first one to have me understand this! Thank you so much🙏🏻

[Solution]

Thank you sir.
I understood with ease and also got my integration by part straight after the video.

[bamideleadeniyi]

Tambuwal, this is brilliantly simplified

[ranmilowoisaiah]

How were you able to integrate 7x to get that 1/7 and also how did you get e^-5x at that e constant . I thought it was positive sign (5x) from the beginning

[asifimran]

Assalamu Alaikum sir. Your lectures are fantastic. Love from Bangladesh 🇧🇩

[marrahmohamedhassan]

Yes sir
Before taking the integral on both sides you need to multiply both sides by dX or transfer the dX to the right hand side by cross multiplication.
Thank you very much for your lectures.God bless you 🙏🙏

[sinthujanvinith]

Thank you sir,
Student from Sri Lanka.

[OsazuwaEro]

You are awesome sir.. Thank you for this

[sengwerbranton]

My first time but you teaching method's top notch 📌

[HILDAYIN-cw]

God bless you very much. But how did you appear on the screen just like that I guess you must have got some maths juju powers 😂😂😂

[amoszegrkaar]

With the linear DE I'm still struggling to understand e* where it's coming into the calculation

[farihamalik]

Jzakillah sir bht shukria bht Acha smjhya 👍👍👍🇵🇰🇵🇰

[kemumawhitney]

Watched 3 vedios of ODE.... perfectly understandable....I appreciate.... 💯 Subscription

[pratyushprayashjena]

Great sir i respect u so much for your content.

[I_am_tahyour]

Thank you very much sir
This is really understanding

[wanjalamudasiru]

Thank you very much sir, I like your discussion

[engmacharia]

YOU are great.finally i understand. thenks

[MATHEWCHERUIYOT-oidw]

Please help to solve this x+1dy/dx=y+x+x^2 given that x=1 and y=2