filmov
tv
The geometric interpretation of sin x = x - x³/3! + x⁵/5! -...

Показать описание
We first learnt sin x as a geometric object, so can we make geometric sense of the Taylor series of the sine function? For a long time, I thought it was just my dream, but actually, it is not!
This proof only uses very elementary methods, and depending on your definition of calculus, doesn't actually use calculus. The most we are using is a limiting process, and definitely no differentiation or integration here.
This proof is very beautiful - not only that it unveils the geometric meaning of each term in the series very beautifully, but also understandable by a normal high-school student with a little bit of patience. I am very surprised that it has not appeared on YouTube before, and even if it does exist on the internet, it is far too unpopular, and so I have to bring this up!
Obviously this is not my proof. See the sources below.
Sources:
Music:
Asher Fulero - Beseeched
Aakash Gandhi - White River, Heavenly, Lifting Dreams, Kiss the Sky
Video Chapters:
00:00 Introduction
00:50 Preliminaries
02:10 Main sketch
06:03 Details - Laying the ground work
09:42 The iteration process
11:11 Finding lengths of involutes
14:57 What? Combinatorics?
18:44 Final calculation
20:45 Fundraiser appeal
Other than commenting on the video, you are very welcome to fill in a Google form linked below, which helps me make better videos by catering for your math levels:
If you want to know more interesting Mathematics, stay tuned for the next video!
SUBSCRIBE and see you in the next video!
If you are wondering how I made all these videos, even though it is stylistically similar to 3Blue1Brown, I don't use his animation engine Manim, but I will probably reveal how I did it in a potential subscriber milestone, so do subscribe!
Social media:
For my contact email, check my About page on a PC.
See you next time!
This proof only uses very elementary methods, and depending on your definition of calculus, doesn't actually use calculus. The most we are using is a limiting process, and definitely no differentiation or integration here.
This proof is very beautiful - not only that it unveils the geometric meaning of each term in the series very beautifully, but also understandable by a normal high-school student with a little bit of patience. I am very surprised that it has not appeared on YouTube before, and even if it does exist on the internet, it is far too unpopular, and so I have to bring this up!
Obviously this is not my proof. See the sources below.
Sources:
Music:
Asher Fulero - Beseeched
Aakash Gandhi - White River, Heavenly, Lifting Dreams, Kiss the Sky
Video Chapters:
00:00 Introduction
00:50 Preliminaries
02:10 Main sketch
06:03 Details - Laying the ground work
09:42 The iteration process
11:11 Finding lengths of involutes
14:57 What? Combinatorics?
18:44 Final calculation
20:45 Fundraiser appeal
Other than commenting on the video, you are very welcome to fill in a Google form linked below, which helps me make better videos by catering for your math levels:
If you want to know more interesting Mathematics, stay tuned for the next video!
SUBSCRIBE and see you in the next video!
If you are wondering how I made all these videos, even though it is stylistically similar to 3Blue1Brown, I don't use his animation engine Manim, but I will probably reveal how I did it in a potential subscriber milestone, so do subscribe!
Social media:
For my contact email, check my About page on a PC.
See you next time!
Комментарии
 0:22:02
0:22:02
 0:05:20
0:05:20
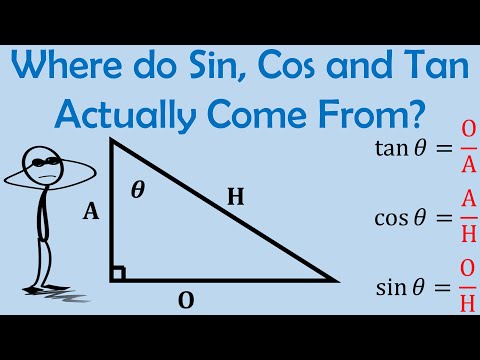 0:09:15
0:09:15
 0:02:19
0:02:19
 0:17:34
0:17:34
 0:00:31
0:00:31
 0:32:35
0:32:35
 0:17:05
0:17:05
 0:22:20
0:22:20
 1:13:17
1:13:17
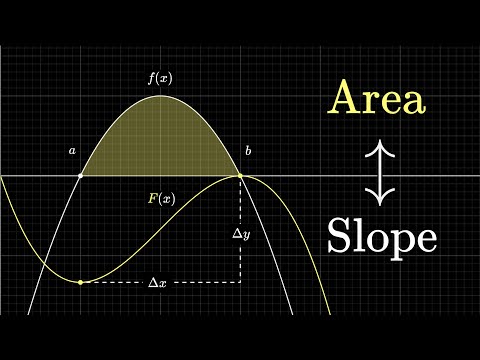 0:12:39
0:12:39
 0:14:45
0:14:45
 0:04:08
0:04:08
 0:20:46
0:20:46
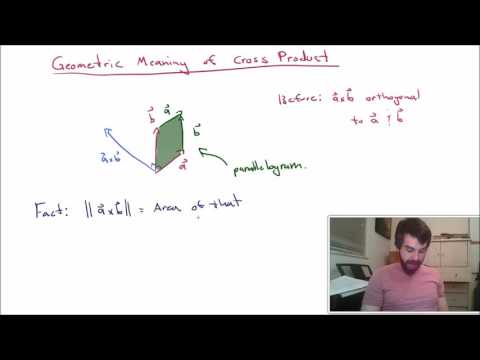 0:05:04
0:05:04
 0:13:11
0:13:11
 0:03:54
0:03:54
 0:06:14
0:06:14
 0:05:47
0:05:47
 0:00:50
0:00:50
 0:10:53
0:10:53
 0:00:58
0:00:58
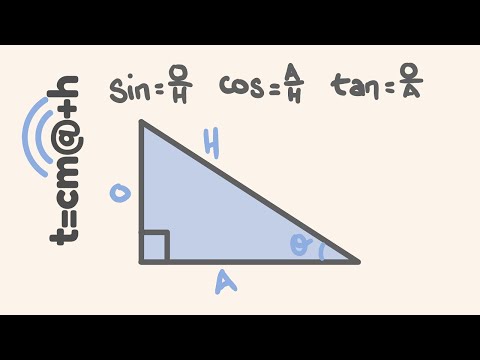 0:12:43
0:12:43
 0:00:54
0:00:54