filmov
tv
Proving the Inequality a(a+b)+b(b+1) ≥ -1/3

Показать описание
If you need to post a picture of your solution or idea:
#ChallengingMathProblems #ProvingInequalities
EXPLORE 😎:
PLAYLISTS 🎵 :
#ChallengingMathProblems #ProvingInequalities
EXPLORE 😎:
PLAYLISTS 🎵 :
Proving the Inequality a(a+b)+b(b+1) ≥ -1/3
Triangle Inequality for Real Numbers Proof
When mathematicians get bored (ep1)
Human Calculator Solves World’s Longest Math Problem #shorts
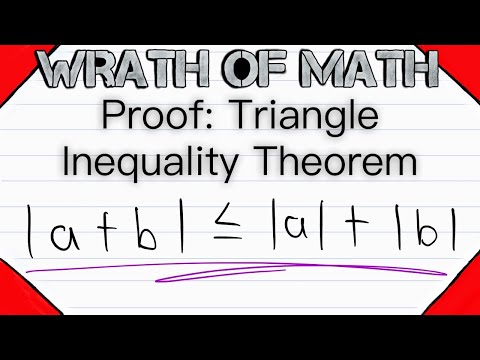
Proof: Triangle Inequality Theorem | Real Analysis
Prove | |a| - |b| | less than or equal to |a - b|
Reverse Triangle Inequality Absolute Value Proof
Proof: Reverse Triangle Inequality Theorem | Real Analysis
A Simple Way to Prove This Inequality | Olympiad Math
Can you prove this Inequality?
Proving an Inequality Using Inequalities
⚠️TRIANGLE INEQUALITY PROOF in 1 MINUTE, Real numbers, Calculus inequalities, #shorts
Inequalities for Math Olympiad: Proof Techniques with Transitivity and Expanding/Shrinking
Inequality Proof - Fraction Sum (1 of 3: Algebraic approach)
Trying transition video for the first time 💙😂 || #transformation #transition #shorts #viralvideo...
Can you prove this inequality? | Moscow Mathematical Olympiad Problem
IIT Bombay CSE 😍 #shorts #iit #iitbombay
Inequalities for Math Olympiad: Prove an Inequality
Proving a quick easy inequality #shorts
Prove this inequality! -International mathematical olympiad 2000
Inequality: a,b,c,x,y,z are real positive numbers and x+y+z=1 ;abc=1 Prove : a^2/x+b^2/y+c^2/z ≥ 9...
Prove an inequality of a, b, c, sides of a triangle, inequality of arithmetic and geometric means.
AM-GM Inequality via negative space
Proving a basic inequality - A different look.
Комментарии
 0:07:30
0:07:30
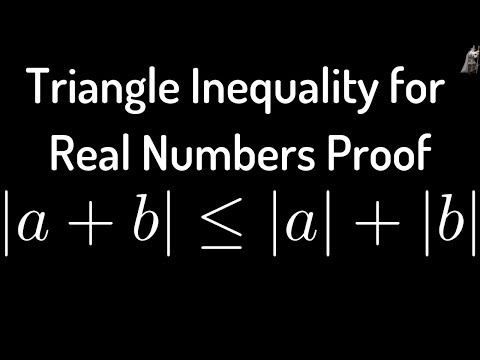 0:03:08
0:03:08
 0:00:37
0:00:37
 0:00:34
0:00:34
 0:05:30
0:05:30
 0:11:58
0:11:58
 0:03:37
0:03:37
 0:08:53
0:08:53
 0:06:20
0:06:20
 0:08:02
0:08:02
 0:14:50
0:14:50
 0:00:59
0:00:59
 0:14:29
0:14:29
 0:10:03
0:10:03
 0:00:15
0:00:15
 0:05:56
0:05:56
 0:00:11
0:00:11
 0:04:16
0:04:16
 0:00:32
0:00:32
 0:12:44
0:12:44
 0:03:01
0:03:01
 0:12:30
0:12:30
 0:01:00
0:01:00
 0:00:33
0:00:33