filmov
tv
Intro Complex Analysis, Lec 34, Series, Zeros, Isolated Singularities, Residues, Residue Theorem

Показать описание
Introduction to Complex Analysis Course, Lecture 34. (0:00) Exam information. (1:28) "Holomorphic function" means "analytic function"...we'll look at "meromorphic functions" as well. (2:26) A function that is analytic at a point has a Taylor series that converges to it in the largest open disk centered at the point over which the function is analytic. (5:32) Given a power series with a positive radius of convergence, it defines an analytic function whose Taylor series is the given power series. (7:30) Some people define analyticity in terms of power series. This also can be done to define real analytic functions, though that is not equivalent to being infinitely differentiable. (9:04) Laurent series representation in an annulus. (10:09) How to calculate the order of a zero of analytic function without calculating its Laurent series. Do a couple examples, including a polynomial and also f(z) = z*sin(z). (19:00) How to calculate the order of an isolated pole for a complex function which is analytic on a punctured disk near the singularity. (22:55) Facts about the behavior of complex functions near a removable singularity, and a pole of order m. (30:15) Picard's Theorem in complex analysis characterizes the wild behavior of a complex function near an essential singularity (and analytic in a punctured neighborhood of the essential singularity). (31:00) Definition of a meromorphic function. All rational functions are meromorphic. (32:16) Definition of the residue of a complex function at an isolated singularity. How to calculate the residue of a complex function at an isolated singularity. State what the theorem says when the the singularity is a pole of order 2 and give the idea of the proof in that case. (37:28) (Cauchy's) Residue theorem statement and give the idea of the proof in a picture when there are five isolated singularities. (42:29) Recall that the improper integral of 1/(1 + x^2) from minus infinity to infinity is equal to pi. This can be confirmed with the residue theorem (first use Mathematica to help recall what happens when we integrate 1/(1 + z^2) over a contour made up of a line segment along the real axis and a semicircle containing the the singularity at z = i. Then let the radius of the semicircle go to infinity).
AMAZON ASSOCIATE
As an Amazon Associate I earn from qualifying purchases.
Комментарии
 0:55:47
0:55:47
 0:56:18
0:56:18
 0:58:57
0:58:57
 0:53:29
0:53:29
 0:11:43
0:11:43
 0:57:43
0:57:43
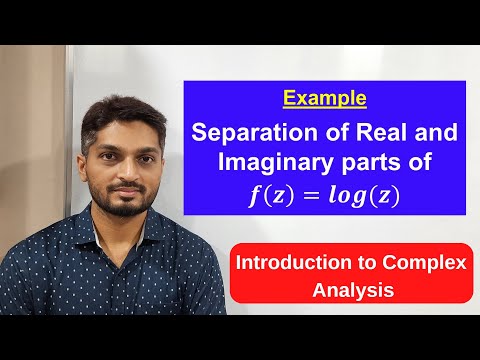 0:04:17
0:04:17
 0:55:30
0:55:30
 0:03:23
0:03:23
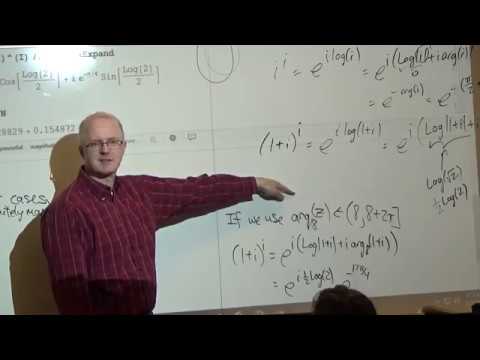 0:55:34
0:55:34
 0:55:15
0:55:15
 0:04:59
0:04:59
 0:58:28
0:58:28
 0:51:44
0:51:44
 0:11:29
0:11:29
 0:58:17
0:58:17
 0:12:40
0:12:40
 0:54:06
0:54:06
 0:19:12
0:19:12
 0:12:34
0:12:34
 0:12:40
0:12:40
 0:37:12
0:37:12
 0:09:46
0:09:46
 0:51:12
0:51:12