filmov
tv
Solution of homogeneous linear ordinary differential equation with constant coefficients
Показать описание
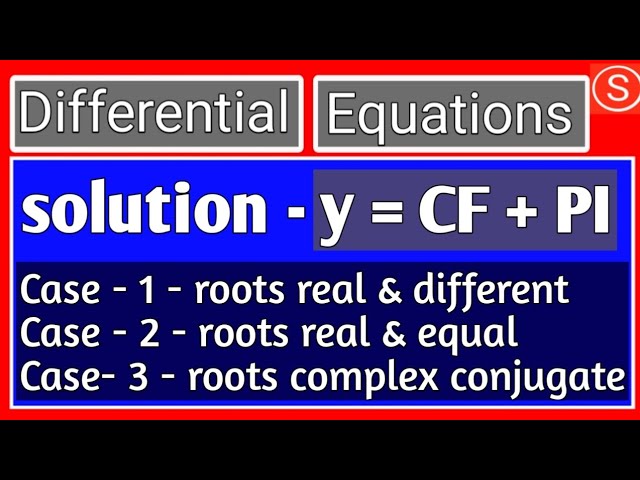
Solution of differential equation
A solution to a differential equation is a function y = f(x) that satisfies the differential equation when f and its derivatives are substituted into the equation.
A differential equation is an equation which contains one or more terms. It involves the derivative of one variable (dependent variable) with respect to the other variable (independent variable).
The order of a differential equation is the order of the highest order derivative involved in the differential equation. The degree of a differential equation is the exponent of the highest order derivative involved in the differential equation
For finding solution steps:
write auxiliary equation
take y outside then we will get quadratic equation
solve quadratic equation to get roots
06:30 Case -1 - roots are real and equal
07:29 case - 2 - roots are real and same
08:00 case -3 - roots are complex
complementary function
linear differential equations
homogeneous differential equation
complementary function and particular integral
complementary function and particular integral in telugu
complementary function and particular integral examples
complementary function of differential equations
complementary function for imaginary roots
complementary function and particular integral in tamil
complementary function of differential equation formula
complementary function of second order differential equations
A solution to a differential equation is a function y = f(x) that satisfies the differential equation when f and its derivatives are substituted into the equation.
A differential equation is an equation which contains one or more terms. It involves the derivative of one variable (dependent variable) with respect to the other variable (independent variable).
The order of a differential equation is the order of the highest order derivative involved in the differential equation. The degree of a differential equation is the exponent of the highest order derivative involved in the differential equation
For finding solution steps:
write auxiliary equation
take y outside then we will get quadratic equation
solve quadratic equation to get roots
06:30 Case -1 - roots are real and equal
07:29 case - 2 - roots are real and same
08:00 case -3 - roots are complex
complementary function
linear differential equations
homogeneous differential equation
complementary function and particular integral
complementary function and particular integral in telugu
complementary function and particular integral examples
complementary function of differential equations
complementary function for imaginary roots
complementary function and particular integral in tamil
complementary function of differential equation formula
complementary function of second order differential equations
Комментарии
 0:26:55
0:26:55
 0:06:41
0:06:41
 0:06:57
0:06:57
 0:08:28
0:08:28
 0:08:33
0:08:33
 0:07:59
0:07:59
 0:04:35
0:04:35
 0:14:30
0:14:30
 0:18:44
0:18:44
 0:03:02
0:03:02
 0:11:19
0:11:19
 0:11:03
0:11:03
 0:11:44
0:11:44
 0:25:17
0:25:17
 0:22:28
0:22:28
 0:24:11
0:24:11
 0:10:53
0:10:53
 0:12:42
0:12:42
 0:09:25
0:09:25
 0:12:44
0:12:44
 0:15:18
0:15:18
 0:02:13
0:02:13
 0:48:35
0:48:35
 0:01:47
0:01:47