How to Solve Constant Coefficient Homogeneous Differential Equations

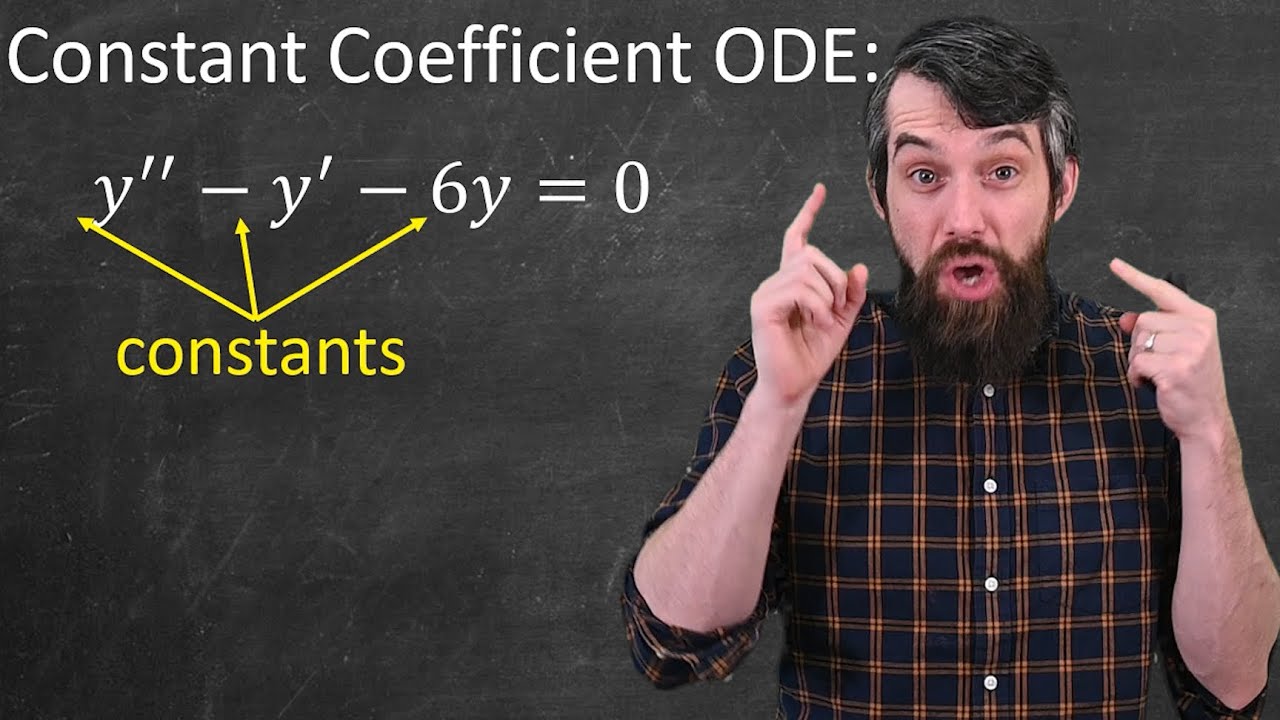
One class of second order ODEs is particularly nice: constant coefficient homogeneous ones. That is, it is linear in the dependent variable and its coefficients, and the right hand side is just zero. For this class, we make a guess or ansatz that the solution is an exponential. Plug that it and you get something called the characteristic equation or the auxiliary equation. Solve that via the quadratic formula and the roots tell you the types of exponentials. It turns out that there are really three cases that can arise, and I'll break those three cases down in the next video. For now we do a full initial value problem (IVP) from start to finish to introduce the methodology

OTHER COURSE PLAYLISTS:

OTHER PLAYLISTS:
► Learning Math Series
►Cool Math Series:

BECOME A MEMBER:

MATH BOOKS & MERCH I LOVE:

SOCIALS:

[punikachi]

Reading my math textbook I became able to solve second order ODE questions, but I didn't understand or have any intuition why the result and characteristic equation arises. The textbook literally just gave me the general solution form and said this is how it is. Thank you Dr. Trefor for actually helping me understand WHY this happens.

[selfstudypk]

Dr. Trefor Bazett i want to tell you that i love your teaching method and i always wait for your videos. thanks alot sir.

[isakwatz]

Took a course on differential equations recently, never understood why the characteristic equation works but your explanation is crystal clear! Thank you very much!

[branndn_]

For the first time, thanks for saving my upcoming engineering mathematics finals next week

[michaelhall]

I swear when I'm in a lecture this stuff sounds so complicated but watching this makes it seem so easy!

[angelmendez-rivera]

You can actually solve the equation y''(x) – y'(x) – 6·y(x) = 0 without having to guess the form of the solutions. In the comments section to a previous video, I discussed a principle, by which you can think of differential equations as being analogous to equations in linear algebra, where y is the unknown vector, and D^2 – D – 6·I is a linear operator, a "matrix" of some sort, which, when applied to y, outputs the function y'' – y' – 6·y. This is motivated by the idea that the derivative operator D is a linear operator, so you can rewrite y'(x) as D[y(x)], and y''(x) as (D^2)[y(x)]. If you also define I as the identity operator, so that I[y(x)] = y(x) is true for any y, then as such, y''(x) – y'(x) – 6·y(x) = 0 is equivalent to (D^2)[y(x)] – D[y(x)] – 6·I[y(x)] = 0. Furthermore, if you think of the distributivity property, you can rewrite this as (D^2 – D – 6·I)[y(x)] = 0. You can think of D^2 – D – 6·I as a single linear operator L, as a single "matrix, " and write the equation as L[y(x)] = 0. Why is this useful? Because what this tells you is that solving the differential equation is just equivalent to finding the null space of L!

This is nice and all, but how does this actually help in solving the equation? All I have done so far is present a different way of thinking about the equation, not an intuitive solution method. But now that I have presented the idea on how to conceptually recast the equation as a problem about linear operators or "matrices, " I can actually present the solution method. For starts, notice that L = D^2 – D – 6·I is a second-order polynomial in D, noting that D^0 = I. What is something that you can typically do with second-order polynomials? You can write them as a product of two first-order polynomials. In general, s^2 – s – 6 = (s + 2)·(s – 3), so perhaps you may think that, similarly, D^2 – D – 6·I = (D + 2·I)·(D – 3·I). Can this be justified rigorously? Yes. Keeping in mind that the multiplication T·S of two linear operators T and S is defined as T{S[y(x)]}, which is also how matrix multiplication is defined, you can use the distributive property to prove the above equality, and the order of the factors is not relevant, because D commutes with I, as do all linear operators. What does this imply? It implies I can write (D^2 – D – 6·I)[y(x)] = 0 as [(D + 2·I)·(D – 3·I)][y(x)] = 0, which you can also rewrite as (D + 2·I){(D – 3·I)[y(x)]} = 0. This is the key to solving the equation. Why? Because now, the structure of the equation calls for a substitution, one which is definitely far more obvious than any other possible substitution you can make here, and that substitution would be (D – 3·I)[y(x)] = z(x), resulting in the equation (D + 2·I)[z(x)] = 0. This is masterful, because this turns a second-order problem into simply two first-order problems! In particular, we already familiar with solving any first-order linear equation. This is more clear once we actually rewrite the equations. For example, (D + 2·I)[z(x)] = 0 can be written as z'(x) + 2·z(x) = 0, and now, surely, this looks like a familiar equation that people who are watching this video should know how to solve. I actually provided additional insight into how solving first-order equations is related to linear algebra in my comments to a previous video, the same ones I mentioned in my first paragraph. Getting into that is obnoxious here, though. For now, all you need to know is how to solve first-order linear equations. z'(x) + 2·z(x) = 0 is a separable equation, and it simply has the solutions z(x) = A·exp(–2·x). Once you know this, you can back-substitute to obtain y(x), so (D – 3·I)[y(x)] = A·exp(–2·x). If you would like, you can definitely just rewrite this as y'(x) – 3·y(x) = A·exp(–2·x). Then, you would just solve this as usual: multiply by the integrating factor, which in this case, is exp(–3·x), to obtain exp(–3·x)·y'(x) – 3·exp(–3·x)·y(x) = A·exp(–5·x). exp(–3·x)·y'(x) – 3·exp(–3·x)·y(x) is the derivative of exp(–3·x)·y(x), so by antidifferentiating, you get exp(–3·x)·y(x) = B – A/5·exp(–5·x). Therefore, y(x) = B·exp(3·x) – A/5·exp(–2·x). Now simply let B = C1, –A/5 = C2, and you obtain the exact same result in the video.

Instead of simply guessing the solution is of the form exp(k·x), though, you can intuitively think of this substitution using the concept eigenvalues. The functions of the form A·exp(k·x) are the solutions to the eigenvalue equation D[y(x)] = k·y(x). Since f(D) = D^2 – D – 6·I is a polynomial in D, and from linear algebra, you would know that D[y(x)] = k·y(x) implies [f(D)][y(x)] = f(k)·y(x) for polynomials f, you may realize that, therefore, the equation [f(D)][y(x)] = f(k)·y(x) is solved by A·exp(k·x) as well. However, as the actual equation says [f(D)][y(x)] = 0, you need f(k) = 0, which is just the famous characteristic equation that you always get in these problems, and in this case, that is simply the equation k^2 – k – 6 = 0, which has solutions k = –2, k = 3, which tells you that A·exp(–2·x) solves the equation, based on the eigenvalue principle, and so does B·exp(3·x), and thus, so does their sum, which is the general linear combination.

[TheSuperNerf]

You just saved me a weeks worth of catching up in my math course. Thank you with all of my soul

[MrJerahmeel]

Dr Bazett i'm an engineering student from italy. I just want to thank you for creating helpful videos and making maths visually more interesting, your videos like the SIR models, renews my love for math. So please keep doing helpful videos as you keep doing interesting and more advance matters too. THANK YOU!

[georgesadler]

Professor Bazett, this is a solid and clear explanation of How to Solve Constant Coefficient Homogeneous Differential Equations.

[chloes]

such a blessing, you explain things in a more engaging tone than my professor

[devrimeskibina]

Thank you so much sir. Your way of teaching is so simple yet effective.

[luccabraun]

Such a great and easy to follow video way better than reading a textbook before an exam. Thank you!

[entelakerri]

I loved DE in Uni!!! They're so fun to work with! Thank you Dr.Bazett !

[muhammadabuzarjanjoa]

Each and every second of your video is full of knowledge.
Loved it!👍

[manrajmann]

Nice work as usual Dr., You are A W E S O M E ! ! !

[noahrubin]

OMG literally learned this yesterday! Perfect timing

[jaredlee]

Dr. Bazett you literally saved my ass!

[mishudhar]

Hello, always loved your video. Do not miss a single one. This is the real blessing of internet. I am thousands mile away from you and learning by your video like in your class room. I have a question and i asked it before also. Do you have any plan to make video on mathematical analysis?

[j.o.]

Very pretty me like. Question to self: how do I guess the solution? Using e^something is a good start, as it's constant. But from experience, the primary way is to just do many problems to get a large reference pool

[anujmishra]

finally you had made it public, thanks to you had tried to join your channel but my card didnt work . Can you use other payment method like UPI which is more prevalent in india for online transaction .