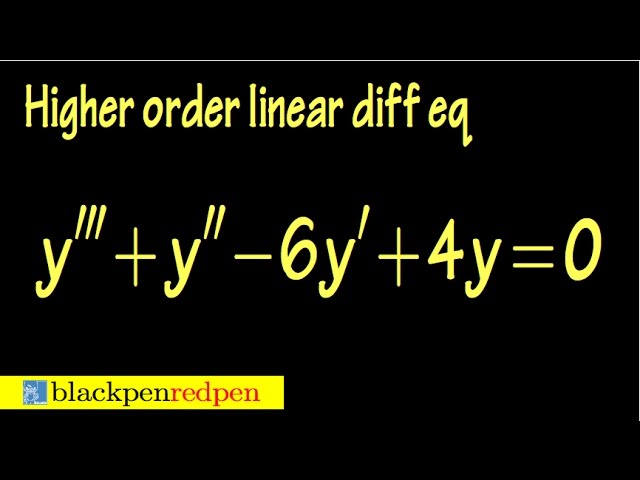
Higher order homogeneous linear differential equation, using auxiliary equation, sect 4.2#37

higher order homogeneous linear differential equation,
Solving higher-order differential equations using the auxiliary equation,
Solving higher-order differential equations using the characteristic equation,
higher order homogeneous linear differential equation, sect 4.2#37

blackpenredpen

[NWSCS]

The key to understanding how this works with this type of differential equation is understanding how the underlying algebra in finding the roots of the equation. The funny things is is that the calculus is not really all that difficult. It is the work you do with the algebra that can make it a rather long and tedious process. Nicely done my friend!

[garthenar]

"Keep your patience and everything will work out nicely" that is the best advice I've ever heard for math.

[matsigh]

I honeslty do not understand how my professor makes this so hard. Thank you so much.

[MediocreChannel]

I just noticed why his channel name is blackpenredpen, never looked at his hands

[stephenfulmer]

I just realized you switch expo markers with them both in your hand. What a awesome teacher. Thank you for all of your videos, I am currently a 4.0 student for Chemical engineering with a biology minor for pharmacy. I now work for my colleges tutoring department for calculus 1-3. I am about to start for DE. Thank you for what my professors couldn’t give me, I really appreciate it!!

[F]

Thank youuu. You're a great guy. You make me feel like you're just a friend explaining it to me, but your explaining is very clear and helpful!

[Anuq]

6 mins of this video was better than 2 hours of my professor

[fikrirhim]

thank you for teaching the easiest way to do long division

[moe]

this teacher is saving my GPA today, pray for me friends I got 3.5 hours to my final on ODE's

[sotosmath]

There is another trick for factoring out polynomials of degree>2.If you take the sum of the coefficients of the polynomial and the contastant one and if it turns out to be zero(the sum of them) then one of the roots of the polynomial is definitely 1!!! In this example if you sum 1+1-6+4=0 so the number 1 is one root of the polynomial!

[meliodas-sama]

This is the smoothest way I have learned HOODEs. Thank you!

[BukhalovAV]

Do you know about D/4? When coefficient B in Ax^2 + Bx +C is even, you can yous simplier formula:
D/4 = (B/2)^2 - AC
x = B/2 ± sqrt(D/4)

This way of solving square equations is better, when coefficients are big.

[jacobtjalkens]

This is the reason I haven't dropped out. If only he could teach all my classes.

[jahansaid]

Hey, I just want to thank you, and you are awesome :) I am going to get an A++, you only solve a couple question, but the good things is your example pretty much cover all the parameters :) Thank you very much !!

[ガアラ-hh]

Foun another way to do this problem sir in this equation it’s obvious that it must be something like e^ax so we can write equation as => e^x(a^3 +a^2 -6a +4) = 0

=> 0 = a^3 + a^2 -6a + 4

Trivial solution a = 1 now do polynomial division then you get all the solution you got

[Theterry]

alright so was I supposed to figure this out on my own for my webwork or...?

[longsteinpufferbatch]

I'm in 12th grade and it's nice to see that higher stuff like this is fairly easy

[mankienkueth]

This is very mature lecturer and he changed my life style in mathematics for all.

[obinnanwakwue]

You actually can factor the whole left side of the equation, just not by grouping: Use the rational root theorem where a_0 = 4 and a_n = 1. The factors of a_0 are 1, 2, and 4 and the factor of a_n is just 1. So you check for the following rational numbers: +/- (1, 2, 4)/1.

1/1 is a root, so (r - 1) can be factored. By applying polynomial long division, you can get (r^2 + 2r + 4). So the equation factors into (r - 1)(r^2 + 2r + 4) = 0. Then solving the equation is easier.

[eggshells]

great video! helped solving alot of constant coefficient problems