filmov
tv
why does 1+1/2+1/3+... = infinity?

Показать описание
Use "WELCOME10" for 10% off
---------------------------------------------------------
If you find this channel helpful and want to support it, then you can
join the channel membership and have your name in the video descriptions:
buy a math shirt or a hoodie (10% off with the code "WELCOME10"):
why does 1+1/2+1/3+... = infinity?
ASTOUNDING: 1 + 2 + 3 + 4 + 5 + ... = -1/12
This Result Keeps Me Up At Night
The 360-Page Proof That 1+1=2
Why is pi here? And why is it squared? A geometric answer to the Basel problem
Numberphile v. Math: the truth about 1+2+3+...=-1/12
I'm Settling This Math Debate Forever (.99 repeating = 1)
1 ^ ∞, It's Not What You Think
Proof that 1+1 = 2 【Fundamentals of Mathematics】
Every Student Should See This
1 + 1 = 3 Proof | Breaking the rules of mathematics
When mathematicians get bored (ep1)
If I did this in 1734 I'd be World Famous
Large Numbers
5 simple unsolvable equations
The Simplest Math Problem No One Can Solve - Collatz Conjecture
A visual infinite sum like you’ve never seen!
Absolute infinity Minus One?!?
Infinite Series - Numberphile
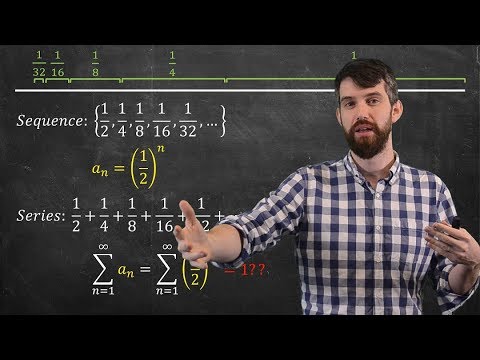
Intro to Series: What is 1/2+1/4+1/8+1/16+...?
The Alternate Math Universe Where Infinity is Tiny
EVERY RUBIK'S CUBE FROM 1x1 TO 19x19
How REAL Men Integrate Functions
the most famous Ramanujan sum 1+2+3+...=-1/12
Комментарии
 0:07:46
0:07:46
 0:07:50
0:07:50
 0:08:53
0:08:53
 0:06:03
0:06:03
 0:17:08
0:17:08
 0:41:44
0:41:44
 0:04:11
0:04:11
 0:04:28
0:04:28
 0:12:16
0:12:16
 0:00:58
0:00:58
 0:08:25
0:08:25
 0:00:37
0:00:37
 0:03:57
0:03:57
 0:00:58
0:00:58
 0:00:50
0:00:50
 0:22:09
0:22:09
 0:00:57
0:00:57
 0:00:21
0:00:21
 0:09:31
0:09:31
 0:05:38
0:05:38
 0:33:06
0:33:06
 0:00:39
0:00:39
 0:00:35
0:00:35
 0:11:40
0:11:40