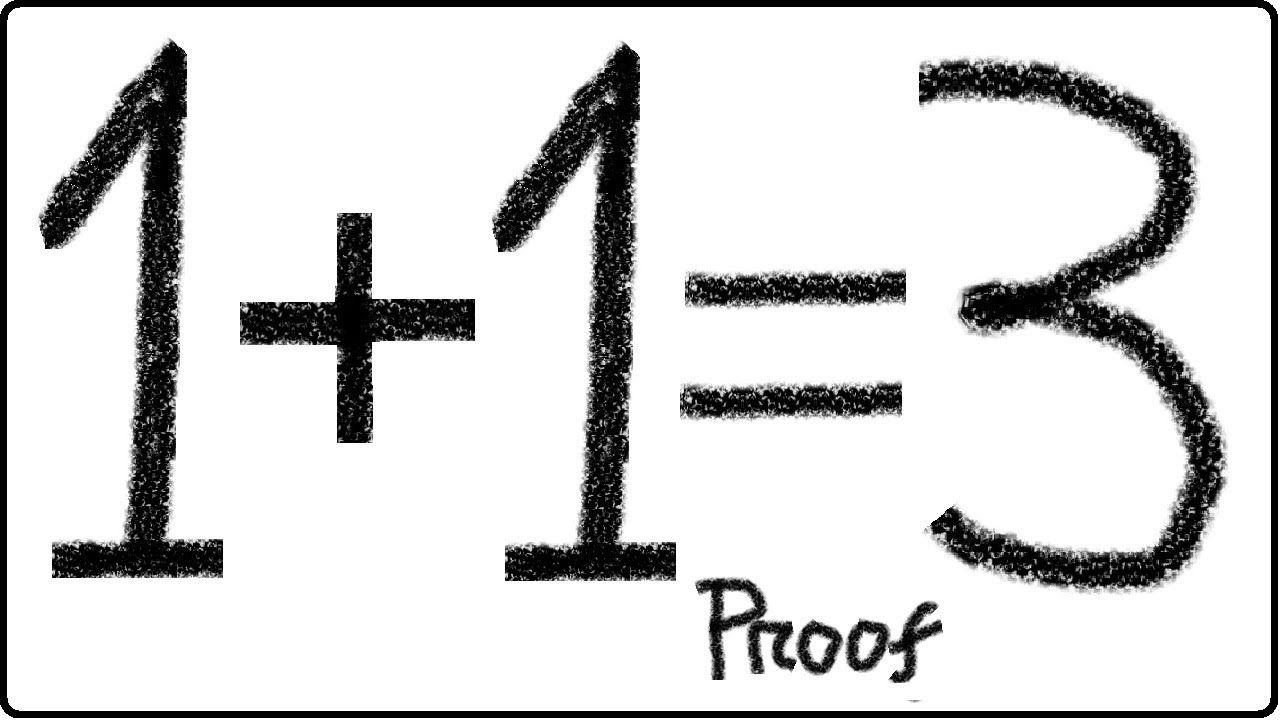
1 + 1 = 3 Proof | Breaking the rules of mathematics

1 + 1 = 3 Proof | Breaking the rules of mathematics.
One plus one equals three is possible only by breaking the rules of mathematics. 1+1=3 is not supported by mathematical logic. These strange results may come by the mistake during the calculations. This viral math tricks video is given here to show a message that a single mistake in mathematical calculations can lead to a destructive result.

The second part of this video containing how to prove 2+2 = 5. Two plus two equals five is an old mathematical illusion that also proves that a mistake in the calculation can make different results. 2+2=5 viral math problem may puzzle anyone. But if someone carefully watches the 2+2=5 video, there is a mistake in the calculation. The secret of 2+2=5 is hidden in its calculation. The ground rules of mathematics were not followed in 2+2=5 calculations.

The third part of this video shows how easy to multiply anything by 11. This fun of mathematics video is intended to show you a message that a simple mistake in mathematical calculations may lead to wrong results.
------------------------------------------------------------
------------------------------------------------------------
Watch other interesting videos on Matescium

------------------------------------------------------------
Thanks for watching it.

#1+1=3 #Mathtricks #Viralmath #1+1=3mathtrick #1+1=3How #1+1=3Proof

[ayushman]

3:04 We can't cancel the powers. Cancelling the powers means taking square root, and we always have to take modulus after taking square root

[lokeshpatel]

Leaving aside the square root step, I like how he uses 1+1 = 2 to get 1+1=3 😂

[parthgijare]

For those who are still wondering (1+1=3) why this happened is because In the step where he cancelled the squares on both the sides he did not use the concept of modulus. While taking square root on both sides the value obtained will be inside a mod sign.

Ex: we have [√(x)²] will not be equal to x but will be equal to | x |

[durgaprasadsimhadri]

In the 1st proof i.e 1+1=3, you should not cancel out the square of a negative number on one side and square of a positive number in another side.3:04
In the 2nd proof i.e 2+2=5, you should not cancel out the expression whose sum adds up to zero(0) in any equation. 6:08

[chimneone]

Math developers: Sorry for the inconvenience, we will patch this bug in the next update.

[farhanmoradi]

You shouldn't ignore the negative roots. You should put x in √(x²) into a absolute-value and reach a meaningful equation.

[minkebaleen]

The issue arises at 3:01 because after taking the square root on both sides of the equation, the result needs to be an absolute value. Therefore, the correct step should be |4-5| = |6-5|, meaning 5-4 = 6-5.

[BrixyBrixhamite]

the step where the squares are removed is where you take the square root of both sides, but the square root of a value has two possible values (one positive, and one negative). when the signs are taken into account you resolve the issues.
In the second calculation your cancellation step involves dividing by the value within the brackets (4-3-1), however given 4-3-1 is zero you are dividing both side by zero. The problem is dividing any integer by zero gives the result of infinity (so the correct result would be infinity = infinity).

[ankitkain]

He himself proved, maths rules are universal, hence can't be

[geeksahid]

In second calculation.

4(4-3-1)=5(4-3-1)
We can not cancel (4-3-1) from both sides, becoz the value of the term is zero and "0÷0" *is not defined* not "1" .

For better understanding

0=0
3×0=4×0

If we zero from both sides
Then
3=4
So thats why we cant say 0÷0 is 1
Its just not defined.

[georgesmith]

In the first case, 1+1 = 3, you reached the following equation:
There you removed the parenthesis power from both sides. while on the right side you can write (4-5) or (5-4) which means 4-5=-1 or 5-4=1 and on the left side you can write 5-6=-1 or Write 6-5=1. In other words, the square root of both sides has two answers: 1 and -1.
You deliberately ignored the -1 from the left side and set it equal to 1 on the right.
This is where the path to the wrong conclusion begins.

[Ministries_of_obedience]

(a-b)^2 = (b-a)^2 whereas (a-b) is not equal to (b-a); so you proved in a wrong way.

[prodigy]

It doesn't. Your formula is broken because you have two different values for x. On arrow line 3 you have x=4 y=5 on left side, then x=6 y=5 on right hand. The 6 should be a "z" "a" etc. so in principle your formula becomes (x-y)2-2xy=(z-y)2-2zy. So here you are considering "1" as a variable value, so then of course it could be equal to 3. But this logic you have shown above does not break the rules of mathematics.

[adityapradhan]

3:12; he just canceled the squares so if we just solve what's inside the bracket we get (-1)^2 = (1)^2 which is true since the square of a negative number is positive but after canceling the squares he wrote -1 = 1 which is not true and if we take the square root of (-1)^2 we get 1 so the correct thing after 3:12 would be 1 = 1 which is true

[samuelblossey]

I have gud proof: 1 dad + 1 mom = 1 child = 3 people

[garryzhou]

when you remove the squares from both sides, you must make sure that each side is a positive root or each is negative.

[terminator]

You can't cancel the squares, it's just for our convenience. The importance of brackets is the base of mathematics. I did the same mistake, but in another equation in class 8th, then after wondering for hours, I found out that this is the wrong way.

[ranijain]

Legends are shock after seeing this calculation 😱
🤣🤣🤣🤣🤣🤣🤣🤣

[vincentsauve]

This is like an advanced Abbot and Costello skit😂

[ts.nathan]

When cancelling the square, you should use (+ or -) in the first sum.

In the seciond sum you cancel (4-3-1). Here you must understand that this cancellation means you are dividing both sides by (4-3-1). It means you are dividing both sides by zero. So the resul is "infinity =infinity", and not " 4=5".