filmov
tv
Probability of Dice

Показать описание
Learn about dice probability. How do you calculate the probability of rolling an 8 with a pair of dice?
You can calculate the probability of rolling a number by using the number of favorable events divided by the number of total events.
You can calculate the probability of rolling a number by using the number of favorable events divided by the number of total events.
Probability of Dice
Die rolling probability | Probability and combinatorics | Precalculus | Khan Academy
Probability with Dice and Cards
Fair Dice (Part 1) - Numberphile
Die rolling probability with independent events | Precalculus | Khan Academy
Probability of a Dice Roll | Statistics & Math Practice | JusticeTheTutor #shorts #math #maths
The unexpected logic behind rolling multiple dice and picking the highest.
Probability - How Can You Use Probability In A Dice 🎲 Game | Probability Of Rolling Dice | BYJU&apo...
PROBABILITY (A - Z) | Part 1 | Introduction & Types of Problems & Coins based Problems
Math Antics - Basic Probability
Predicting How Dice Will Roll Isn't So Hard
Roll A Pair Of Dice | Probability Math Problem
Probability of Consecutive Coin Flips
Probability When Rolling Two Dice Matrix Method
How to solve probability problems with dice
Probability Curves and the Psychology of Dice - How to Create a Game Experience with Dice
How to Determine the Probability of Rolling a Dice When the Number Is Greater Than 3 or Less Than 5
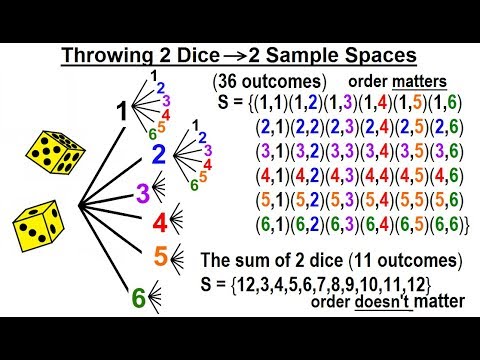
Statistics: Ch 4 Probability in Statistics (24 of 74) Throwing 2 Dice: 2 Sample Spaces
Dice Games - Probability - GCSE Mathematics
Two dice are rolled. What is the probability of getting a sum of 6?
Probability - Equally Likely Events | Throwing a die | Don't Memorise
Probability - P(11) When Two Dice are Rolled? | Don't Memorise
Probability: Cases of Dice | Part 1/3 | English | Class 10
Probability Of Rolling Dice | How To Calculate Dice Probabilities #Shorts #NowWeKnow
Комментарии
 0:02:44
0:02:44
 0:05:15
0:05:15
 0:04:01
0:04:01
 0:13:14
0:13:14
 0:02:28
0:02:28
 0:00:38
0:00:38
 0:27:29
0:27:29
 0:03:43
0:03:43
 1:02:37
1:02:37
 0:11:28
0:11:28
 0:03:48
0:03:48
 0:00:41
0:00:41
 0:00:25
0:00:25
 0:08:35
0:08:35
 0:04:37
0:04:37
 0:08:55
0:08:55
 0:01:14
0:01:14
 0:02:42
0:02:42
 0:10:31
0:10:31
 0:04:47
0:04:47
 0:03:23
0:03:23
 0:04:13
0:04:13
 0:03:11
0:03:11
 0:00:16
0:00:16